A satellite of mass m is in an elliptical orbit around the earth of mass M(M>>m) the speed of the satellite at its nearest point ot the earth (perigee) is √6GM5R where R= its closest distance to the earth it is desired to transfer this satellite into a circular orbit around the earth of radius equal its largest distance from the earth. Find the increase in its speed to be imparted at the apogee (farthest point on the elliptical orbit).Solution in Gujrati
The correct Answer is:√GMR[√23−√815]
Let r= distance of apogee
COAM: √6GM5RR=vr (i)
COME: −GMmR+12m(6GM5R)
=−GMmr+12mv2 ..(ii)
⇒r=3R2 and v=√8GM15R
Orbital speed at r
=√GMr=√23GMR
∴increase in speed
=√GMR[√23−√815]
Topper's Solved these Questions
- GRAVITATIONBOOK - ALLEN CHAPTER - GRAVITATIONEXERCISE - Exercise 5 A (Previous Year Questions)
26 Videos
- GRAVITATIONBOOK - ALLEN CHAPTER - GRAVITATIONEXERCISE - Exercise 5 B (Previous Year Questions)
9 Videos
- GRAVITATIONBOOK - ALLEN CHAPTER - GRAVITATIONEXERCISE - Exercise 4 A (Conceptual Subjective Exercise)
14 Videos
- GEOMETRICAL OPTICSBOOK - ALLEN CHAPTER - GEOMETRICAL OPTICSEXERCISE - subjective
14 Videos
- KINEMATICS-2DBOOK - ALLEN CHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)
47 Videos
Similar Questions
If suddenly the gravitational force of attraction between earth and satellite revolving around it becomes zero, then the satellite will
View SolutionA satellite S is moving in an elliptical orbit around the earth. The mass of the satellite is very small compared to the mass of the earth.
View Solution_E01_076_Q01.png)
In the given figure L=1 metre if total gravitational force on 4 kg mass is →F1 and on 2 kg mass is →F2 then find out the ratio of ∣∣∣→F1→F2∣∣∣
View SolutionA prism of refractive index n1 & another prism of reactive index n2 are stuck together without a gap as shown in the figure.The angle of the prisms are as shown. n1&n2depend onλ,the wavelength of light according to n1=1.20+10.8×104λ2&n2=1.45+1.80×104λ2 where λ is in nm.

(i)Calculate the wavelength λ0for which rays incident at any angle on the interfaceBCpass through without bending at that interface.
(ii) for light of wavelength λ0,find the angle of incidencei on faceACsuch that the deviation produced by the combination of prism is minimum.
View SolutionA particle is moving in xy-plane .At certain instant the components of its velocity and acceleration are as follows ux=3m/s,uy=4m/s,ax=2m/s2anday=1m/s2. The rate of change of speed at this moment is
View SolutionA satellite moves around the earth in a circular orbit with speed v . If m is the mass of the satellite, its total energy is
View SolutionA satellite of mass m is in an elliptical orbit around the earth. The speed of the satellite at its nearest position is (6GM)//(5r) where r is the perigee (nearest point) distance from the centre of the earth. It is desired to transfer the satellite to the circular orbit of radius equal to its apogee (farthest point) distance from the centre of the earth. The change in orbital speed required for this purpose is
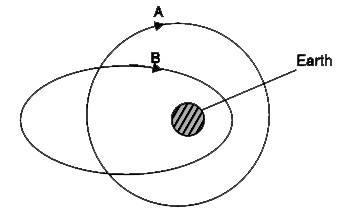
View SolutionSatellite A is following a circular path of radius a around the earth another satellite B follows an elliptical path around the earth. The two satellites have same mechanical energy and their orbits intersect. Find the speed of satellite B at the point where its path intersects with the circular orbit of A. Take mass of earth to be M.
View SolutionA satellite of mass m is orbiting around the earth at a height equal to twice the radius of the earth (R). Its potential energy is given by
View Solutionएक उपग्रह पृथ्वी के चारों ओर त्रिज्या r की कक्षा में चाल v से घूम रहा है | यदि उपग्रह का द्रव्यमान M है तो उसकी कुल ऊर्जा होगी :
View SolutionA satellite is orbiting around the Earth in a circular orbit with velocity v. If m is the mass of the satellite, its total energy is .........
View SolutionA satellite moves around the earth in a circular orbit with speed V. If 'm' is the mass of the satellite, its potential energy is
View SolutionA satellite moves around the earth in a circular orbit with speed V. If 'm' is the mass of the satellites, its potential energy is
View SolutionA satellite moves around the earth in a circular orbit with speed V. If 'm' is the mass of the satellites, its total energy is
View SolutionA satellite moves around the earth in a circular orbit with speed V. If 'm' is the mass of the satellite, its potential energy is
View SolutionA satellite moves around the earth in a circular orbit with speed V. If 'm' is the mass of the satellites, its potential energy is
View Solution
