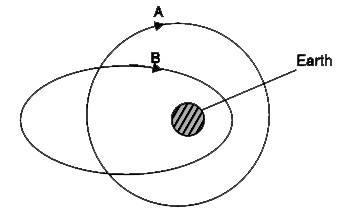
[Gujrati] Consider two satellites A and B of equal mass m, moving in t

If suddenly the gravitational force of attraction between earth and satellite revolving around it becomes zero, then the satellite will

View Solution

A satellite S is moving in an elliptical orbit around the earth. The mass of the satellite is very small compared to the mass of the earth.

View Solution

ocr_image
In the given figure $L = 1$ metre if total gravitational force on 4 kg mass is ${\to F}_{1}$ and on 2 kg mass is ${\to F}_{2}$ then find out the ratio of $∣ ∣ ∣ \frac{{\to F}_{1}}{{\to F}_{2}} ∣ ∣ ∣$

View Solution

A prism of refractive index $n_{1}$ & another prism of reactive index $n_{2}$ are stuck together without a gap as shown in the figure.The angle of the prisms are as shown. $n_{1} & n_{2}$ depend on $λ$ ,the wavelength of light according to $n_{1} = 1.20 + \frac{10.8 \times 10^{4}}{λ^{2}} & n_{2} = 1.45 + \frac{1.80 \times 10^{4}}{λ^{2}}$ where $λ$ is in nm.
ocr_image
(i)Calculate the wavelength $λ_{0}$ for which rays incident at any angle on the interface $B C$ pass through without bending at that interface.
(ii) for light of wavelength $λ_{0}$ ,find the angle of incidencei on face $A C$ such that the deviation produced by the combination of prism is minimum.

View Solution

A particle is moving in xy-plane .At certain instant the components of its velocity and acceleration are as follows $u_{x} = 3 m / s, u_{y} = 4 m / s, a_{x} = 2 m / s^{2} and a_{y} = 1 m / s^{2} .$ The rate of change of speed at this moment is

View Solution

Consider two satellites A and B of equal mass, moving in the same circular orbit of radius r around the earth but in the opposite sense and therefore a collision occurs. (a) Find the total mechanical energy E_(A) + E_(B) of the two satellite-plus-earth system before collision. (b) If the collision is completely inelastic, find the total mechanical energy immediately after collision. Describe the subsequent motion of the combined satellite.

View Solution

Consider two satellites A and B of equal mass m , moving in the same circular orbit about the earth, but in the opposite sense as shown in Fig. The orbital radius is r . The satellites undergo a collision which is perfectly inelastic. For this situation, mark out the correct statement(s). [Take mass of earth as M ]

View Solution

Satellite A is following a circular path of radius a around the earth another satellite B follows an elliptical path around the earth. The two satellites have same mechanical energy and their orbits intersect. Find the speed of satellite B at the point where its path intersects with the circular orbit of A. Take mass of earth to be M.
ocr_image

View Solution

Two satellite of same mass are launched in the same orbit of radius $r$ around the earth so as to rotate opposite to each other. If they collide inelastically and stick together as wreckage, the total energy of the system just after collision is

View Solution

Match the Column I with Column II
For a satellite in circular orbit
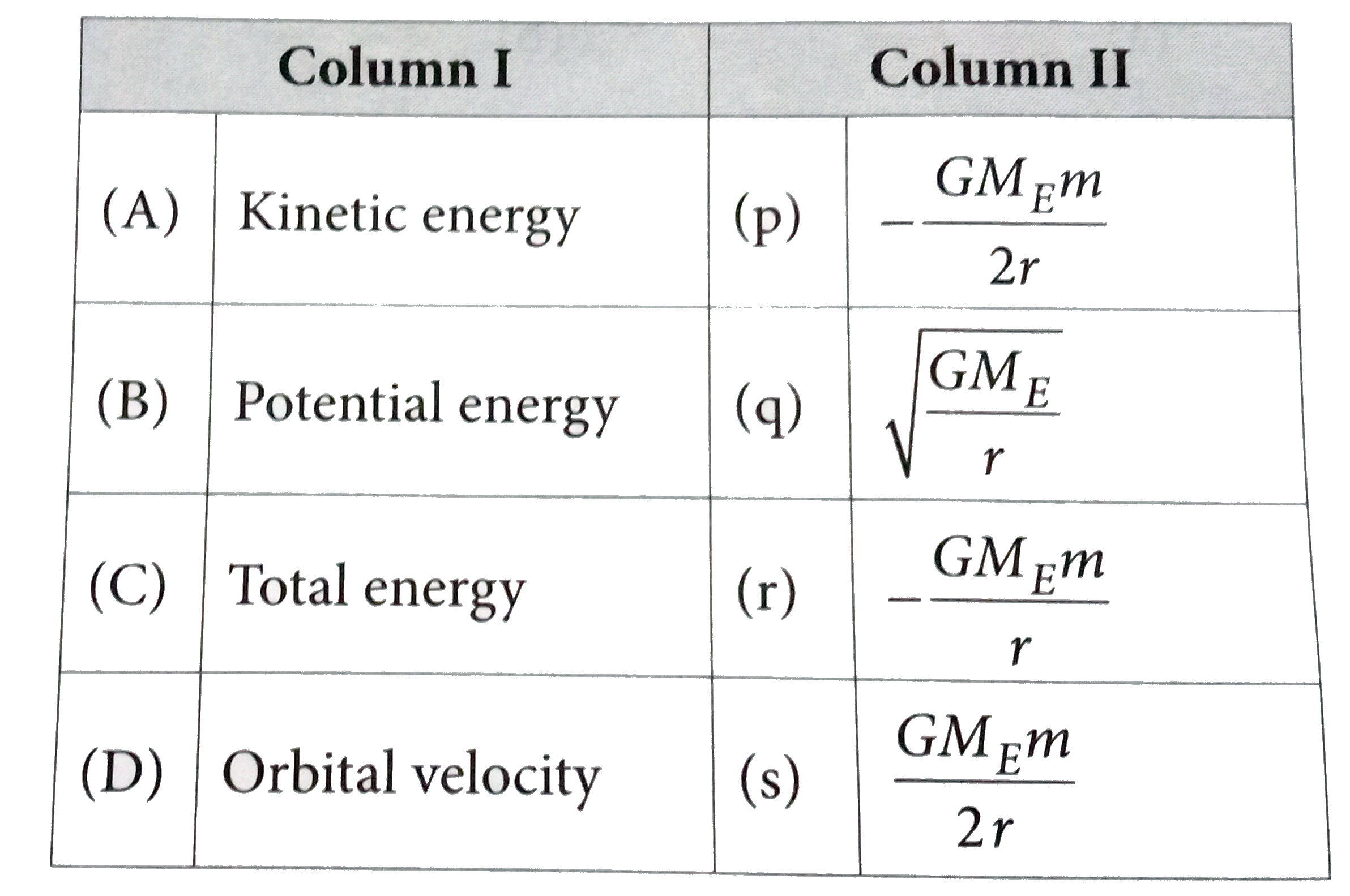
ocr_image
(where $M_{E}$ is the mass of the earth , m is the mass of the satellite and r is the radius of the orbit )

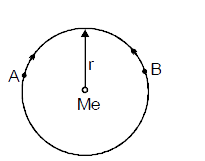
View Solution

Consider two satellites A and B of equal mass m, moving in the same circular orbit of radius r around the earth E but in opposite sense of rotation and therefore on a collision course (see figure).
In terms of G, Me, m and r find the total mechanical energy EA + EB of the two satellite plus earth system before collision.
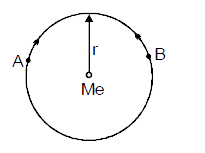
ocr_image

View Solution

Consider two satellites A and B of equal mass m, moving in the same circular orbit of radius r around the earth E but in opposite sense of rotation and therefore on a collision course (see figure).
If the collision is completely inelastic so that wreckage remains as one piece of tangle d material (mass = 2m), find the total mechanical energy immediately after collision.
ocr_image

View Solution

एक उपग्रह पृथ्वी के चारों ओर त्रिज्या r की कक्षा में चाल v से घूम रहा है | यदि उपग्रह का द्रव्यमान M है तो उसकी कुल ऊर्जा होगी :

View Solution

m, द्रव्यमान का एक उपग्रह पृथ्वी के चारों ओर r त्रिज्या के वृत्तीय मार्ग में परिक्रमा कर रहा है । यदि पृथ्वी का द्रव्यमान $M_{e}$ हो तो सिद्ध कीजिए कि उपग्रह का कोणीय संवेग $\sqrt{G m^{2} M_{e} r}$ होगा ।

View Solution

m द्रव्यमान का एक उपग्रह,M द्रव्यमान के ग्रह के चारों ओर R त्रिज्या की वृत्तीय कक्षा में घूम रहा है, तो इसकी कुल ऊर्जा E होगी :

View Solution

A satellite of mass m is moving in a circular orbit of radius r = (R_(e) + h) around earth of radius R_(e) and mass M_(e) , and density of earth rho . Match the following

View Solution

The correct Answer is:(i). $\frac{- G m M_{e}}{r}$
(ii). $- \frac{2 G m M_{e}}{r}$
(iii). Free fall

Topper's Solved these Questions

Similar Questions

ALLEN -GRAVITATION-Exercise 4 B (Brain Storming Subjective Exercise)

The correct Answer is:(i). −GmMer (ii). −2GmMer (iii). Free fall

By applying conservation of linear momentum m(v)−m(v)=2mv',v'=0 Initially energy of a satellite 'A' and 'B' is EA=−GMem2R,EB=−GMem2R Total enegy EA+EB=−GMemR After collision velocity of satellite becomes zero then the K.E.=0 therefore total mechanical energy becomes −GMe2MR

Topper's Solved these Questions

Similar Questions

ALLEN -GRAVITATION-Exercise 4 B (Brain Storming Subjective Exercise)

The correct Answer is:(i). $\frac{- G m M_{e}}{r}$
(ii). $- \frac{2 G m M_{e}}{r}$
(iii). Free fall