A ball collides with a frictionless wall with velocity u as shown in the figure. Coefficient of restitution for the impact is e.
(a) Find expression for the velocity of the ball immediately after the impact.
(b) If impact is perfectly elastic what do you observe?
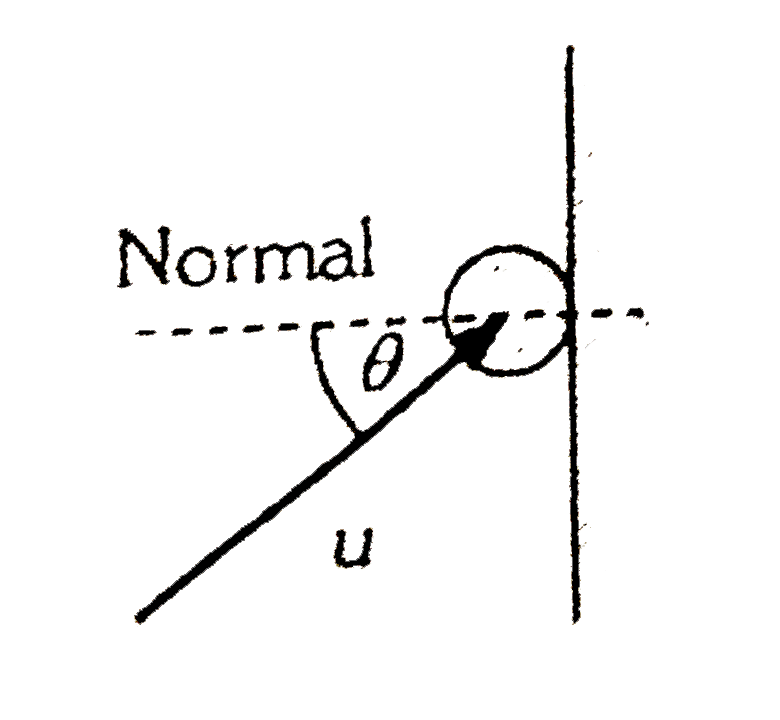 Solution in Gujrati
Solution in Gujrati
(a) Let us consider the ball as the body A and the wall as the body B. Since the wall has infinte,y large inertia (mass) as compared to the ball, the state of motion of the wall, emains unaltered during the impact i.e. the wall remain stationary.
Now we show velocities of the ball and its t and n-components immediately before and after the impact. For the purpose we have assumed velocity of the ball after the impact v.
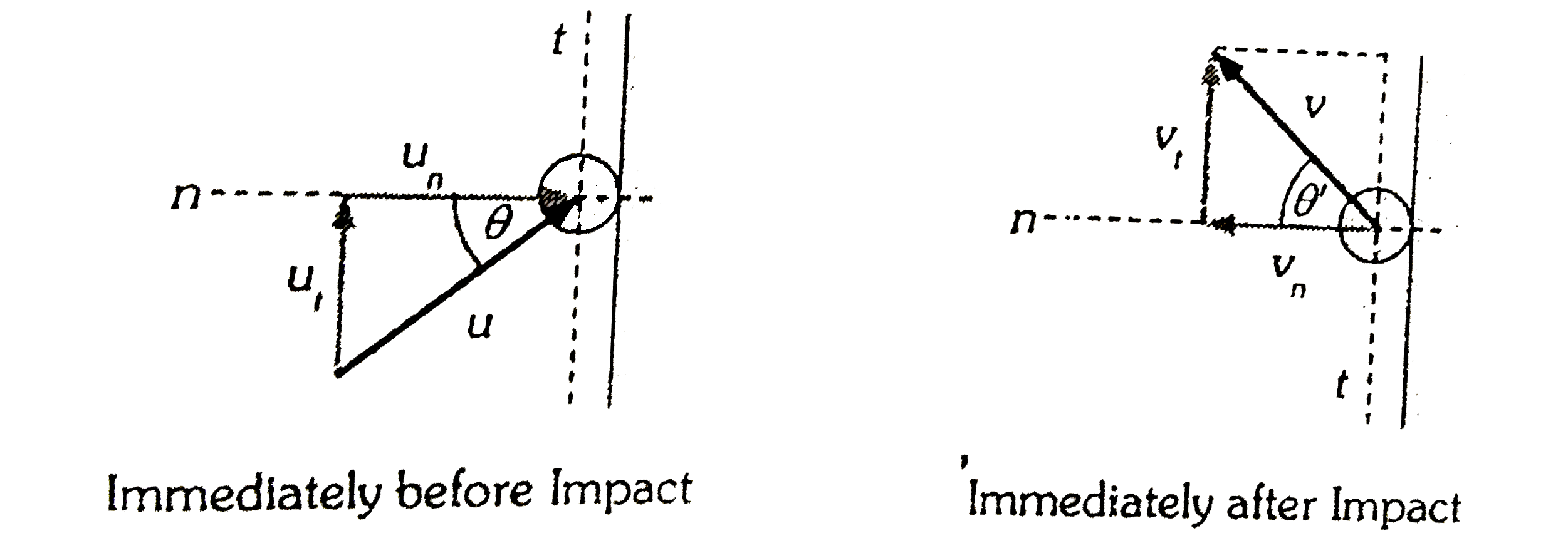
Component along t-axis Components of momentum along t-axis of the ball is conserved. Hence, t-component of velocities of each of the bodies remains unchanged. ...(i)
Component along -axis Concept of coefficient of restitution e is applicable only for the n-component velocities.
,
From equations (i) and (ii), the t and n-components of velocity of the ball after the impact are
and
(b) If the impact is perfectly elastic, we have and The ball will rebound with the same speed making the same angle with the vertical at which it has collided. In other words, a perfectly elastic collsion of a ball with a wall follows the same laws as light follows in reflection at a plane mirror.
Topper's Solved these Questions
- CENTRE OF MASSBOOK - ALLEN CHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-I
40 Videos
- CENTRE OF MASSBOOK - ALLEN CHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-II
43 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLEN CHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3 Videos
Similar Questions
The centre of mass of a non uniform rod of length L whoose mass per unit length varies as p=kx2/L, (where k is a constant and x is the distance measured form one end) is at the following distances from the same end
View SolutionTwo particle A and B start moving due to their mutual interaction only. If at any tiem 't', →aA and →aB are their respective accelerations, →vA and →vB are their respective velocities, and upto that time WA and WB are the work done on A and B respectively by the mutual force, mA and mB are their masses respectively, then which of the following is alwys correct.
View SolutionAssertion: If the initial and final positions coincide, the displacement is a null vector.
Reason: A physical quantity can not be called a vector, if its magnitude is zero.
View SolutionTwo soap bubbles A and B are kept in a closed chamber where the air is maintained at pressure 8N/m2.The radii of bubbles A and B are 2cm and 4cm, respectively. Surface tension of the soap-water used to make bubbles is 0.04N/m. Find tha ratio nB/nA, where nA and nB are the number of moles of air in bubbles A and B, respectively. [Neglect the effect of gravity.]
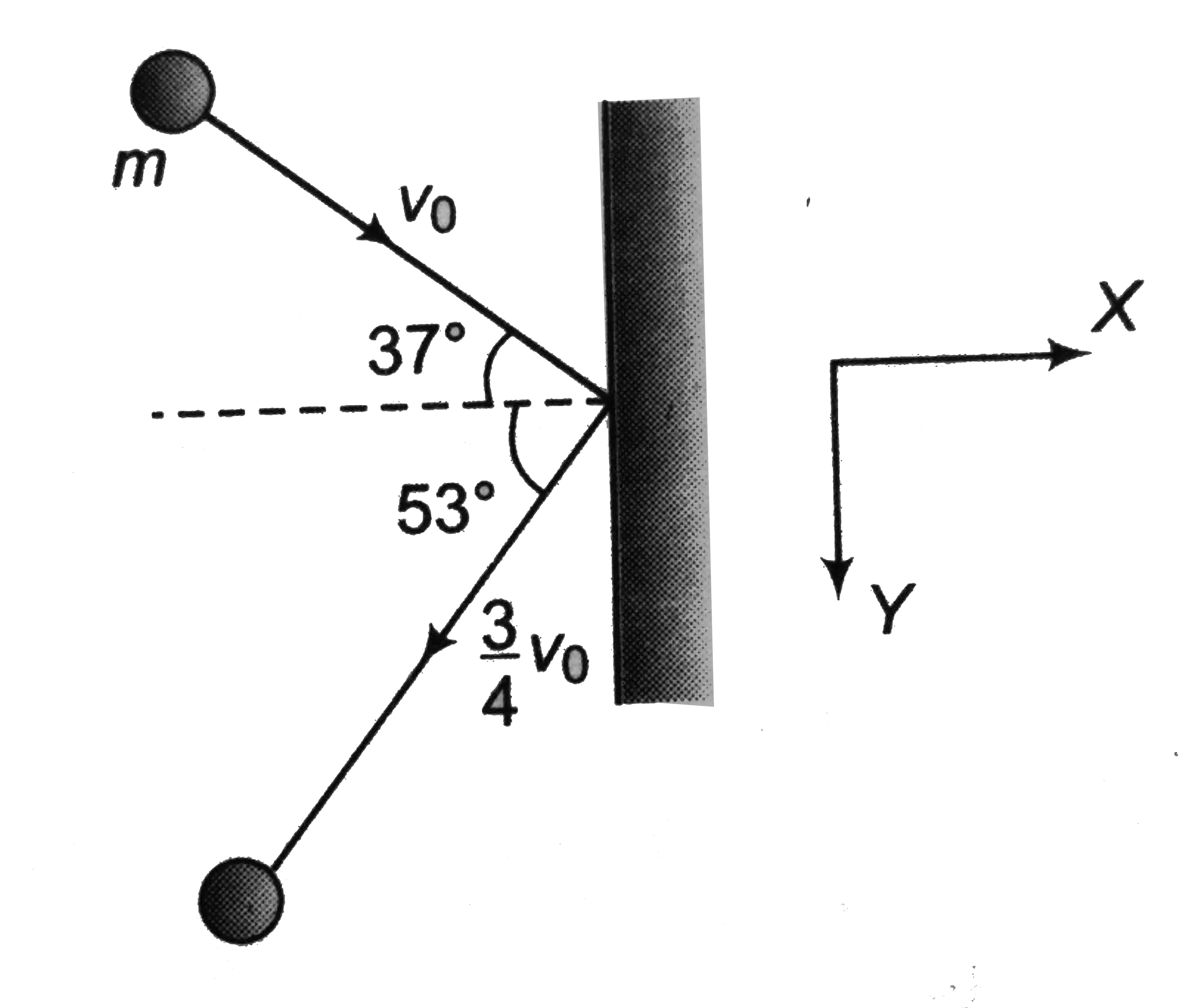
View SolutionA ball of mass m moving with velocity v0 collides a wall as shown in figure. After impact it rebounds with a velocity 34v0. The impulse acting on ball during impact is
View SolutionA ball collides impinges directly on a similar ball at rest. The first ball is brought to rest after the impact. If half of the kinetic energy is lost by impact, the value of coefficient of restitution ( e) is
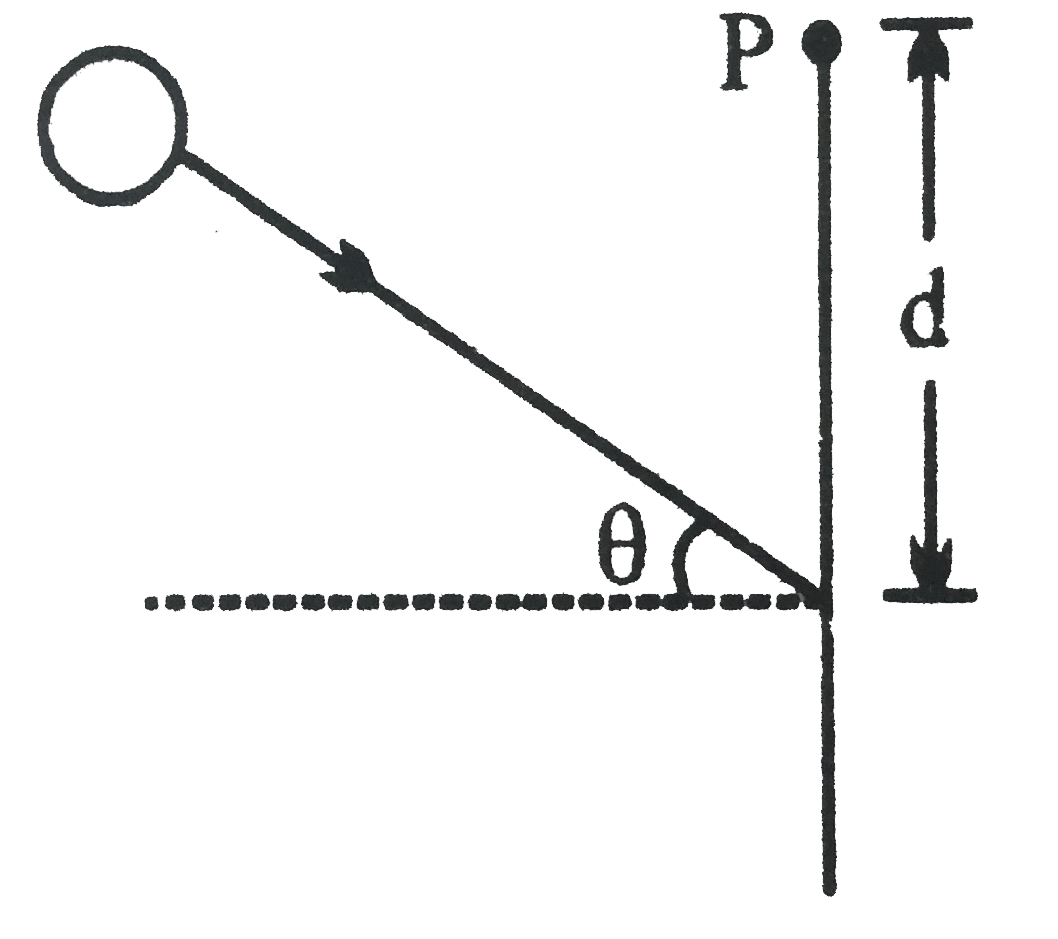
View SolutionA ball of mass m moving with velocity v, collide with the wall elastically as shown in the figure. After impact the change in angular momentum about P is :
 .
.
View SolutionA ping-pong ball strikes a wall with a velocity of 10ms−1. If the collision is perfectly elastic, find the velocity if ball after impact
View SolutionA disk sliding with velocity u on a smooth horizontal plane strikes another identical disk kept at rest as shown in the figure. If the impact between the disks is perfectly elastic impact, find velocities of the disks after the impact.
View SolutionA smooth ball A travels towards another identical ball B with a velocity u. Ball B is at rest and the impact parameter d is equal to sqrt(3) R where R is radius of each ball. Due to impact the direction of motion of ball A changes by 30^(@) . Find the velocity of B after the impact. It is given that collision is elastic
View SolutionA ball moving with a velocity v strikes a wall moving toward the wall with a velocity u. An elastic impact occurs. Determine the velocity of the ball after the impact. What is the cause of the change in the kinetic energy of the ball ? Consider the mass of the wall to be infinitely great.
View SolutionThe ball A collides elastically with an identical ball B with a speed v. The ball B touches another identical ball C. The velocity of C, after the impact, is equal to
View SolutionA ball moving with a velocity v strikes a wall moving towards the ball with a velocity u. An elastic impact occurs. Determine the velocity of ball after the impact. What is the cause of change in kinetic energy of the ball? Consider the mass of the wall to be infinitely large.
View SolutionA ball of mass m moving with velocity v0 collides a wall as shown in figure. After impact it rebounds with a velocity 34v0. The impulse acting on ball during impact is

View SolutionA ball collides impinges directly on a similar ball at rest. The first ball is brought to rest after the impact. If half of the kinetic energy is lost by impact, the value of coefficient of restitution ( e) is
View SolutionA ball moving with a velocity v hits a massive wall moving towards the ball with a velocity a. An elastic impact lasts for time △t
View Solution
