Particle is dropped from the height of 20 m from horizontal ground. A constant force acts on the particle in horizontal direction due to which horizontal acceleration of the particle becomes 6m/s2. Find the horizontal displacement of the particle till it reaches ground.Solution in Gujrati
6m
10m
12m
24m
The correct Answer is:C
Time to reach the ground =√2×2010=2 sec
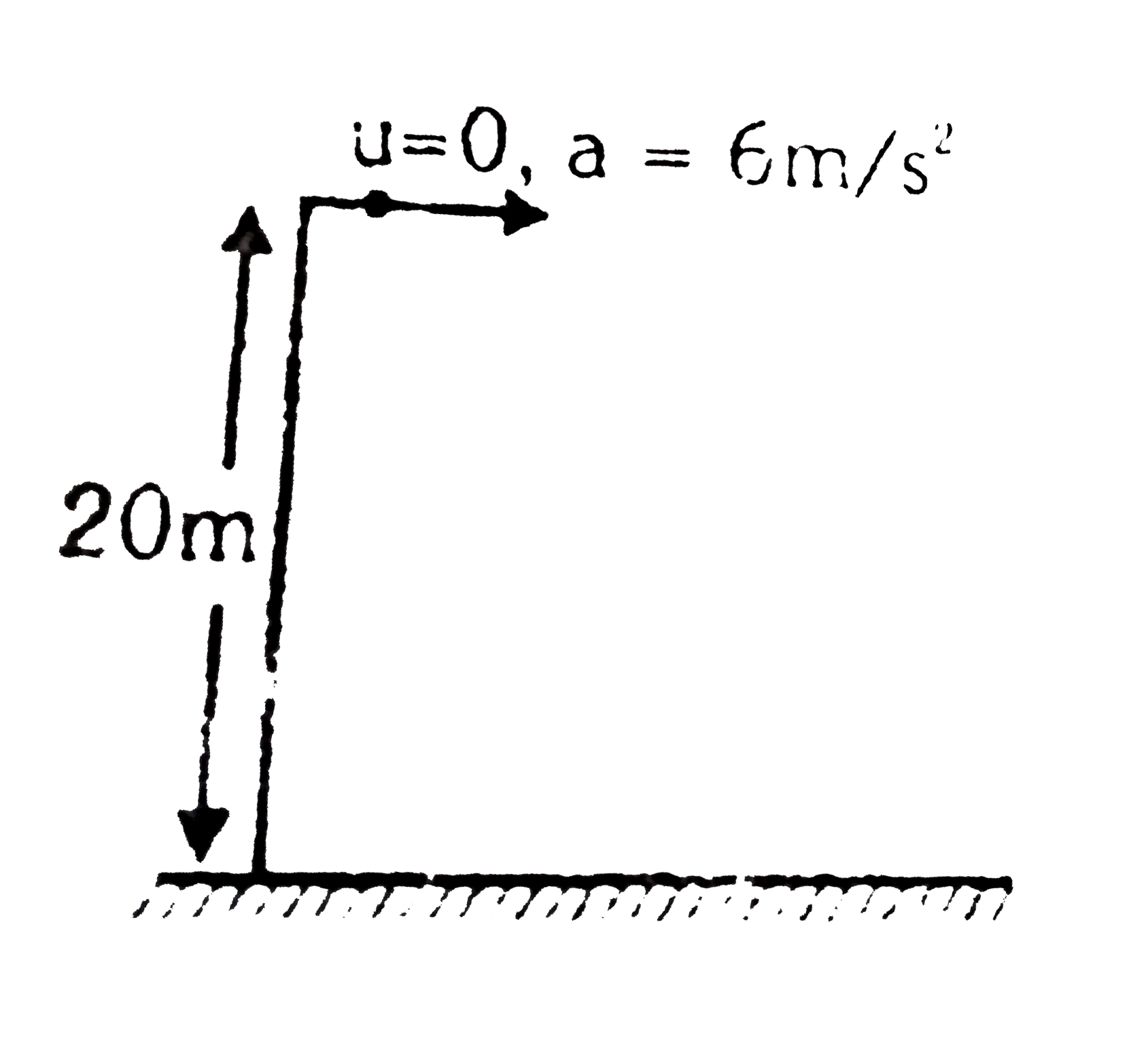
So horizontal displacement =0+12×6×4=12m
Topper's Solved these Questions
- BOOK - ALLEN CHAPTER - KINEMATICSEXERCISE - EXERCISE-0257videos
KINEMATICS
- BOOK - ALLEN CHAPTER - KINEMATICSEXERCISE - EXERCISE-036videos
KINEMATICS
- BOOK - ALLEN CHAPTER - KINEMATICSEXERCISE - EXERCISE-289videos
KINEMATICS
- BOOK - ALLEN CHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)22videos
ERROR AND MEASUREMENT
- BOOK - ALLEN CHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-78videos
KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)
Similar Questions
A particle is moving in a plane with velocity given by →u=u0ˆi+(aωcosωt)ˆj, where ˆi and ˆj are unit vectors along x and y axes respectively. If particle is at the origin at t=0. Calculate the trajectory of the particle :-
02:09View SolutionThe equation of one dimensional motion of the particle is described in column I. At t=0, particle is at origin and at rest. Match the column I with the statement in Column II.
Column IColumn II(A)x=(3t2+2)m(p)Velocity of particle at t=1s is 8m/s(B)v=8tm/s(q)Particle moves with uniform acceleration(C)a=16t(r)Particle moves with variable acceleration(D)v=6t−3t2(s)Particle will change its direction some time05:17View SolutionA student performs an experiment to determine how the range of a ball depends on the velocity with which it is projected. The "range" is the distance between the points where the ball lends and from where it was projected, assuming it lands at the same height from which it was projected. It each trial, the student uses the same baseball, and launches it at the same angle. Table shows the experimental results.
∣∣ ∣ ∣ ∣ ∣ ∣∣TrailLaunch speed(m/s)Range(m)110822031.833070.7440122.5∣∣ ∣ ∣ ∣ ∣ ∣∣
Based on this data, the student then hypothesizes that the range, R, depends on the initial speed v0 according to the following equation : R=Cvn0, where C is a constant and n is another constant.
Based on this data, the best guess for the value of n is :-View SolutionA cube has a side of length 1.2×10−2m. Calculate its volume.
01:21View SolutionA football player kicks a ball at an angle of 30∘ with an initial speed of 20 m/s. Assuming that the ball travels in a vertical plane, calculate (a) the time at which the ball reaches the highest point (b) the maximum height reached (c) the horizontal range of the ball (d) the time for which the ball is in the air, (g=10m/s2)
03:32View SolutionA particle is dropped from a height h. Another particle which is initially at a horizontal distance d from the first is simultaneously projected with a horizontal velocity u and the two particles just collide on the ground. Then
06:01View SolutionA particle is released from a certain height H = 400m . Due to the wind, the particle gathers the horizontal velocity component v_x = ay "where a "= (sqrt5)s^(-1) and y is the vertical displacement of the particle from the point of release, then find (a) the horizontal drift of the particle when it strikes the ground , (b) the speed with which particle strikes the ground.
04:36View SolutionA particle is dropped from a tower in a uniform gravitational field at t=0. The particle is blown over by a horizontal wind with constant velocity. The slope (m) of the trajectory of the particle with horizontal and its kinetic energy vary according to curves. Here, x is the horizontal displacement and h is the height of particle from ground at time t.
02:40View SolutionParticle is dropped form the height of 20m form horizontal ground. There is wind blowing due to which horizontal acceleration of the particles becomes 6ms−2. Find the horizontal displacement of the particle till it reaches ground.
View SolutionA particle A is projected from the ground with an initial velocity of 10m/s at an angle of 60∘ with horizontal. From what height should an another particle B be projected horizontally with velocity 5m/s so that both the particles collide in ground at point C if both are projected simultaneously g=10m/s2.
 05:42View Solution
05:42View SolutionA particle is projected from the ground at an angle of 60∘ with horizontal at speed u=20m/s. The radius of curvature of the path of the particle, when its velocity. makes an angle of 30∘ with horizontal is :
(g=10m/s203:50View SolutionVelocity of a projectile at height 15m from ground is v=(20ˆi+10ˆj)m/s. Here ˆi is in horizontal direction and ˆj is vertically upwards. Then
Speed with which particle is projected from ground is……… m/s03:04View SolutionAssertion Particle-1 is dropped from a tower and particle-2 is projected horizontal from the same tower. Then both the particles reach the ground simultaneously.
Reason Both are particles strike the ground with different speeds.06:20View SolutionA particle is projected from horizontal ground with speed 5ms−1 at 53∘ with horizontal. Find time after which velocity of particle will be 45∘ with horizontal:
05:21View SolutionA particle is projected horizontally from a tower with velocity V0. Wind is blowing in opposite direction and is providing a constant horizontal acceleration a0. If particle strikes the ground vertically then height of building is
 02:47View Solution
02:47View Solutionक्षैतिज धरातल से 20m ऊँचाई से एक कण मुक्त किया जाता है। कण पर क्षैतिज दिशा में स्थिर बल कार्य कर रहा है जिसके कारण क्षैतिज त्वरण 6ms−2 उत्पन्न होता है। धरातल पर पहुचने पर कण का क्षैतिज विस्थापन ज्ञात करो
06:18View Solution

