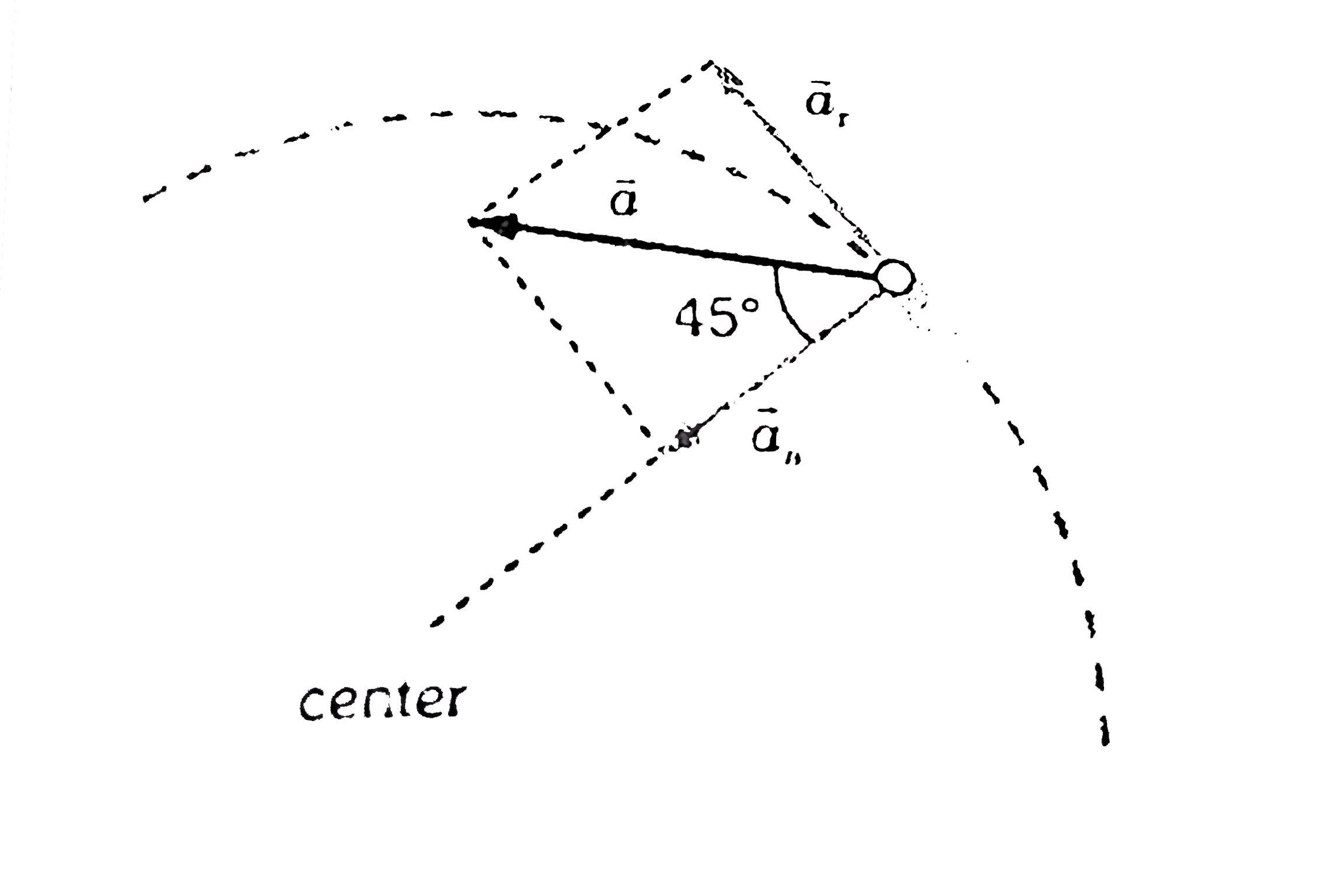
A particle is moving in a circular orbit with a constant tangential acceleration. After a certain time t has elapsed after the beginning of motion, the between the total acceleration a and the direction along the radius r becomes equal to 45∘. What is the angular acceleration of the particle.Solution in Gujrati
The correct Answer is:1t2
at=ar,αr=ω2r,α=α2t∘⇒α=1t2
Topper's Solved these Questions
- BOOK - ALLEN CHAPTER - KINEMATICSEXERCISE - Exercise-04[B]14videos
KINEMATICS
- BOOK - ALLEN CHAPTER - KINEMATICSEXERCISE - Exercise-05 [A]11videos
KINEMATICS
- BOOK - ALLEN CHAPTER - KINEMATICSEXERCISE - Comprehension#73videos
KINEMATICS
- BOOK - ALLEN CHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)22videos
ERROR AND MEASUREMENT
- BOOK - ALLEN CHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-78videos
KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)
Similar Questions
Two towns A and B are connected by a regular bus service with a bus leaving in either direction every T minutes. A man cycling with a speed of 20kmh−1 in the direction A to B notices that a bus goes past him every 18 min in the direction of his motion, and every 6 min in the opposite direction. What is the period T of the bus service and with what speed (assumed constant) do the buses ply on the road ?
01:35View SolutionA ball whose kinetic energy is E , is projected at an angle of 45∘ to the horizontal . The kinetic energy of the ball at the highest point of its flight will be
02:04View SolutionA projectile is projected with some initial velocity and some initial angle of projection. A wind is also blowing, due to which constant horizontal retardation a is imparted to the particle in the plane of motion. It is found that, the particle is at same height at two different time t1 & t2 and particle is at same horizontal distance at two different time t3 & t4
Angle of projection of particle is :-02:35View SolutionA cube has a side of length 1.2×10−2m. Calculate its volume.
03:51View SolutionA football player kicks a ball at an angle of 30∘ with an initial speed of 20 m/s. Assuming that the ball travels in a vertical plane, calculate (a) the time at which the ball reaches the highest point (b) the maximum height reached (c) the horizontal range of the ball (d) the time for which the ball is in the air, (g=10m/s2)
03:42View SolutionA point moves along a circle having a radius 20cm with a constant tangential acceleration 5cm//s^(2) . How much time is needed after motion begins for the normal acceleration of the point to be equal to tangential acceleration?
03:06View SolutionA point mass moves along a circle of radius R with a constant angular acceleration alpha . How much time is needed after motion begins for the radial acceleration of the point mass to be equal to its tangential acceleration ?
02:02View SolutionA particle is moving in a circular orbit when a constant tangential acceleration. After 2s from the beginning of motion, angle between the total acceleration vector and the radius E becomes 45∘. What is the angular acceleration of the particle?
 05:11View Solution
05:11View SolutionA particle is moving in circular path with constant acceleration. In time t after the beginning of motion the direction of net acceleration is at 30∘ to the radius vector at that instant. The angular acceleration of the particle at that time t is
02:24View SolutionA particle starts moving with a constant angular acceleration in a circular path. The time at which the magnitudes of tangential and radial acceleration are equal is
01:47View SolutionA particle moves along circle of radius (20π) m with constant tangential acceleration. If the speed of the particle is 80m/s at the end of the second revolution after motion has begun, the tangential acceleration is
View SolutionA particle is moving in a circular orbit with fixed tactile acceleration. 2S after starting speed, the angle between the total thrust vector and radius R 45^(@) it happens. What is the particle acceleration of a particle?
02:43View SolutionA particle moves along a circle if radius (20 //pi) m with constant tangential acceleration. If the velocity of the particle is 80 m//s at the end of the second revolution after motion has begun the tangential acceleration is .
02:36View SolutionA point moves along a circle having a radius 20cm with a constant tangential acceleration 5cm/s2 . How much time is needed after motion begins for the normal acceleration of the point to be equal to tangential acceleration?
08:16View SolutionA particle moves along a circle if radius (20 //pi) m with constant tangential acceleration. If the velocity of the particle is 80m/s at the end of the second revolution after motion has begun the tangential acceleration is .
03:03View SolutionA particle moves along a circle of radius (20/pi) m with constant tangential acceleration. If the speed of the particle is 80 m/s at the end of the second revolution after motion has begun, the tangential acceleration is
03:12View Solution

