A conical pendulum is moving in a circle with angular velocity ω. If tension in the string is T, which of following equations are correct ?Solution in Gujrati
T=mω2l
Tsinθ=mω2l
T=mgcosθ
T=mω2lsinθ
The correct Answer is:A
Step by step video & image solution for A conical pendulum is moving in a circle with angular velocity omega. If tension in the string is T, which of following equations are correct ? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - ALLEN CHAPTER - CIRCULAR MOTIONEXERCISE - MCQ
14 Videos
- CIRCULAR MOTIONBOOK - ALLEN CHAPTER - CIRCULAR MOTIONEXERCISE - Martrix match
1 Videos
- CIRCULAR MOTIONBOOK - ALLEN CHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE(S-2)
6 Videos
- BASIC MATHSBOOK - ALLEN CHAPTER - BASIC MATHSEXERCISE - Question
1 Videos
- CURRENT ELECTRICITYBOOK - ALLEN CHAPTER - CURRENT ELECTRICITYEXERCISE - EX.II
66 Videos
Similar Questions
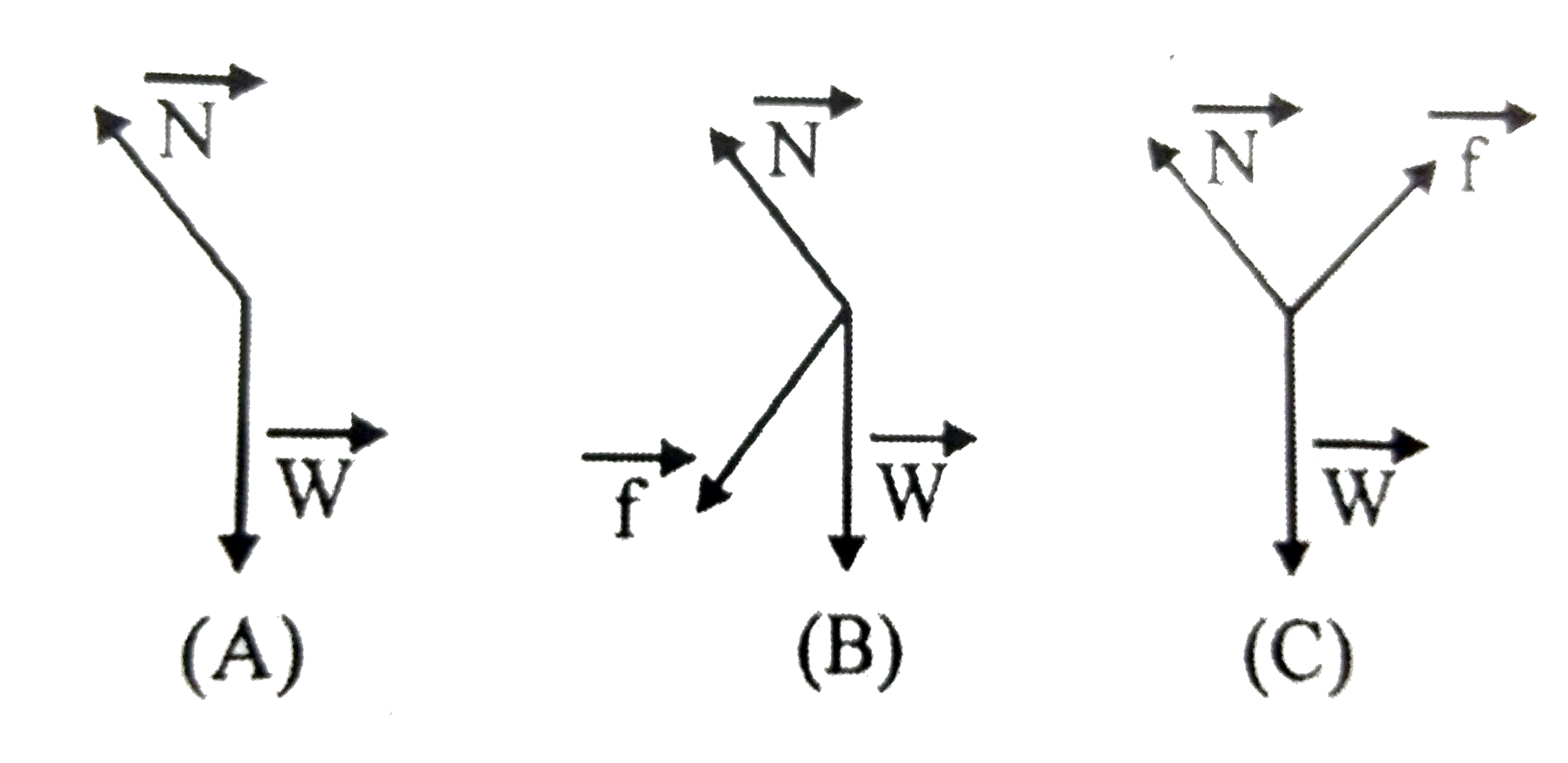
A car is moving with constant speed on a rough banked road.

Figure shows the free body diagram of car in three situation A, B & C respectively:
View SolutionA block is placed on a horizontal table which can rotate about its axis.The block is placed at certain distance from centre as shown in figure. Table rotates such that particle does not slide. See possible direction of net acceleration of block at the instant shown in figure
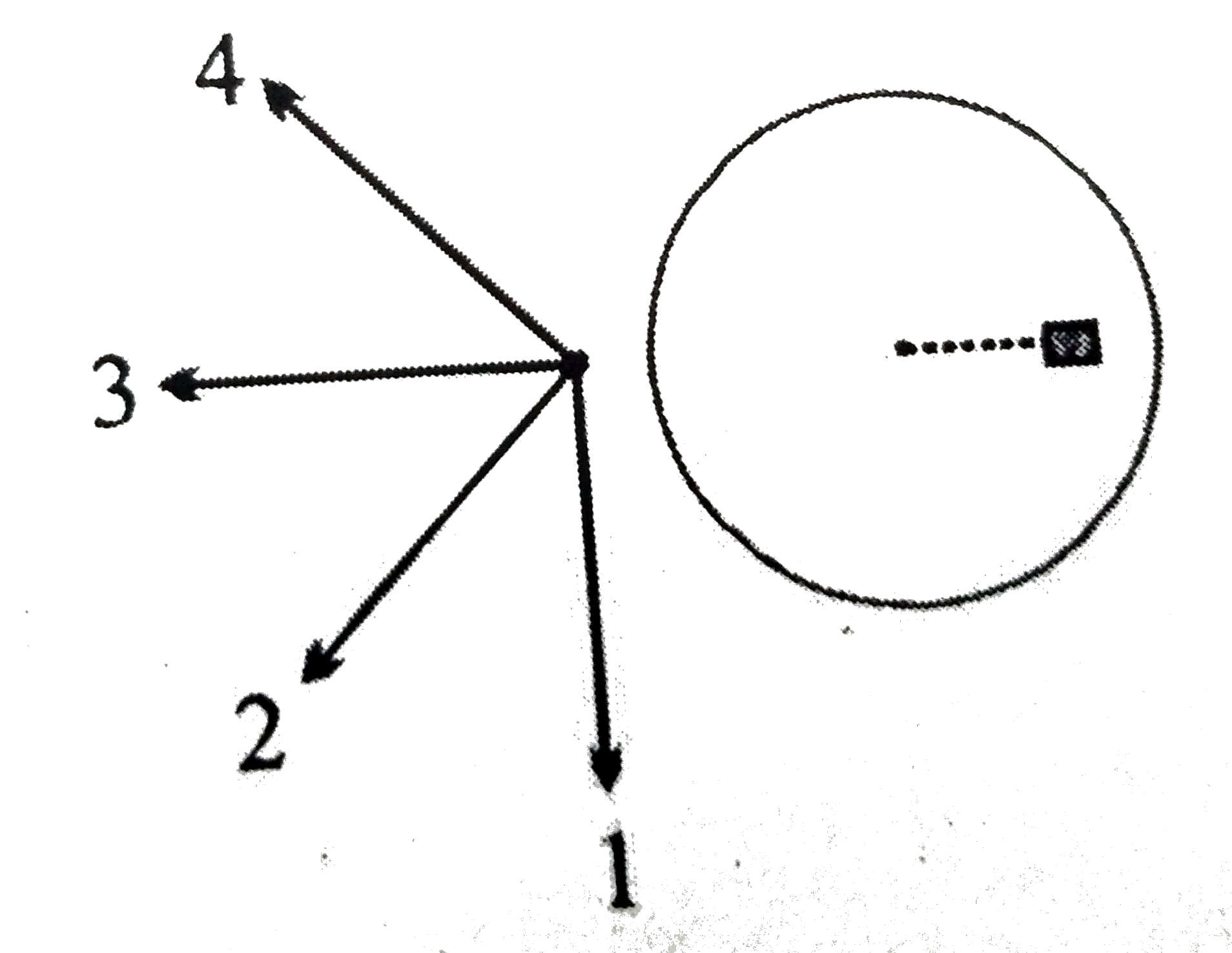
Column-IColumn-II(A)When rotation is clockwise with constant ω(P)1(B)When rotation is clock wise with decreasing ω(Q)2(C)When rotation is clockwise with increasing ω(R)3(D)Just after clockwise rotation beings from rest(S)4
View SolutionA stone is launched upward at 45∘ with speed v0. A bee follow the trajectory of the stone at a constant speed equal to the initial speed of the stone.
(a) Find the radius of curvature at the top point of the trajectory.
(b) What is the acceleration of the bee at the top point of the trajectory? For the stone, neglect the air resistance.
View SolutionFind dydx for the following :
(i) y=x7/2 (ii) y=x−3
(iii)y=x (iv) y=x5+x3+4x1/2+7
(v) y=5x4+6x3/2+9x (vi) y=ax2+bx+C
(Vii)y=3x^(5)-3x-1/x
View SolutionIn the Bohr's model of hy drogen atom, the electron moves around the nucleus in a circular orbit of radius 5×10−11m. Its time period is 1.5×10−16s. The current associated with the electron motion is:-
View SolutionShow that the angle made by the string with the vertical in a conical pendulum is given by cosθ=gLω2 , where L is the string and ω is the angular speed.
View SolutionA conical pendulum consists of a simple pendulum moving in a horizontal circle as shown in the figure. C is the pivot, O the centre of the circle in which the pendulum bob moves and omega the constant angular velocity of the bob. If vecL is the angular momentum about point C , then
View SolutionConsider a conical pendulum having bob is mass m is suspended from a ceiling through a string of length L. The bob moves in a horizontal circle of radius r. Find (a) the angular speed of the bob and (b) the tension in the string.
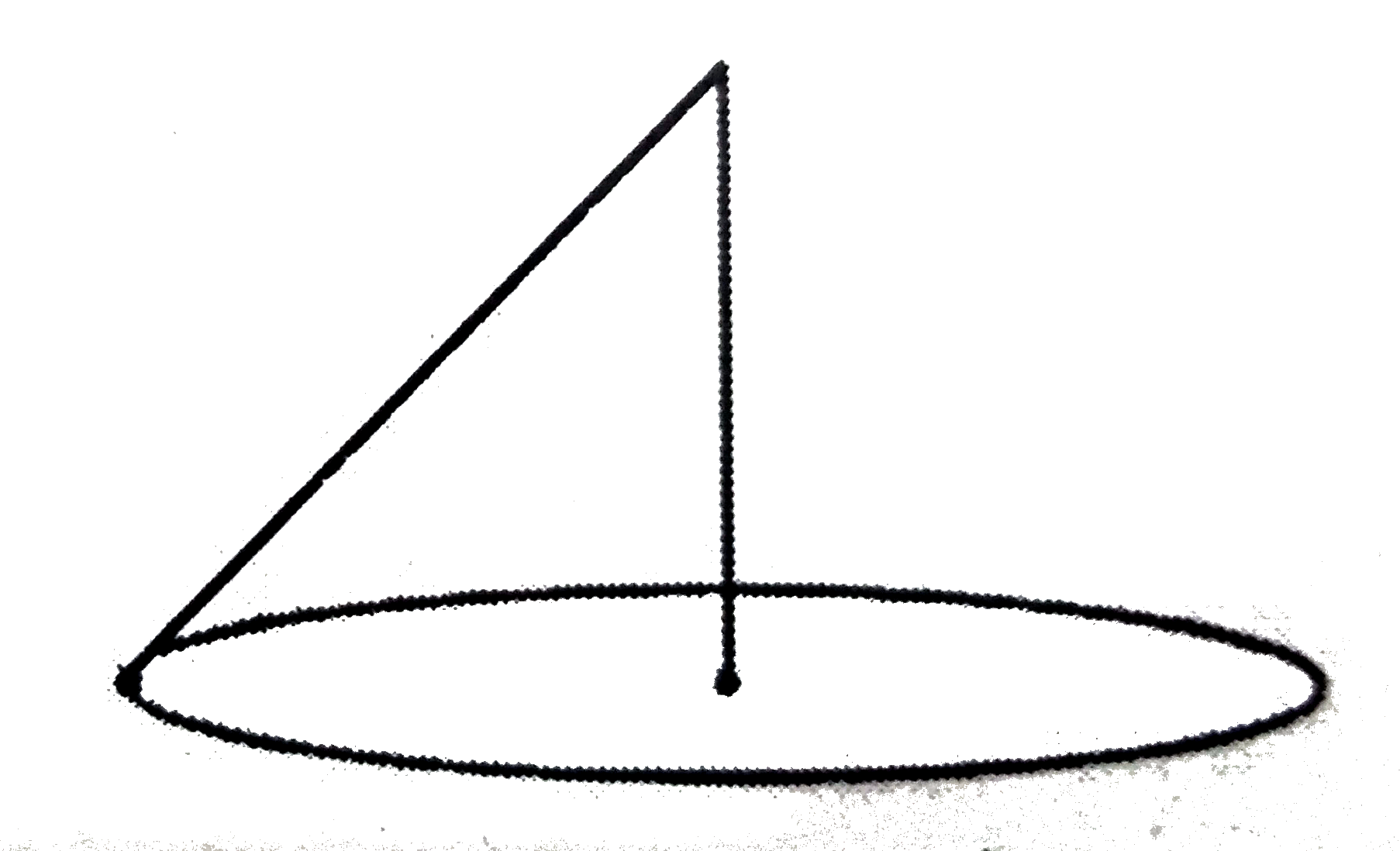
View SolutionA conical pendulum is moving in a circle with angular velocity omega as shown. If tension in the string is T, which of following equations are correct ?
View SolutionFor a conical pendulum of string length L, its angular speed is proportional to
View SolutionThe work done by the tension in the strings of a simple pendulum in one complete oscillation is equal to
View SolutionA stone is tied to one end of a steing and rotated in horizontal circle with a uniform angular velocity. The tension in the string is T, if the lrngth of the string is halved and its angular velocity is doubled , the tension in the string will be
View SolutionA mass attached to a string rotates about a fixed centre with an angular velocity omega in a horizontal plane ,The length of the string and the angular velocity are now doubled .IF T_(0) is the initial tension in the string , then the new tension will be
View SolutionThe maximum tension in the string of an oscillating simple pendulum is 3% more than the minimum tension in the string. The angular amplitude of oscillations of the pendulum is
View SolutionThe conical pendulum is in steady circular motion with constant angular velocity ω as shown in Fig. Mass of the particle is M and string makes angle α with vertical.
(a) Find angular momentum of the particle about the center C of the circle and the hinge O about which the string is attached.
(b) Also check whether L is changing or is constant.
View Solutionएक शकूकार लोलक (conical pendulum) चित्रानुसार स्थिर कोणीय वेग ω से वृत्ताकार पथ पर घूम रहा है । घागे में तनाव T का मान होगा ?

View Solution
