A ball is projected horizontally. After 3s from projection its velocity becomes 1.25 times of the velocity of projection. Its velocity of projection isSolution in Gujrati
10 m/s
20 m/s
30 m/s
40 m/s
The correct Answer is:D
Step by step video & image solution for A ball is projected horizontally. After 3s from projection its velocity becomes 1.25 times of the velocity of projection. Its velocity of projection is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ALLEN CHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)47videos
KINEMATICS-2D
- BOOK - ALLEN CHAPTER - KINEMATICS-2DEXERCISE - Exercise (S-2)14videos
KINEMATICS-2D
- BOOK - ALLEN CHAPTER - GRAVITATIONEXERCISE - EXERCISE 49videos
GRAVITATION
- BOOK - ALLEN CHAPTER - MOTION IN A PALNEEXERCISE - SOLVED EXAMPLE28videos
MOTION IN A PALNE
Similar Questions
A particle is moving in xy-plane .At certain instant the components of its velocity and acceleration are as follows ux=3m/s,uy=4m/s,ax=2m/s2anday=1m/s2. The rate of change of speed at this moment is
11:37View SolutionA particle travels so that its acceleration is given by →a=5costˆi−3sintˆj. If the particle is located at (−3,2) at time t=0 and is moving with a velocity given by (−3ˆi+2ˆj). Find
(i) The velocity [→v=∫→a.dt] at time t and
(ii) The position vector [→r=∫→v.dt] of the particle at time t(t>0).05:44View SolutionAssertion : The difference in the value of acceleration due to gravity at pole and equator is proportional to square of angular velocity of earth.
Reason : the value of acceleration due to gravity is minimum at the equator and maximum at the pole.03:59View SolutionA particle thrown over a triangle from one end of a horizontal base falls on the other end of the base after grazing the vertex. If αandβ are the base angles of triangle and angle of projection is θ, then prove that (tanθ=tanα+tanβ)
06:37View SolutionDisplacement And Instantaneous Velocity After Horizontal Project
View SolutionA particle is projected from a point on the level ground and its height is h when at horizontal distances a and 2 a from its point of projection. Find the velocity of projection.
01:52View SolutionA ball is projected from the ground with velocity u such that its range is maximum Then
02:56View SolutionA ball is projected horizontal from the top of a tower with a velocity v_(0) . It will be moving at an angle of 60^(@) with the horizontal after time.
02:39View SolutionA ball is projected from the ground with velocity v such that its ranege is maximum.
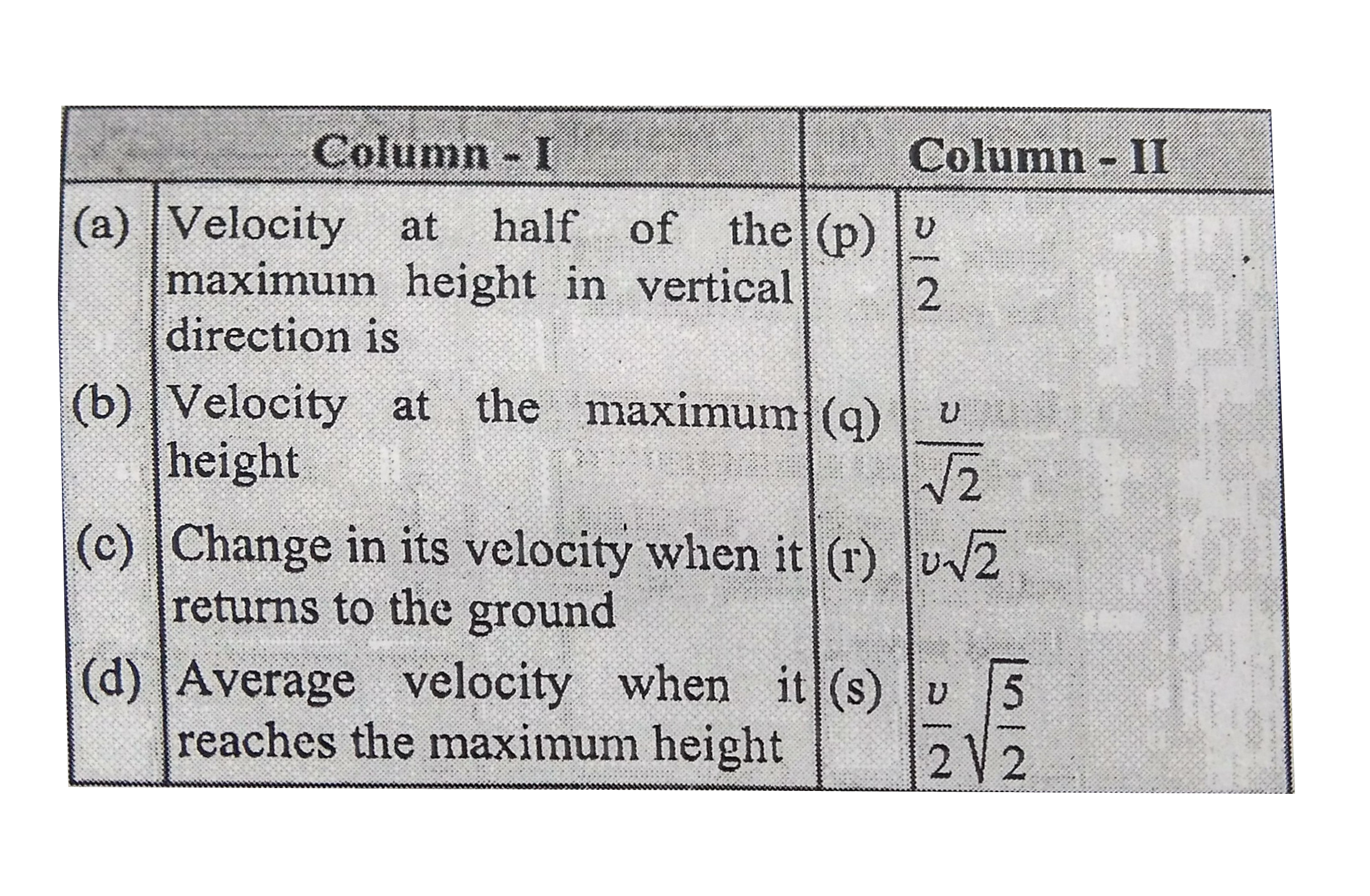 02:32View Solution
02:32View Solutionप्रक्षेप्य का प्रक्षेपण वेग दोगुना करने पर उसकी क्षैतिज परास ……………….. हो जाएगी ।
01:54View SolutionWhen a body is projected from a level ground the ratio of its speed in the vertical and horizontal direction is 4 3. If the velocity of projection is u, the time after which the ratio of the velocities in the vertical and horizontal directions are reversed is
02:32View SolutionA body is projected horizontally from the top of a tower of height 10m with a velocity 10m/s .Its velocity after 1 second is
View SolutionA stone is projected horizontally with a velocity 9.8ms−1 from a tower of height 100m. Its velocity one second after projection is
01:52View SolutionA body is projected horizontally from the top of a tower. When the body strikes the ground its velocity is V and the direction of motion makes 30° with the horizontal. The velocity of projection is
View SolutionA stone is projected horizontally with a velocity 9.8ms−1 from a tower of height 100m. Its velocity one second after projection is
View SolutionA body is projected with a velocity u at an A body is projected with a velocity u at anangle of 60^(@) to the horizontal. The time interval after which it will be moving in a direction of 30^(@) to the horizontal is
View Solution

