Find the first derivatives
(i) If y=x−1/3, find dydx
(ii) If x=t6, find dxdt
(iii) If z=u3, find dzdu
(iv) If f=r2+r1/3, find dfdr
(v) If x=2t3−3t2+5t−6, find dxdt
(vi) If y=3x2
(vii) If y=xlnx
(viii) If y=xsinx
(ix) If y=sinxcosx
(x) If y=sin2x+cos2x
(xi) If y=cos2x
(xii) If v=43πr3, find dvdr
(xiii) If s=4πr2, find dsdrSolution in Gujrati
The correct Answer is:(i)−13x−4/3
(ii)6t5
(iii)3u3
(iv)2r+13r−2/3
(v)6t2−6t+5
(vi)6x
(vii)1+lnx
(viii)xcosx+sinx
(ix)cos2x−sin2x
(x)0
(xi)−2sin2x
(xii)4πr2
(xiii)8πr
Step by step video & image solution for Find the first derivatives (i) If y=x^(-1//3)," find "(dy)/(dx) (ii) If x=t^(6)," find "(dx)/(dt) (iii) If z=u^(3)," find "(dz)/(du) (iv) If f=r^(2)+r^(1//3)," find "(df)/(dr) (v) If x=2t^(3)-3t^(2)+5t-6," find "(dx)/(dt) (vi) If y=3x^(2) (vii) If y=xlnx (viii) If y=xsinx (ix) If y=sinx cosx (x) If y=sin^(2)x+cos^(2)x (xi) If y=cos2x (xii) If v=(4)/(3)pir^(3)," find "(dv)/(dr) (xiii) If s=4pir^(2)," find "(ds)/(dr) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Integration)
12 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Graphs))
28 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Trigonometry)
22 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLEN CHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
Find the value of x1, so that ∫x10ydx=5
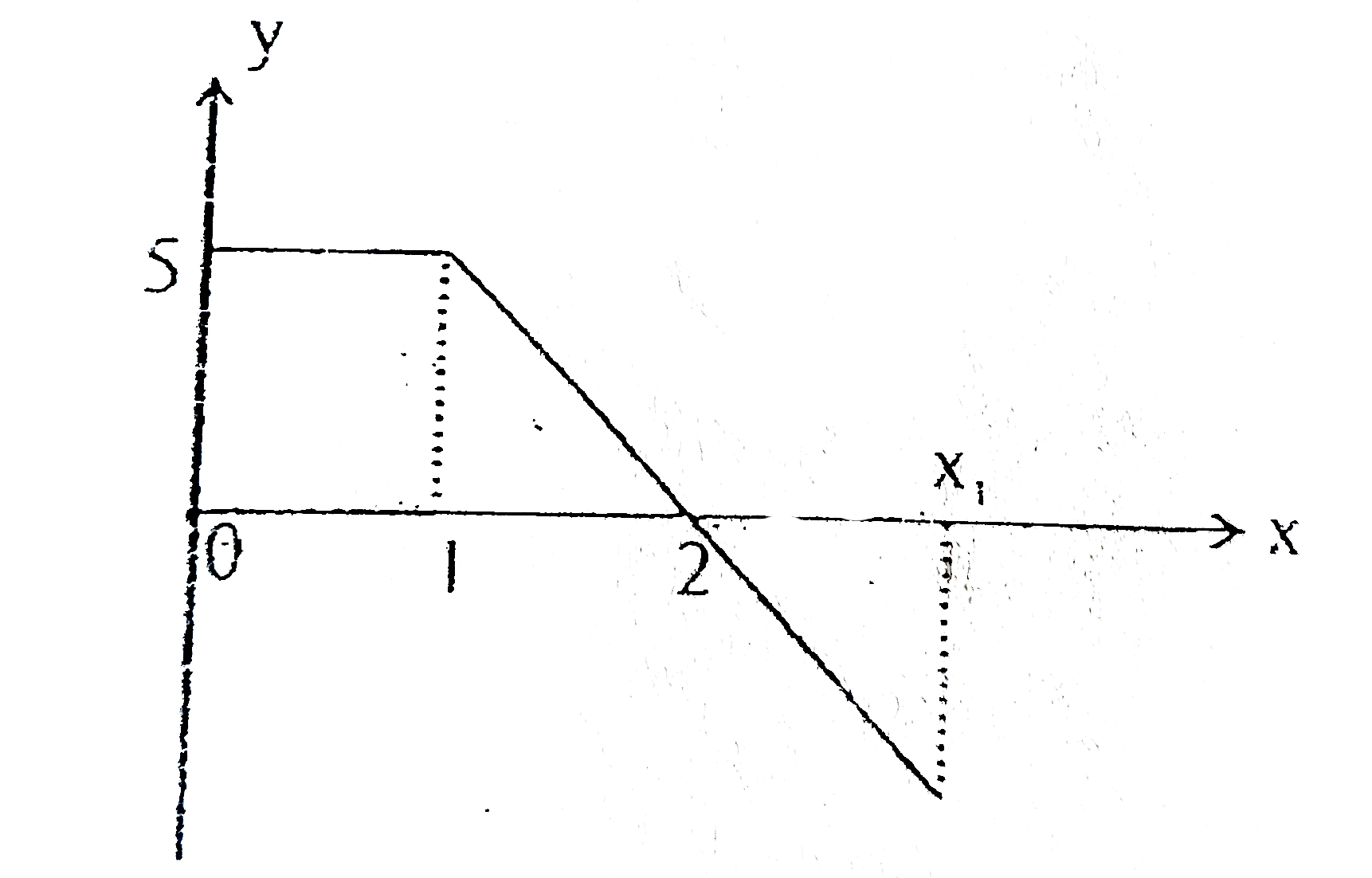
View SolutionWhich of the following equation is best representation of following graph's?
View SolutionConvert the angle from degree to radian :
(a) 30∘(b) 45∘(c) 60∘(d) 90∘(e) 120∘(f) 135∘(g) 210∘(h) 270∘(i) 315∘
View SolutionAssertion: The driver in a vechicle moving with a constant speed on a straight road is in a non-inertial frame of referance.
Reason: A reference frame in which Newton's laws of motion are applicable is non-inertial.
View SolutionA rod with rectangular cross section oscillates about a horizontal axis passing through one of its ends and it behaves like a seconds pendulum, its length will be
View Solutionif dydx=x3−3x2+1 and y =2 when x = 1 find y =?
View SolutionFind the first derivatives (i) If y=x^(-1//3)," find "(dy)/(dx) (ii) If x=t^(6)," find "(dx)/(dt) (iii) If z=u^(3)," find "(dz)/(du) (iv) If f=r^(2)+r^(1//3)," find "(df)/(dr) (v) If x=2t^(3)-3t^(2)+5t-6," find "(dx)/(dt) (vi) If y=3x^(2) (vii) If y=xlnx (viii) If y=xsinx (ix) If y=sinx cosx (x) If y=sin^(2)x+cos^(2)x (xi) If y=cos2x (xii) If v=(4)/(3)pir^(3)," find "(dv)/(dr) (xiii) If s=4pir^(2)," find "(ds)/(dr)
View Solution(i) (If) y=x2√4x+3(3x+1)2, (find) dydx निकालें|
(ii) यदि (If) y=2(−sinx)32√x,(find)dydx निकालें ।
View Solutionयदि y=1+x+x22!+x33!+x43!+..., तब dydx का मान ज्ञात कीजिएः।
View SolutionIf x3+y2=sin(x+y), find dydx
View SolutionIf y=(3x+1)2(x+4)3 find dydx.
View Solution(i). If y=x3−1, find dydx.
(ii). If y=2x2+4, find dydx
View SolutionIf y3−3xy2=x3+3x2 y , find dydx
View Solutionfind dy/dx if x3 + x2 y + x y2 + y3= 81
View SolutionFind dy/dx
x12y−12+x32y−32=0
View Solution
