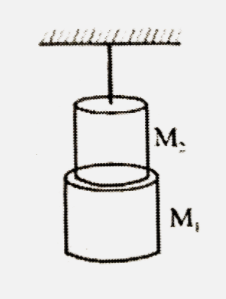
The length of wire, when M1 is hung from it, is l1 and is l2 with both M1 and M2 hanging. The natural length of wire is :-
 Solution in Gujrati
Solution in Gujrati
M1M2(l1−l2)+l1
M2l1−M1l2M1+M2
l1+l22
√l1l2
The correct Answer is:A
Step by step video & image solution for The length of wire, when M_(1) is hung from it, is l_(1) and is l_(2) with both M_(1) and M_(2) hanging. The natural length of wire is :- by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Properties of Matter & Fluid Mechanics)(Fluid Dynamics + Viscosity)
20 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Properties of Matter & Fluid Mechanics)(Hydrostatics)
20 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (GRAVITATION)
25 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLEN CHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
Blood is flowing at the rates of 200 cm3/sec in a capillary of cross-sectional area 0.5m2. The velocity of flow, (in mm/sec) is:
View SolutionA tank 5 m high is half filled with water and then is filled to top with oil of density 0.85g/cm3 The pressure at the bottom of the tank, due to these liquids is
View SolutionTwo paricles of equal mass m go round a circle of radius R under the action of their mutual gravitional attraction. The speed of each particle is
View SolutionAssertion: The driver in a vechicle moving with a constant speed on a straight road is in a non-inertial frame of referance.
Reason: A reference frame in which Newton's laws of motion are applicable is non-inertial.
View SolutionA rod with rectangular cross section oscillates about a horizontal axis passing through one of its ends and it behaves like a seconds pendulum, its length will be
View SolutionThe length of wire, when M_(1) is hung from it, is l_(1) and is l_(2) with both M_(1) and M_(2) hanging. The natural length of wire is :-
View SolutionABC is a right-angled triangle in which ∠B=90∘andBC=a. If n points L1,L2,…,Ln on AB is divided in n+1 equal parts and L1M1,L2M2,…,LnMn are line segments paralllel to BC and M1,M2,….,Mn are on AC, then the sum of the lengths of L1M1,L2M2,...,LnMn is
View SolutionABC is a right angled triangle in which ∠B=90∘ and BC=a. If n points L1,L2,…….,Ln on AB are such that AB is divided in n+1 equal parts and L1M1,L2M2,......,LnMn are line segments parallel to BC and M1,M2,M3,......,Mn are on AC, the sum of the lenghts of L1M1,L2M2,......,LnMn is
View SolutionIf (l1,m1,n1),(l2,m2,n2) are D.C's of two lines, then (l1m2−l2m1)2+(m1n2−n1m2)2+(n1l2−n2l1)2+(l1l2+m1m2+n1n2)2=
View SolutionA wire of length L is hanging from a fixed support. The length changes to L1andL2 when masses M1andM2 are suspended respectively from its free end. Then L is equal to
View SolutionIf L1=(2.02±0.01)m and L2=(1.02±0.01)m then L1+2L2 is (in m)
View Solutionएक स्थिर आधार से L लम्बाई का तार लटकाया गया है । इसके मुक्त सिरे पर द्रव्यमान M1 तथा M2 लगाये जाते है तब लम्बाई में परिवर्तन क्रमशः L1 तथा L2 हो जाता है अतः L बराबर होगा :
View SolutionABC is a right-angled triangle in which ∠B=90∘andBC=a. If n points L1,L2,…,Ln on AB is divided in n+1 equal parts and L1M1,L2M2,…,LnMn are line segments paralllel to BC and M1,M2,….,Mn are on AC, then the sum of the lengths of L1M1,L2M2,...,LnMn is
View SolutionIf (l1,m1,n1),(l2,m2,n2) are d.c's of two lines then find the value of (l1m2−l2m1)2+(m1n2−n1m2)2+(n1l2−n2l1)2+(l1l2+m1m2+n1n2)2
View SolutionIf (l1,m1,n1),(l2,m2,n2) are d.c's of two lines then find the value of (l1m2−l2m1)2+(m1n2−n1m2)2+(n1l2−n2l1)2+(l1l2+m1m2+n1n2)2
View SolutionA wire of length L is hanging from a fixed support. The length changes to L_(1) and L_(2) when masses M_(1)and M_(2) are suspended respectively from its free end. Then L is equal to
View Solution
