In ABC,P is an interior point such that ∠PAB=100∠PBA=200,∠PCA=300,∠PAC=400 then ABC is (a) isosoceles (b) right angled (c) obtuse angledSolution in Bengali
Step by step video & image solution for In A B C ,P is an interior point such that /_P A B=10^0/_P B A=20^0,/_P C A=30^0,/_P A C=40^0 then A B C is (a) isosoceles (b) right angled (c) obtuse angled by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
The pair of lines joining the origin to the points of intersection of the curves
ax2+2hxy+by2+2gx=0 and
a'x2+2h'xy+b'y2+2g'x=0
will be at right angles to one another , if
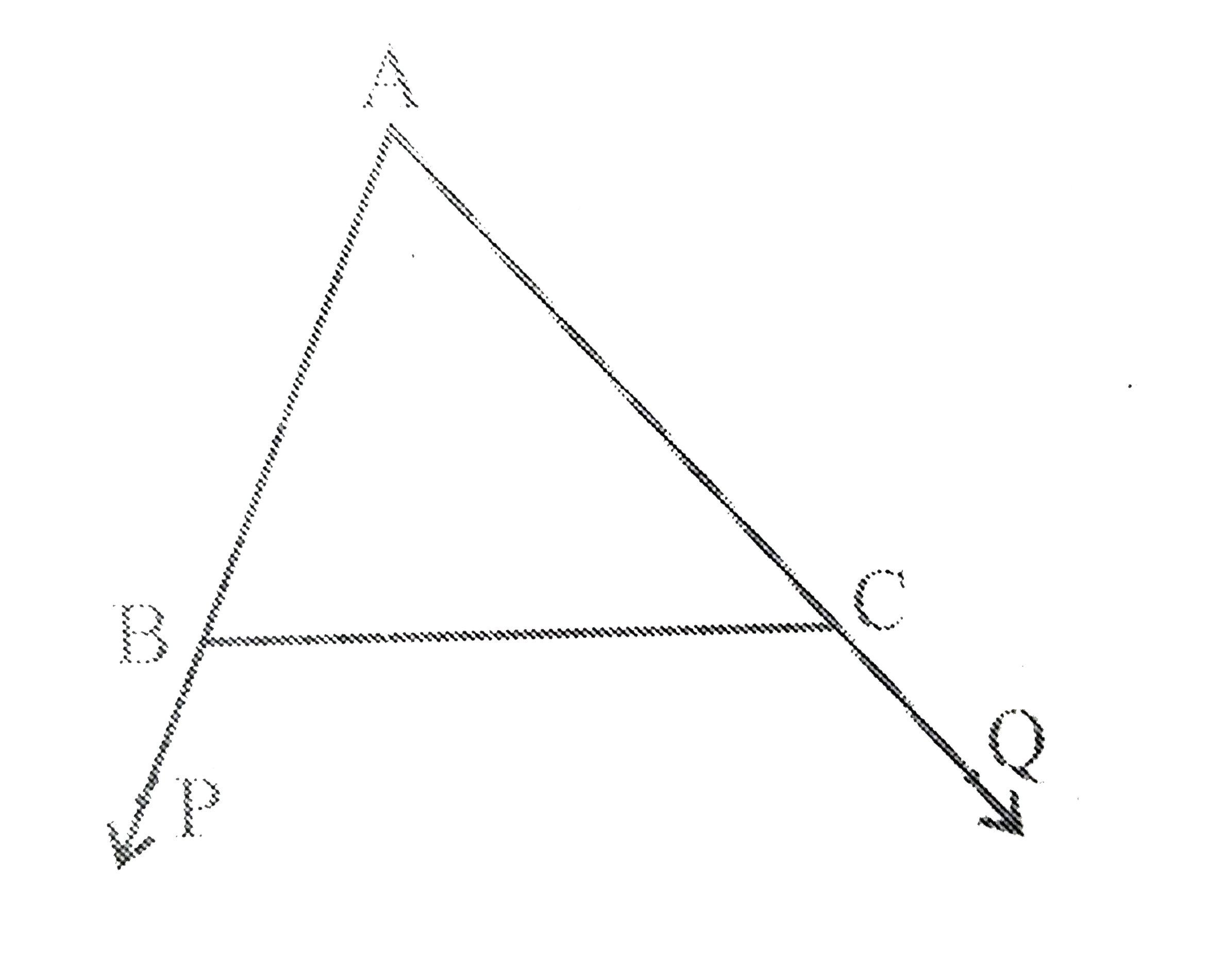
View SolutionIn Fig. 7.48, sides ABandAC of ΔABC are extended to points PandQ respectively. Also, ∠PBC <∠QCB. Show that AC > AB.
View SolutionIf /_A and /_P are acute angle such that tan A=tan P, then show that /_A=/_P
View SolutionIn ABC,P is an interior point such that ∠PAB=100∠PBA=200,∠PCA=300,∠PAC=400 then ABC is (a) isosoceles (b) right angled (c) obtuse angled
View SolutionThe sides AB and AC of a ABC are produced to P and Q respectively. If the bisectors of ∠PBC AND ∠QCB intersect at O, then ∠BOC=900−12∠A GIVEN : A ABC in which sides AB and AC are produced to P and Q respectively. The bisectors of ∠PBC and ∠QCB intersect at O.
View SolutionIn Figure, ABC is an isosceles triangle in which AB=AC.CPparel≤lAB and AP is the bisector of exterior ∠CAD of ABC . Prove that ∠PAC=∠BCA and (ii) ABCP is a parallelogram.
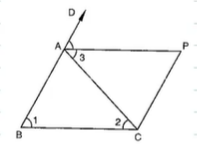
View SolutionIf PQR ≅EFD, then ∠E= ∠P (b) ∠Q (c) ∠R (d) None of these
View SolutionIn figure AB=AC and CP∣∣BA and AP is the bisector of exterior ∠CAD of ABC . Prove that ∠PAC=∠BCA (ii) ABCP is a parallelogram
View SolutionIn Fig., list the points which are: in the interior △P (ii) in the exterior of ∠P and lie on ∠P.
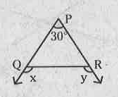
View Solutionపటంలో ∠p=300 అయిన x+y=
View SolutionIn Fig. 7.48, sides ABandAC of ΔABC are extended to points PandQ respectively. Also, ∠PBC <∠QCB. Show that AC > AB.

View SolutionIn ABC,P is an interior point such that ∠PAB=100∠PBA=200,∠PCA=300,∠PAC=400 then ABC is (a) isosoceles (b) right angled (c) obtuse angled
View SolutionThe sides AB and AC of a ABC are produced to P and Q respectively. If the bisectors of ∠PBC AND ∠QCB intersect at O, then ∠boc=900−12∠A GIVEN : A ABC in which sides AB and AC are produced to P and Q respectively. The bisectors of ∠PBC and ∠QCB intersect at O.
View SolutionIn Figure, ABC is an isosceles triangle in which AB=AC.andCP∣∣AB and AP is the bisector of exterior ∠CAD of ABC . Prove that ∠PAC=∠BCA and (ii) ABCP is a parallelogram.
View SolutionGiven ∠POR=3x and ∠QOR=2x+100. If POQ is straight line, then the value of x is 300 (b) 340 (c) 360 (d) none of these
View SolutionIn figure AB=AC and CP∣∣BA and AP is the bisector of exterior ∠CAD of ABC . Prove that
(i) ∠PAC=∠BCA
(ii) ABCP is a parallelogram
View Solution
