In ΔABC, D is the midpoint of side BC, E is mid point of AD, F is midpoint of BE and G is mid-point of FC. What part of the area of ΔABC is the area of ΔEFG?
a)14
b)27
c)38
d)18
To find the area of triangle ΔEFG in relation to the area of triangle ΔABC, we will follow these steps:
Step 1: Understand the Midpoints
In triangle ΔABC:
- D is the midpoint of side BC.
- E is the midpoint of segment AD.
- F is the midpoint of segment BE.
- G is the midpoint of segment FC.
Step 2: Determine the Area Ratios
Since D is the midpoint of BC, the area of triangle ΔABD is half the area of triangle ΔABC. Thus, we can express the area of triangle ΔABD as:
Area of ΔABD=12×Area of ΔABC
Step 3: Area of Triangle ΔABE
Since E is the midpoint of AD, the area of triangle ΔABE is half the area of triangle ΔABD:
Area of ΔABE=12×Area of ΔABD=12×12×Area of ΔABC=14×Area of ΔABC
Step 4: Area of Triangle ΔBEF
Since F is the midpoint of BE, the area of triangle ΔBEF is half the area of triangle ΔABE:
Area of ΔBEF=12×Area of ΔABE=12×14×Area of ΔABC=18×Area of ΔABC
Step 5: Area of Triangle ΔEFG
Since G is the midpoint of FC, the area of triangle ΔEFG is half the area of triangle ΔBEF:
Area of ΔEFG=12×Area of ΔBEF=12×18×Area of ΔABC=116×Area of ΔABC
Step 6: Final Ratio
Now we can find the ratio of the area of triangle ΔEFG to the area of triangle ΔABC:
Ratio=Area of ΔEFGArea of ΔABC=116×Area of ΔABCArea of ΔABC=116
Conclusion
Thus, the area of triangle ΔEFG is 116 of the area of triangle ΔABC.
---
In triangle ΔABC:
- D is the midpoint of side BC.
- E is the midpoint of segment AD.
- F is the midpoint of segment BE.
- G is the midpoint of segment FC.
Since D is the midpoint of BC, the area of triangle ΔABD is half the area of triangle ΔABC. Thus, we can express the area of triangle ΔABD as:
Area of ΔABD=12×Area of ΔABC
Since E is the midpoint of AD, the area of triangle ΔABE is half the area of triangle ΔABD:
Area of ΔABE=12×Area of ΔABD=12×12×Area of ΔABC=14×Area of ΔABC
Since F is the midpoint of BE, the area of triangle ΔBEF is half the area of triangle ΔABE:
Area of ΔBEF=12×Area of ΔABE=12×14×Area of ΔABC=18×Area of ΔABC
Since G is the midpoint of FC, the area of triangle ΔEFG is half the area of triangle ΔBEF:
Area of ΔEFG=12×Area of ΔBEF=12×18×Area of ΔABC=116×Area of ΔABC
Now we can find the ratio of the area of triangle ΔEFG to the area of triangle ΔABC:
Ratio=Area of ΔEFGArea of ΔABC=116×Area of ΔABCArea of ΔABC=116
Thus, the area of triangle ΔEFG is 116 of the area of triangle ΔABC.
Topper's Solved these Questions
Similar Questions
D is the mid-point of side AB of the triangle ABC.E is the mid-point of CD and F is the mid-point of AE. Prove that 8× area of (ΔAFD)= area of ΔABC
View SolutionIn the given figure, D is the mid-point of BC, E is the mid-point of BD and O is the mid-point of AE. Find the ratio of area of ΔBOEandΔABC

View Solution
Knowledge Check
In triangle ABC,D is the midpoint of AB, E is the midpoint of DB and F is the midpoint of BC. If the area of ΔABC is 96, the area of ΔAEF is
A16B24C32D36In a ΔABC,D is the mid - point of BC and E is mid - point of AD, BF passes through E. What is the ratio of AF:FC?
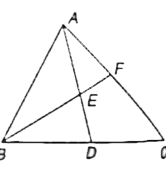 A1:1B1:2C1:3D2:3
A1:1B1:2C1:3D2:3In △ABC, if D is the midpoint of BC and E is the midpoint of AD then ar(△BED) = ?
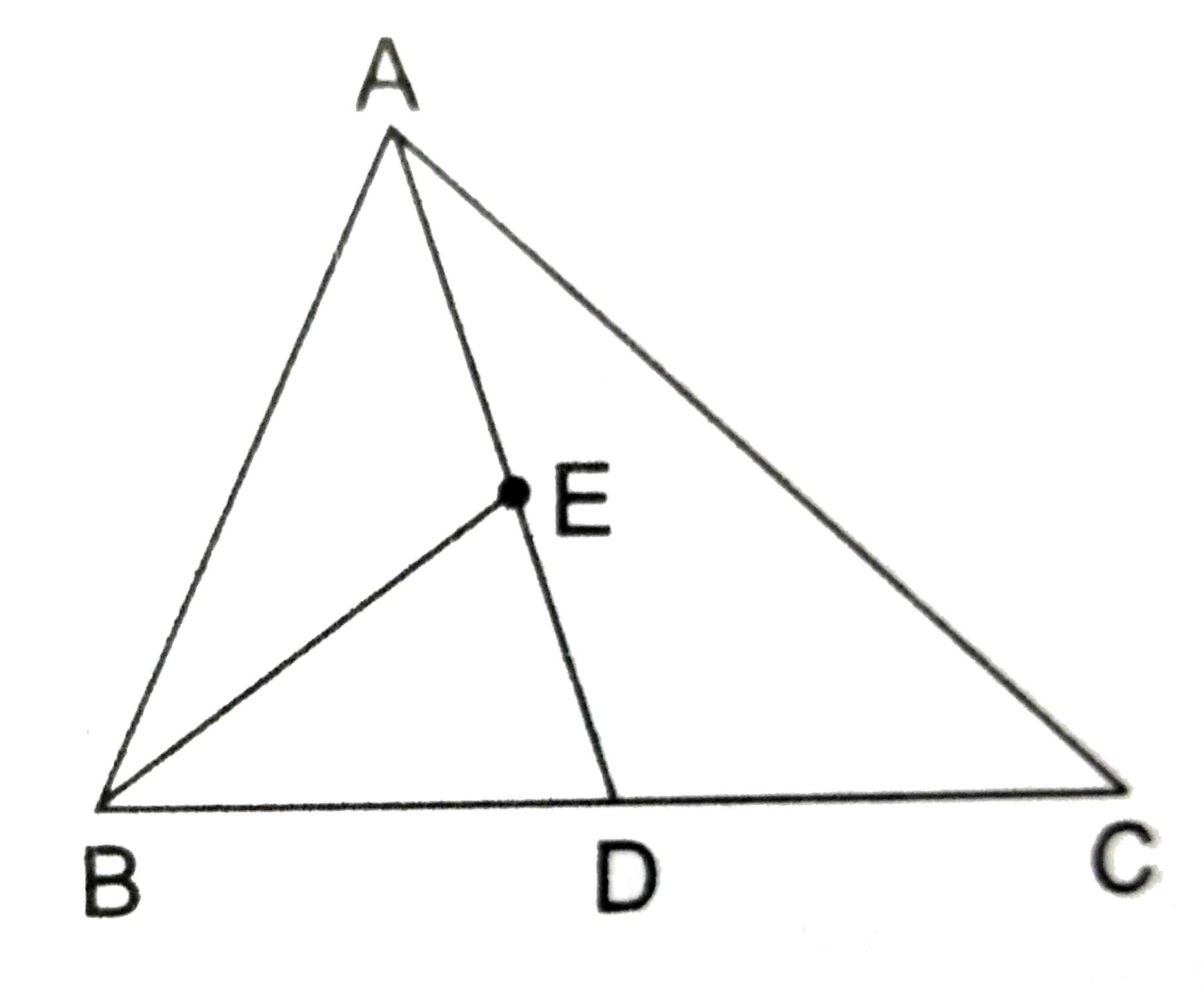 A12ar(△ABC)B13ar(△ABC)C14ar(△ABC)D23ar(△ABC)
A12ar(△ABC)B13ar(△ABC)C14ar(△ABC)D23ar(△ABC)
In ΔABC,E is the midpoint of ¯¯¯¯¯¯BC, while F is midpoint of ¯¯¯¯¯¯AE,and¯¯¯¯¯¯BF meets AC at D, as shownin Fig, If the area of ΔABC=48, find the area of ΔAFD.
View SolutionIn ΔABC, D is the midpoint of side AB. Line DE∣∣ side BC. A−E−C. Prove that pont E is the midpoint of side AC.
View SolutionIn ΔABC,D is the mid-pointpoint of BC and E is mid-point of AD.BE is produced to meet the side AC in F.Then BF is equal to
View SolutionThe area of ΔABC is 44 cm2 . If D is the midpoint of BC and E is the midpoint of AB, then the area ( in cm2) of ΔBDE is :
View SolutionIn ΔABC,∠B=90∘. If P is the mid-point of side AC, then
PA=PB=
View Solution
