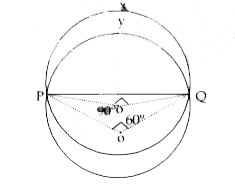
In the figure below, two circular curves create $60^{\circ} and 90^{\circ}$ with their respectively centers. If the length of the bottom curve y is $10 π$ . Find the length of the other curve. Who is equal x?

$\frac{9 π}{\sqrt{2}}$

$15 π$

$9 π$

$\frac{15 π}{\sqrt{2}}$

Video Solution

Answer

Step by step video & image solution for In the figure below, two circular curves create 60^(@) and 90^(@) with their respectively centers. If the length of the bottom curve y is 10pi. Find the length of the other curve. Who is equal x? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.

Updated on:21/07/2023

Class 14 MATHS GEOMETRY

Topper's Solved these Questions

Knowledge Check

The length of subtangent to the curve, $y = e^{x / a}$ is
A2a
Ba unit from origin
C43832
Da/4
The tangent and the normal drawn to the curve $y = x^{2} = x + 4$ at $P (1, 4)$ cut the X=-axis at A and B respectively. If the length of the subtangent drawn to the curve at $P$ is equal to the length of the subnormal, then the area of the triangle $P A B$ (in sq units) is
A4
B32
C8
D16
The equation of the tangent to the curve $y = 4 e^{- \frac{x}{4}}$ at the point where the curve crosses Y-axis is equal to
A $3 x + 4 y = 16$
B $4 x + y = 4$
C $x + y = 4$
D $4 x - 3 y = - 12$

MOTHERS-GEOMETRY -MULTIPLE CHOICE QUESTIONS

Home
Ask Doubt
Profile

In the figure below, two circular curves create 60∘and90∘ with their respectively centers. If the length of the bottom curve y is 10π. Find the length of the other curve. Who is equal x?

Topper's Solved these Questions

Similar Questions

Knowledge Check

The length of subtangent to the curve, y=ex/a is

The tangent and the normal drawn to the curve y=x2=x+4 at P(1,4) cut the X=-axis at A and B respectively. If the length of the subtangent drawn to the curve at P is equal to the length of the subnormal, then the area of the triangle PAB (in sq units) is

The equation of the tangent to the curve y=4e−x4 at the point where the curve crosses Y-axis is equal to

MOTHERS-GEOMETRY -MULTIPLE CHOICE QUESTIONS

In the figure below, two circular curves create $60^{\circ} and 90^{\circ}$ with their respectively centers. If the length of the bottom curve y is $10 π$ . Find the length of the other curve. Who is equal x?

The length of subtangent to the curve, $y = e^{x / a}$ is

The tangent and the normal drawn to the curve $y = x^{2} = x + 4$ at $P (1, 4)$ cut the X=-axis at A and B respectively. If the length of the subtangent drawn to the curve at $P$ is equal to the length of the subnormal, then the area of the triangle $P A B$ (in sq units) is

The equation of the tangent to the curve $y = 4 e^{- \frac{x}{4}}$ at the point where the curve crosses Y-axis is equal to