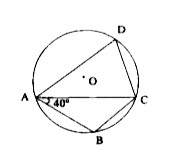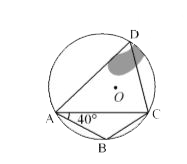
The centre of circumcircle of a quadrilateral ABCD is O. If AB = BC and ∠BAC=40∘ then find angle ∠ADC.
50∘
60∘
70∘
80∘
Step by step video & image solution for The centre of circumcircle of a quadrilateral ABCD is O. If AB = BC and angleBAC=40^(@) then find angle angleADC. by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
In the given figure, ABCD is a cyclic quadrilateral and AB is diameter. ∠ADC=140∘, then find ∠BAC :
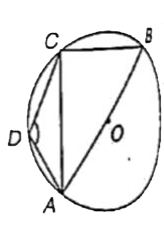 A45∘B40∘C50∘Dnone of the above
A45∘B40∘C50∘Dnone of the above
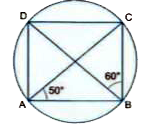
In Fig , ABCD is a cyclic quadrilateral . If ∠BAC=50∘and∠DBC=60∘ then find ∠BCD.
View SolutionABCD is a cyclic quadrilateral. If AD∣∣BCand∠B=70∘, find the other angles of ABCD.
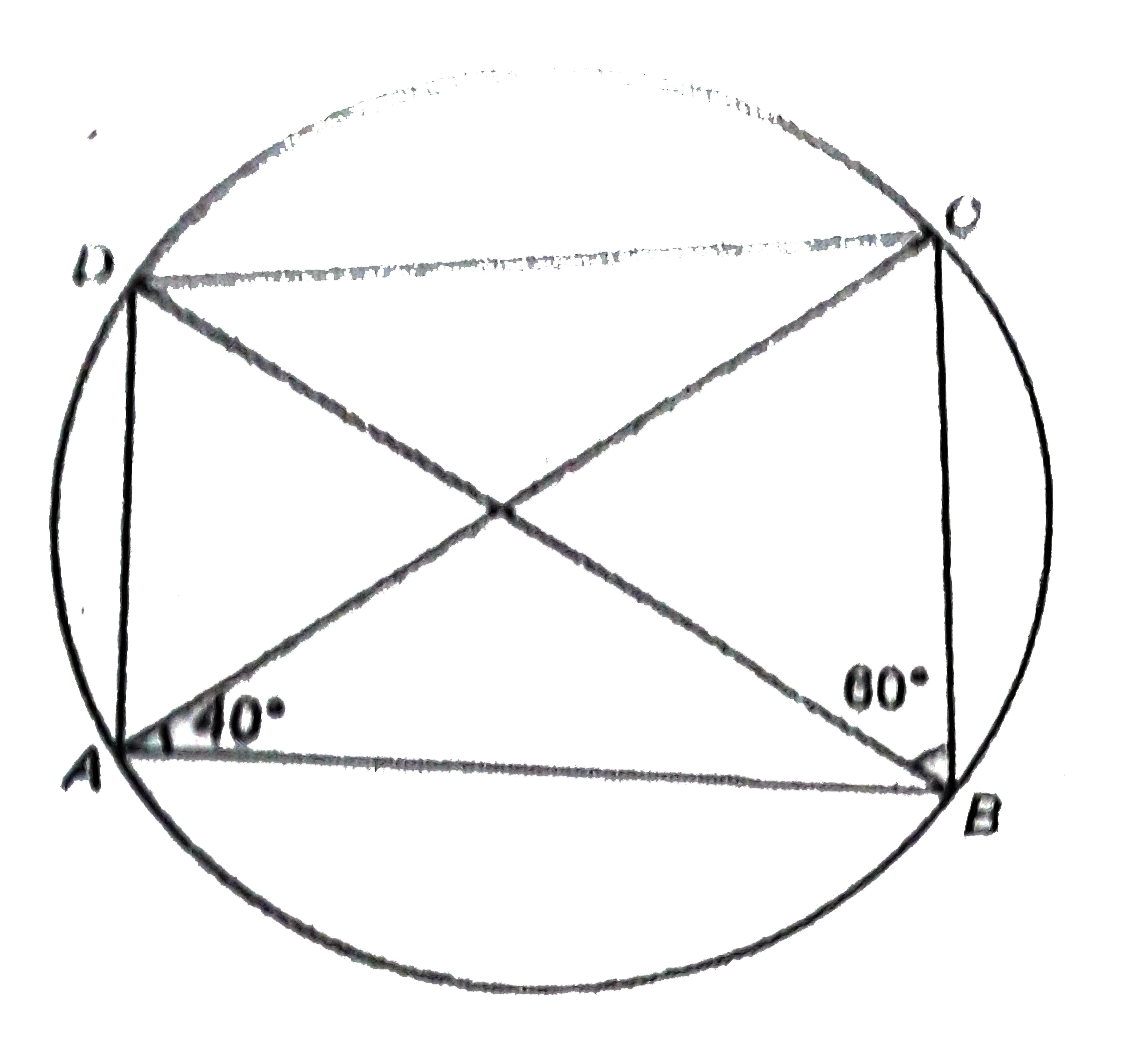
View SolutionIn the adjoining figure, ABCD is a cyclic quadrilateral. If ∠DBC=60∘ and ∠BAC=40∘ , then find the value of ∠BCD .
View SolutionIn cyclic quadrilateral ABCD, AD∣∣BC. Prove that AB = CD.
View SolutionIn a quadrilateral ABCD, if AB = 10 cm, CD = 6 cm and ∠A+∠D=180∘, what type of quadrilateral is ABCD ?
View SolutionAB is the diameter of a circle whose centre is O. DC is a chord of that circle. If DC||AB and ∠BAC=20∘ then ∠ADC=?
View SolutionABCD is a quadrilateral in which AB||DC and AD = BC. Prove that ∠A=∠Band∠C=∠D.
View Solution