In the triangle ABC, MN is parallel to AB. Area of trapezium ABNM is twice the area of triangle CMN. What is ratio of CM : AM?
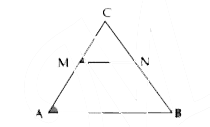
1√3−1
√3+12
√3+12
None of these
Step by step video & image solution for In the triangle ABC, MN is parallel to AB. Area of trapezium ABNM is twice the area of triangle CMN. What is ratio of CM : AM? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Suppose ABC is a triangle with AB of unit length. D and E are the points lying on AB and AC respectively such that BC and DE are parallel. If the area of triangle ABC is twice the area of triangle ADE, then the length of AD is
View SolutionThe centroid of a triangle ABC is G. The area of triangle ABC is 60 cm2. The area of triangle GBC is.
View Solution
Knowledge Check
Let ABC be a triangle and M be a point on side AC closer to vertex C than A. Let N be a point on side AB such that MN is parallel to BC and let P be a point on side BC such that MP is parallel to AB. If the area of the quadrilateral BNMP is equal to 518th of the area of triangle ABC, then the ratio AM/MC equals.
A5B6C185D152ABCD is a trapezium in which AB is parallel to CD and AB = 4 (CD). The diagonals of the trapezium intersects at O. What is the ratio of area of triangle DCO to the area of the triangle ABO?
A1:4B1:2C1:8D1:16
ABCD is a trapezium in which AB is parallel to CD. Let M be the midpoint of CD.
Consider the following statements :
1. Area of triangle ABM - Area of triangle ADM is always equal to area of triangle BCM, if AB = CD
2. Half of area of triangle ABM is equal to one-eighth of area of trapezium ABCD, if AB = CD.
Which of the above statements is/are correct?
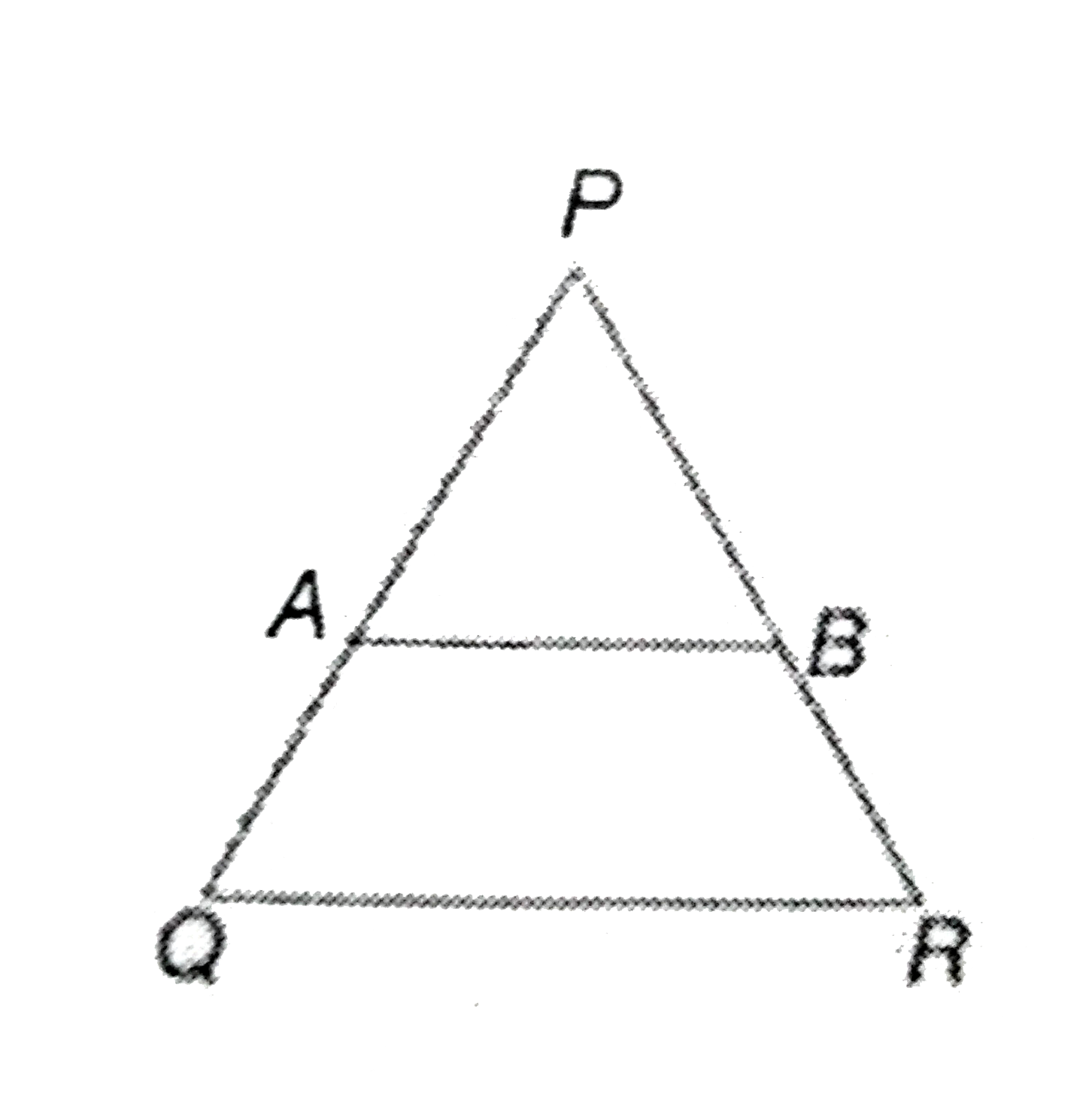
View SolutionIn the triangle PQR, AB is parallel to QR. The ratio of the areas of two similar triangles PAB and PQR is 1:2. Then PQ:AQ=
View SolutionIn the triangle PQR, AB is parallel to QR. The ratio of the areas similar triangles PAB and PQR is 1:2, then PQ:AQ= __________
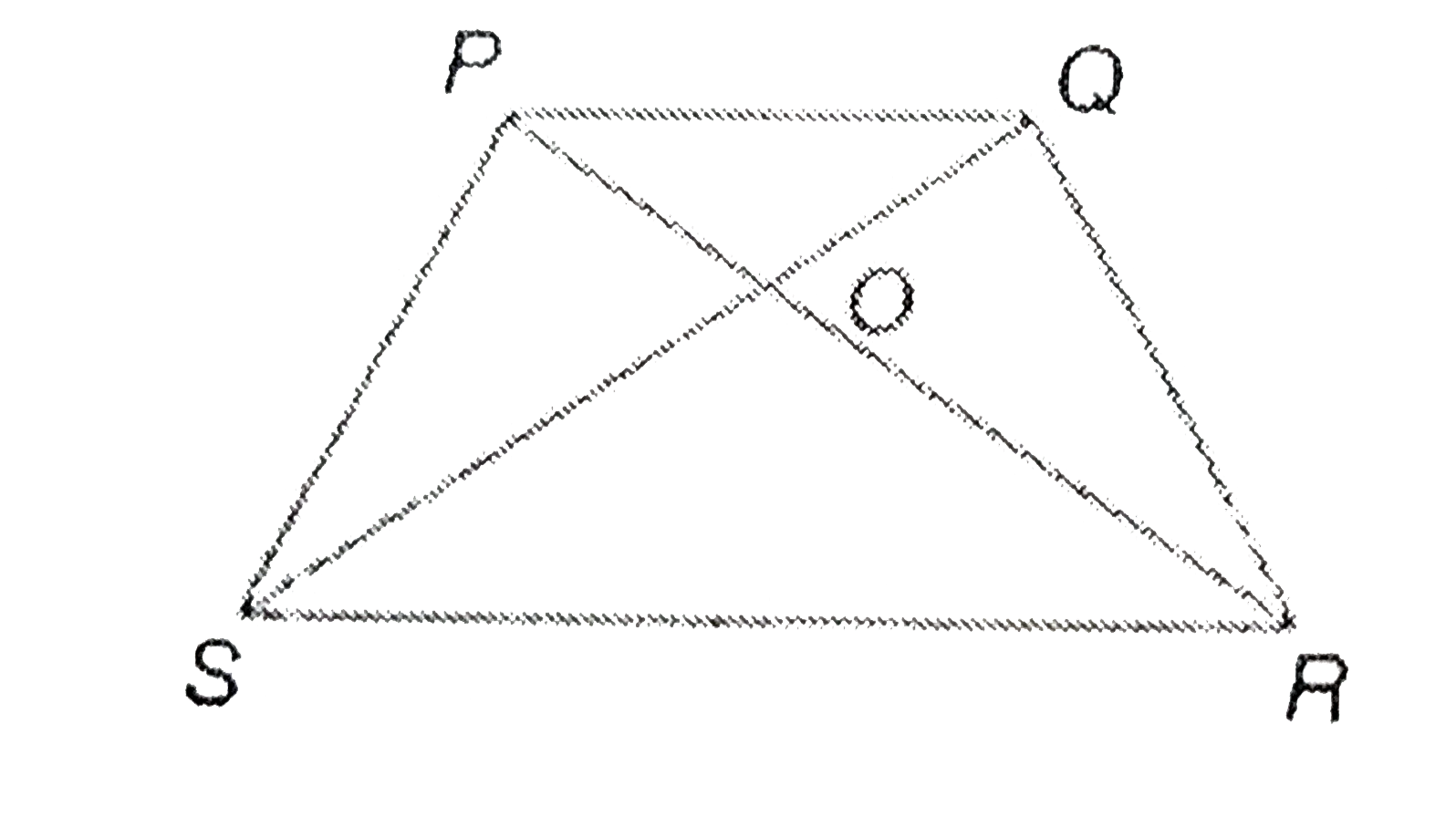
View SolutionThe sides AD, BC of a trapezium. ABCD are parallel and the diagonals AC and BD meet at O. If the area of triangle AOB is 3 cm and the area of triangle BDC is 8 cm2, then what is the area of triangle AOD?
View SolutionAD is a median of triangle ABC. G is a mid point of AD. If area of triangle ABC is 16 sq. cm. Find the area of triangle GDC
View SolutionIn the trapezium PQRS, PQ is parallel to RS and the ratio of the areas of the triangle POQ to triangle ROS is 225:900 . Then SR= _________.
View Solution
