Chords PQ and RS of a circle, when produced, meet at a point O. If PQ = 6cm, OQ = 8cm and OS = 7cm, then length (in cm) of the chord RS is
16
9
10
12
To find the length of the chord RS in the given circle, we can use the Power of a Point theorem, which states that if two chords intersect at a point outside the circle, the product of the lengths of the segments of one chord is equal to the product of the lengths of the segments of the other chord.
1. Identify the lengths given in the problem:
- Length of chord PQ = 6 cm
- Length of segment OQ = 8 cm
- Length of segment OS = 7 cm
2. Determine the length of OP:
- Since PQ = 6 cm, we can find OP by recognizing that OQ + OP = PQ.
- Therefore, OP = PQ - OQ = 6 cm - 8 cm = -2 cm (which doesn't make sense in this context).
- Instead, we should recognize that OQ is part of the entire length of the chord PQ. Thus, we need to calculate OP as follows:
- Let OP = x. Then OQ + OP = PQ gives us 8 cm + x = 6 cm, which leads us to x = -2 cm. This indicates that we need to consider the lengths correctly.
3. Calculate the total length of OP:
- Since OQ is 8 cm and the total length of PQ is 6 cm, we need to find the total length of OP.
- OP = PQ + OQ = 6 cm + 8 cm = 14 cm.
4. Determine the length of OR:
- Since OS = 7 cm and we need to find OR, we can use the Power of a Point theorem:
- According to the theorem, OQ * OP = OS * OR.
- We have OQ = 8 cm, OP = 14 cm, and OS = 7 cm.
- Therefore, we can express this as:
8×14=7×OR
5. Solve for OR:
- Calculate the left side:
8×14=112
- Now, we set up the equation:
112=7×OR
- To find OR, divide both sides by 7:
OR=1127=16cm
6. Calculate the length of chord RS:
- Now that we have both OS and OR, we can find the length of chord RS.
- The length of RS is given by:
RS=OS+OR=7cm+16cm=23cm
Final Answer:
The length of the chord RS is 23 cm.
- Length of chord PQ = 6 cm
- Length of segment OQ = 8 cm
- Length of segment OS = 7 cm
- Since PQ = 6 cm, we can find OP by recognizing that OQ + OP = PQ.
- Therefore, OP = PQ - OQ = 6 cm - 8 cm = -2 cm (which doesn't make sense in this context).
- Instead, we should recognize that OQ is part of the entire length of the chord PQ. Thus, we need to calculate OP as follows:
- Let OP = x. Then OQ + OP = PQ gives us 8 cm + x = 6 cm, which leads us to x = -2 cm. This indicates that we need to consider the lengths correctly.
- Since OQ is 8 cm and the total length of PQ is 6 cm, we need to find the total length of OP.
- OP = PQ + OQ = 6 cm + 8 cm = 14 cm.
- Since OS = 7 cm and we need to find OR, we can use the Power of a Point theorem:
- According to the theorem, OQ * OP = OS * OR.
- We have OQ = 8 cm, OP = 14 cm, and OS = 7 cm.
- Therefore, we can express this as:
8×14=7×OR
- Calculate the left side:
8×14=112
- Now, we set up the equation:
112=7×OR
- To find OR, divide both sides by 7:
OR=1127=16cm
- Now that we have both OS and OR, we can find the length of chord RS.
- The length of RS is given by:
RS=OS+OR=7cm+16cm=23cm
The length of the chord RS is 23 cm.
Topper's Solved these Questions
Similar Questions
In a circle, chords PQ and TS are produced to meet at R. If RQ= 14.4 cm, PQ= 11.2 cm and SR=12.8 cm, then the length of chord TS is:
एक वृत्त में, जीवाओं PQ तथा TS को बढ़ाया जाता है जो R पर मिलती हैं | यदि RQ = 14.4 सेमी, PQ =11.2 सेमी तथा SR = 12.8 सेमी है, तो जीवा TS की लंबाई कितनी है ?
View SolutionIn a circle, AB and DC are two chords. When AB and DC are produced, they meet at P. If PC = 5.6 cm, PB = 6.3 cm and AB = 7.7 cm, then the length of CD is:
View Solution
Knowledge Check
Chords PQ and RS of a circle, when produced, meet at a point O. If PQ = 6cm, OQ = 8cm and OS = 7cm, then length (in cm) of the chord RS is
A10B12C16D9In a circle, chords PQ and TS are produced to meet at R. If RQ= 14.4 cm, PQ= 11.2 cm and SR=12.8 cm, then the length of chord TS is:
एक वृत्त में, जीवाओं PQ तथा TS को बढ़ाया जाता है जो R पर मिलती हैं | यदि RQ = 14.4 सेमी, PQ =11.2 सेमी तथा SR = 12.8 सेमी है, तो जीवा TS की लंबाई कितनी है ?A18 cmB16 cmC14.2 cmD112.4 cmIn a circle chords PO and TS are produced to meet at R. If RO 14.4 cm, PO = 11.2 cm and SR = 12.8 cm then the length of chord TS is :
A18cmB14.2 cmC12.4 cmD16 cm
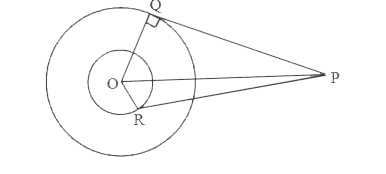
In the given figure, PQ is tangent to outer circle and PR is tangent to inner circle. If PQ = 4cm, OQ = 3 cm and OR = 2 cm then find the length of PR.
View SolutionTwo chords ABandPQ of a circle intersect at D inside a circle. If AD = 4 cm., DB = 6 cm., QD = 3 cm., the length of PQ is equal to
View SolutionTwo chords AB and PQ of a circle mutually intersect at an outside point D. If AD=12 cm, AB=8 cm, DQ=6 cm then find PQandPD.
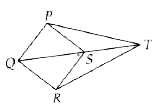
View SolutionIn the figure, PQ = QR = RS = SP = SQ = 6 cm and PT = RT = 14 cm. The length of ST (in cm) is
View SolutionPQ and RS are two parallel chords of a circle whose centre is O and radius is 10 cm. If PQ = 16 cm and RS = 12 cm, find the distance between PQ and RS, if they lie :
(i) On the same side of the centre O.
(ii) On opposite side of the centre O.
View Solution
