Question

State Gauss' law in electrostatics. Using this law, derive an expression for the electric field due to an infinitely long straight charged wire at a point distant r from it. Plot a graph showing the variation of electric field with r.

Explanation

Electric field due to infinitely long, straight uniformly charged wire : Consider a long thin charged wire having positive charge uniformly distributed over it. Let `lambda` be the linear charge density.
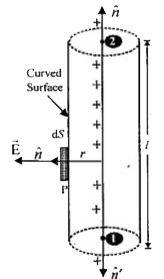
Let P be a point at a perpendicular distance r from the line, electric field intensity is to be determined.
Let us choose a cylindrical gaussian surface of radius r and lenght l coaxial with the wire as shown in Fig. The circular ends (1) and (2) i.e., plane surfaces of cylinder do not contribute to the electric flux because `vec(E ) and hat(n)` are at right angle to each other. `hat(n)` gives the direction of area vector `(vec(dS))andvec(dS)=hat(n)dS`
i.e., `vec(E)hat(n)dS=E(1)cos90^(@)dS=0`
`[therefore cos 90^(@) = 0]`
The only contribution to the flux is due to curved surface of the cylinder.
By symmetry cosisderation.the magnitude of electric intensity will be same at all the points of the curved surface to the cylinder and the direction is radialy outward.
Now, cosider a small area element dS around P. The flux through this area is given by
`d phi_(E ) = vec(E ). vec(dS) = vec(E ). hat(n).dS " " [dvec(S) = hat(n)dS]`
= `E (1) cos 0^(@) dS " " [therefore vec(E ) || hat(n), therefore theta = 0^(@)]`
and total electric flux through whole of the curved surface is
`phi_(E) = oint E dS = E oint ds`
or `phi_(E) = E (2 pi rl)`
`therefore oint E ds = 2 pi rl` = total area of curved surface of cylinder
From Gauss.s theorem, `phi_(E) = (q)/(epsilon_(0))`
Comparing eqns, (i) and (ii), we get
`E (2 pi r l) = (q)/(epsilon_(0)) or E = (q)/(2 pi r epsilon_(0)l)`
But `(q)/(l) = lambda` is the linear charge density.
`therefore E = (lambda)/(2 pi epsilon_(0)r)`
It is the expression for electric field intensity due to long, straight charged, wire. When wire is placed in a medium of dielectric constant K, then above eqn. becomes.
`E = (lambda)/(2 pi k epsilon_(0)r)`
Direction of the electric field is radially outward along the radius of cylinder on either side of long straight charged wire.