A bullet ofmass m is fired with a velocity 10m/s at angle θ with horizontal. At the hightest point of its trajectory, it collsides head-on with a bob of mass 3m suspended by a massless string of length 2/5m and gets embedded in the bob. After th collision the string moves through an angle of 60∘.
The vertical coordinate of the initial positon of the bob w.r.t. the point of firing of the bullet is
94m
95m
245m
None of these
The correct Answer is:(B)
To solve the problem step by step, we will follow these main points:
1. Understanding the Problem: A bullet of mass m is fired at an angle θ with a velocity of 10m/s. At the highest point of its trajectory, it collides with a bob of mass 3m suspended by a massless string of length 25m. After the collision, the string moves through an angle of 60∘. We need to find the vertical coordinate of the initial position of the bob with respect to the point of firing of the bullet.
2. Finding the Horizontal Velocity of the Bullet: At the highest point of the trajectory, the vertical component of the bullet's velocity is zero. The horizontal component of the velocity remains 10cosθ.
3. Conservation of Momentum: Before the collision, the momentum of the bullet is:
pinitial=m⋅(10cosθ)+0=m⋅(10cosθ)
After the collision, the bullet and bob move together with a combined mass of 4m and a common velocity V:
pfinal=4m⋅V
By conservation of momentum:
m⋅(10cosθ)=4m⋅V
Simplifying gives:
V=10cosθ4=5cosθ2
4. Using Conservation of Energy: The kinetic energy just after the collision is converted into potential energy as the bob rises. The change in kinetic energy is:
ΔKE=12⋅4m⋅V2−0
The potential energy gained is:
PE=4mgh
Setting these equal gives:
12⋅4m⋅V2=4mgh
Simplifying:
12V2=gh
5. Finding Height h: We can express h in terms of V:
h=V22g
Substituting V=5cosθ2:
h=(5cosθ2)22g=25cos2θ8g
6. Using the Geometry of the Problem: The bob moves through an angle of 60∘. The vertical component of the string length gives:
h=l(1−cos60∘)=25(1−12)=25⋅12=15m
7. Finding the Maximum Height of the Bullet: The maximum height H attained by the bullet is given by:
H=u2sin2θ2g
Here, u=10m/s and g=10m/s2:
H=102sin2θ2⋅10=100sin2θ20=5sin2θ
8. Finding sin2θ: From the triangle formed by sinθ and cosθ:
sin2θ+cos2θ=1
If cosθ=45, then:
sin2θ=1−(45)2=1−1625=925
9. Calculating H:
H=5⋅925=4525=1.8m
Thus, the vertical coordinate of the initial position of the bob with respect to the point of firing of the bullet is 1.8m.
pinitial=m⋅(10cosθ)+0=m⋅(10cosθ)
After the collision, the bullet and bob move together with a combined mass of 4m and a common velocity V:
pfinal=4m⋅V
By conservation of momentum:
m⋅(10cosθ)=4m⋅V
Simplifying gives:
V=10cosθ4=5cosθ2
ΔKE=12⋅4m⋅V2−0
The potential energy gained is:
PE=4mgh
Setting these equal gives:
12⋅4m⋅V2=4mgh
Simplifying:
12V2=gh
h=V22g
Substituting V=5cosθ2:
h=(5cosθ2)22g=25cos2θ8g
h=l(1−cos60∘)=25(1−12)=25⋅12=15m
H=u2sin2θ2g
Here, u=10m/s and g=10m/s2:
H=102sin2θ2⋅10=100sin2θ20=5sin2θ
sin2θ+cos2θ=1
If cosθ=45, then:
sin2θ=1−(45)2=1−1625=925
H=5⋅925=4525=1.8m
Topper's Solved these Questions
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-I
40 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-II
43 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3 Videos
Similar Questions
A bullet ofmass m is fired with a velocity 10m/s at angle θ with horizontal. At the hightest point of its trajectory, it collsides head-on with a bob of mass 3m suspended by a massless string of length 2/5m and gets embedded in the bob. After th collision the string moves through an angle of 60∘.
The horizontal coordinate of the intital position of the bob w.r.t. the point of firing of the bullet is n
View SolutionA bullet ofmass m is fired with a velocity 10m/s at angle θ with horizontal. At the hightest point of its trajectory, it collsides head-on with a bob of mass 3m suspended by a massless string of length 2/5m and gets embedded in the bob. After th collision the string moves through an angle of 60∘.
The angle θ is
View SolutionA bob of mass m=50g is suspended form the ceiling of a trolley by a light inextensible string. If the trolley accelerates horizontally, the string makes an angle 37∘ with the vertical. Find the acceleration of the trolley.

View SolutionA bob of mass m suspended by a light inextensible string of length 'l' from a fixed point. The bob is given a speed of √6gl. Find the tension in the string when string deflects through an angle 120∘ from the vertical.
View SolutionA bob of mass 1 kg is suspended from an inextensible string of length 1 m. When the string makes an angle 60∘ with vertical, speed of the bob is 4 m/s
Upto what maximum height will the bob rise with respect to the bottommost point ?
View SolutionA mass of M kg is suspended by a weightless string. The horizontal force required to displace it until string makes an angle of 45∘ with the initial vertical direction is:
View SolutionA bob of mass 1 kg is suspended from an inextensible string of length 1 m. When the string makes an angle 60∘ with vertical, speed of the bob is 4 m/s
Net acceleration of the bob at this instant is
View SolutionA pendulum bob has a speed of 3ms−1 at its lowest position. The pendulum is 0.5 m long. The speed of the bob, when string makes an angle of 60∘ to the vertical is (take, g=10ms−1)
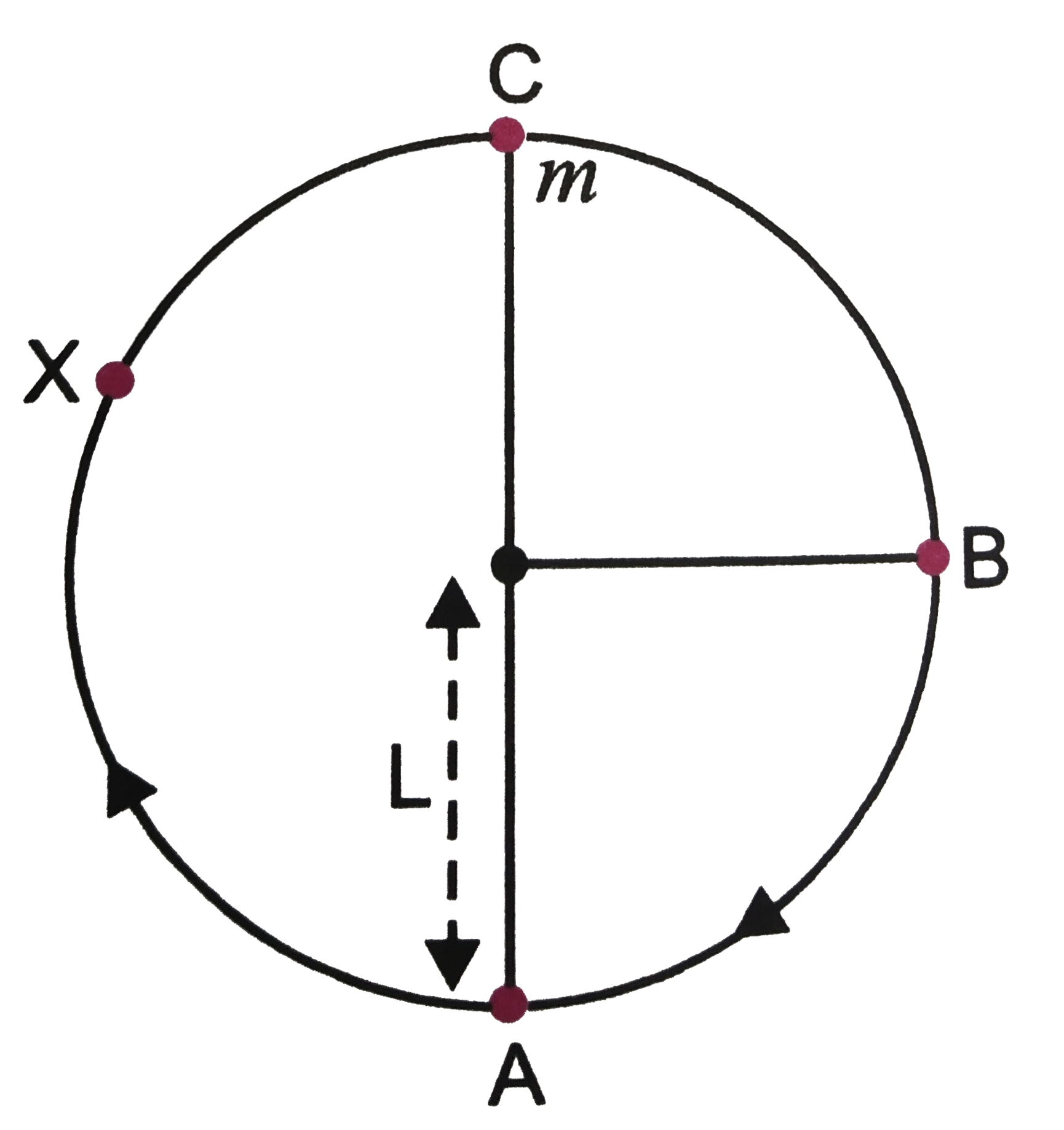
View SolutionA bob of mass m suspended by a light string of length L is whirled into a vertical circle as shown in figure . What will be the trajectory of the particle if the string is cut at
(a) Point B ? (b) Point C? (c) Point X?
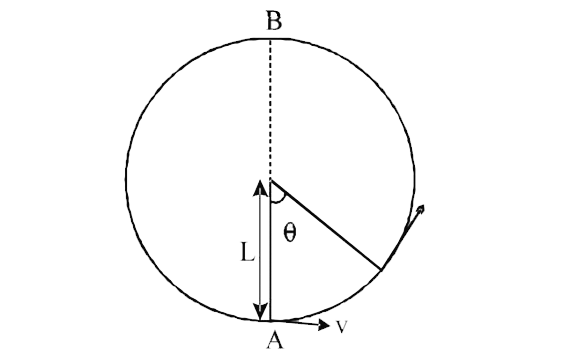
View SolutionA bob of mass M is suspended by a massless string of length L. The horizontal velocity v at position A is just sufficient to make it reach the point B. The angle θ at which the speed of the bob is half of that at A, satisfies
View Solution
