M is the mid-point of a line segment AB. AXB and MYB are equilateral triangles on opposite sides of AB.XYcuts AB at Z. Find m if AZ=mZB.
Step by step video & image solution for M is the mid-point of a line segment AB. AXB and MYB are equilateral triangles on opposite sides of AB. XY cuts AB at Z. Find m if AZ=m ZB . by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - part-2 Mathematic
18 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - part-2 Mathematics
48 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - PHYSICS
4 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - CHEMISTRY SECTION-II
3 Videos
Similar Questions
M is the mid-point of a line segment AB, AXB and MYB are equilateral triangles on opposite sides of AB, XY cuts AB at Z. Prove that: AZ= 2ZB.
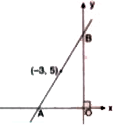
View SolutionThe mid - point of line segment AB (shown in the diagram) is (-3, 5), Find the co-ordinates of A and B.
 .
.
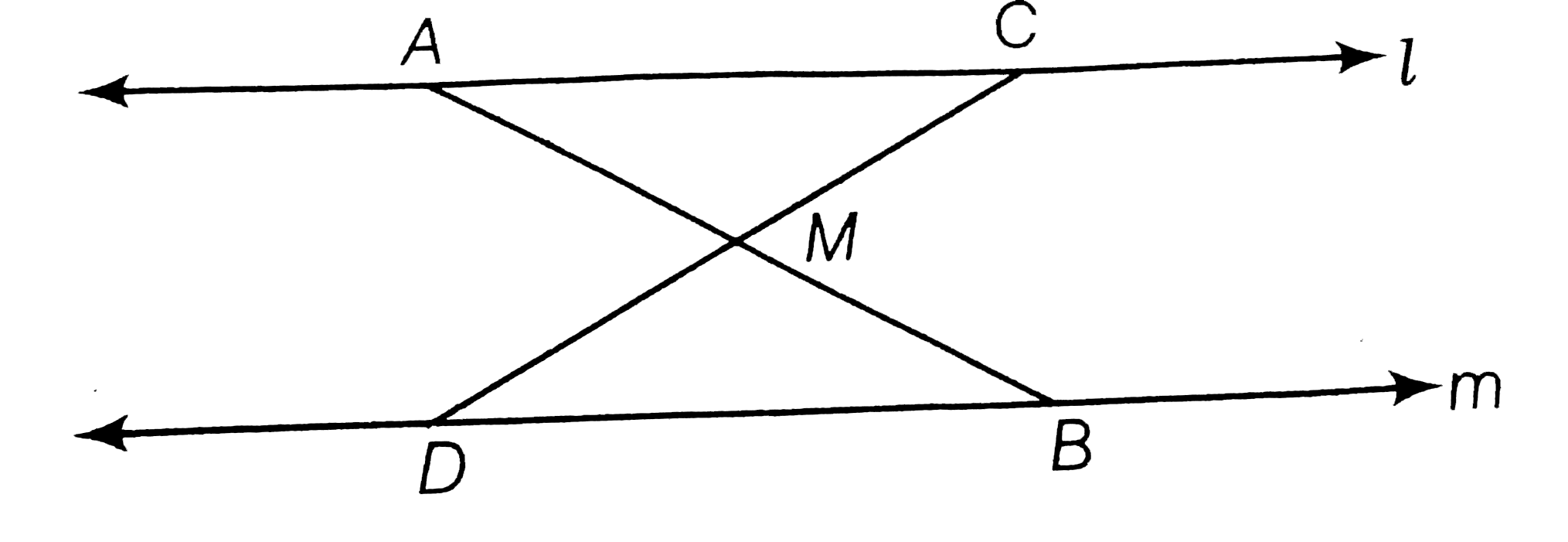
View SolutionIn the given figure l||m and M is the mid-point of a line segment AB .Show that M is also the mid- point of any line segment CD, having its end points on l and m, respectively.
View SolutionP (-3, 2) is the mid point of line segment AB as shown in the given figure. Find the P (-3,2) co-ordinates of points A and B.
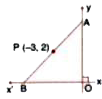
View SolutionD and F are the mid-points of sides AB and AC of a triangle ABC. A line through F and parallel to AB meets BC at point E.
Find AB, if EF=4.8cm.
View SolutionAD and BC are equal perpendiculars to a line segment AB. If AD and BC are on different sides of AB prove that CD bisects AB.
View SolutionDraw a line segment AB of length 7 cm. Using ruler and compasses, find a point P on AB such
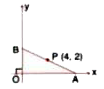
View SolutionIn the given figure, P (4, 2) is mid-point of line segment AB. Find the co-ordinates of A and B.
View SolutionIn Figure, lIIm and M is the mid-point of the line segment AB. Prove that M is also the mid-point of any line segment CD having its end-points on l and m respectively.
View SolutionIn Figure, l∣∣m and M is the mid-point of the line segment AB . Prove that M is also the mid-pooint of any line segment CD having its end-points on l and m respectively.
View Solution
