A flag is mounted on a car moving due North with velocity of . Strong winds are blowing due East with velocity of . The flag will point in direction
East
North-East
South-East
South-West
The correct Answer is:C
To solve the problem of determining the direction in which the flag will point, we can follow these steps:
Step 1: Understand the velocities involved
- The car is moving due North with a velocity of 20 km/hr.
- The wind is blowing due East with a velocity of 20 km/hr.
Step 2: Represent the velocities as vectors
- The velocity of the car can be represented as a vector pointing upwards (North) on a coordinate system:
→Vcar=(0,20) km/hr
- The velocity of the wind can be represented as a vector pointing to the right (East):
→Vwind=(20,0) km/hr
Step 3: Calculate the resultant velocity vector
- The resultant velocity vector →Vresultant can be found by adding the two vectors:
→Vresultant=→Vcar+→Vwind=(0+20,20+0)=(20,20) km/hr
Step 4: Determine the direction of the resultant vector
- The resultant vector (20,20) indicates that the flag will point in a direction that is at an angle to both the North and East directions.
- To find the angle θ with respect to the North direction, we can use the tangent function:
tan(θ)=OppositeAdjacent=2020=1
- Thus, θ=45∘.
Step 5: Identify the direction of the flag
- Since the angle is 45∘ from the North towards the East, the flag will point in the North-East direction.
Conclusion
- The flag will point in the North-East direction.
- The car is moving due North with a velocity of 20 km/hr.
- The wind is blowing due East with a velocity of 20 km/hr.
- The velocity of the car can be represented as a vector pointing upwards (North) on a coordinate system:
→Vcar=(0,20) km/hr
- The velocity of the wind can be represented as a vector pointing to the right (East):
→Vwind=(20,0) km/hr
- The resultant velocity vector →Vresultant can be found by adding the two vectors:
→Vresultant=→Vcar+→Vwind=(0+20,20+0)=(20,20) km/hr
- The resultant vector (20,20) indicates that the flag will point in a direction that is at an angle to both the North and East directions.
- To find the angle θ with respect to the North direction, we can use the tangent function:
tan(θ)=OppositeAdjacent=2020=1
- Thus, θ=45∘.
- Since the angle is 45∘ from the North towards the East, the flag will point in the North-East direction.
- The flag will point in the North-East direction.
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)46videos
KINEMATICS-2D
- BOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (S-2)15videos
KINEMATICS-2D
- BOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 49videos
GRAVITATION
- BOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - SOLVED EXAMPLE28videos
MOTION IN A PALNE
Similar Questions
A car A moves along north with velocity 30 km/h and another car B moves along east with velocity 40 km/h. The relative velocity of A with respect to B is
View SolutionA man going east in a car with a velocity of 20 km/hr, a train appears to him to move towards north with a velocity of 20√3 km/hr. What is the actual velocity and direction of motion of train?
View SolutionThe vector difference between the absolute velocities of two bodies is called their relative velocity. The concept of relative velocity enables us to treat one body as being at rest while the other is in motion with the relative velocity. This jormalism greatly simplifies many problems. If →vAis the absolute velocity of a body A and →–––(B)that of another body B then the relative velocity of A in relation to B is →–––(AD)=→VA−→vB and →vBA=→vB-→vA.
The principle follows here is that the relative velocity of two bodies remains unchaged if the same additional velocity is imparted to both the bodies. A simple way of carrying out this operation is to be represent the velocities in magnitude and direction from a common point. Then the line joining the tips of the vectors represents the relative velocity
Q A train A moves to the east with velocity of 40 km/hr and a train B moves due north with velocity of 30 km/hr. The relative velocity of A w.rt. B is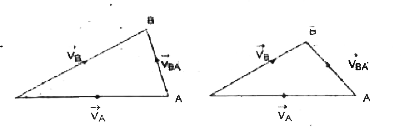 View Solution
View SolutionA man moves on a cycle with a velocity of 4 km/hr. The rain appears to fall on him with a velocity of 3 km/hr vertically. The actual velocity of the rain is
View SolutionA boat is moving towards east velocity 4 m/s w.r.t still water and river is flowing towards north with velocity 2 m/s and the wind blowing towards north with velocity 6 m/s. the direction of the flag blown over by the wind hpisted on the boat is
View SolutionA bota has a velocity 4m/s towards east with respect to river is flowing north with velocity 2m/s Wind is blowing towards north with velocity 6m/s. The direction of the flag blown over by the wind hoisted on the boat is :
View SolutionOut of the two cars A and B car A is moving towards east with velocity of 10 m/s whereas B is moving towards north with a velocity 20 m/s, then velocity of A w.r.t B is ( nearly )
View SolutionAn observer is travelling in east with a velocity of 2 m/s and observes that wind is blowing in north with a velocity of 2m/s. If observer doubles his velocity then find out the velocity of wind appears to observer ?
View SolutionWhat is the velocity of the highest point of a rotating wheel of a car moving with a velocity of 20 m/s ?
View SolutionAn observer is travelling in west with velocity of 5 km/h and observes that wind is blowing in north with the velocity of 5√3 km/h. Find out the actual velocity of wind.
View Solution

