An observer on ground sees a boat cross a river of width 800 m perpendicular to its stream in 200 seconds. He also finds a man on a raft floating at speed of 3 m/s with river. The distance travelled by boat as seen by man on the raft in crossing the river is -
800 m
1000 m
1200 m
1600 m
The correct Answer is:B
To solve the problem step-by-step, we will analyze the motion of the boat and the raft separately and then combine the information to find the distance traveled by the boat as seen by the man on the raft.
Step 1: Determine the speed of the boat with respect to the ground.
The width of the river is given as 800 meters, and the time taken to cross it is 200 seconds. We can calculate the speed of the boat using the formula:
Speed=DistanceTime
Substituting the values:
Speed of the boat=800m200s=4m/s
Step 2: Determine the speed of the raft.
The speed of the raft is given as 3 m/s. This speed is in the direction of the river's current.
Step 3: Calculate the velocity of the boat with respect to the raft.
To find the velocity of the boat as seen by the man on the raft, we need to consider the velocities as vectors. The boat is moving perpendicular to the current (in the y-direction), while the raft is moving with the current (in the x-direction).
- Velocity of the boat with respect to the ground: →vb=4^jm/s
- Velocity of the raft with respect to the ground: →vr=3^im/s
The velocity of the boat with respect to the raft is given by:
→vbr=→vb−→vr=4^j−3^i
Step 4: Calculate the magnitude of the velocity of the boat with respect to the raft.
To find the magnitude of the velocity vector →vbr:
|→vbr|=√(−3)2+(4)2=√9+16=√25=5m/s
Step 5: Calculate the distance traveled by the boat as seen by the man on the raft.
Now, we can find the distance traveled by the boat as observed by the man on the raft. The time taken to cross the river is still 200 seconds.
Using the formula for distance:
Distance=Speed×Time
Substituting the values:
Distance=5m/s×200s=1000m
Final Answer:
The distance traveled by the boat as seen by the man on the raft is 1000 meters.
---
The width of the river is given as 800 meters, and the time taken to cross it is 200 seconds. We can calculate the speed of the boat using the formula:
The speed of the raft is given as 3 m/s. This speed is in the direction of the river's current.
To find the velocity of the boat as seen by the man on the raft, we need to consider the velocities as vectors. The boat is moving perpendicular to the current (in the y-direction), while the raft is moving with the current (in the x-direction).
- Velocity of the raft with respect to the ground: →vr=3^im/s
To find the magnitude of the velocity vector →vbr:
Now, we can find the distance traveled by the boat as observed by the man on the raft. The time taken to cross the river is still 200 seconds.
The distance traveled by the boat as seen by the man on the raft is 1000 meters.
Topper's Solved these Questions
Similar Questions
A man crosses the river perpendicular to river in time t seconds and travels an equal distance down the stream in T seconds. The ratio of man's speed in still water to the river water will be:
View SolutionA man swims across a river with speed of Vm perpendicular to the flow direction of river. If the water flows with a speed Vw with what resullant velocity does the man cross the river ?
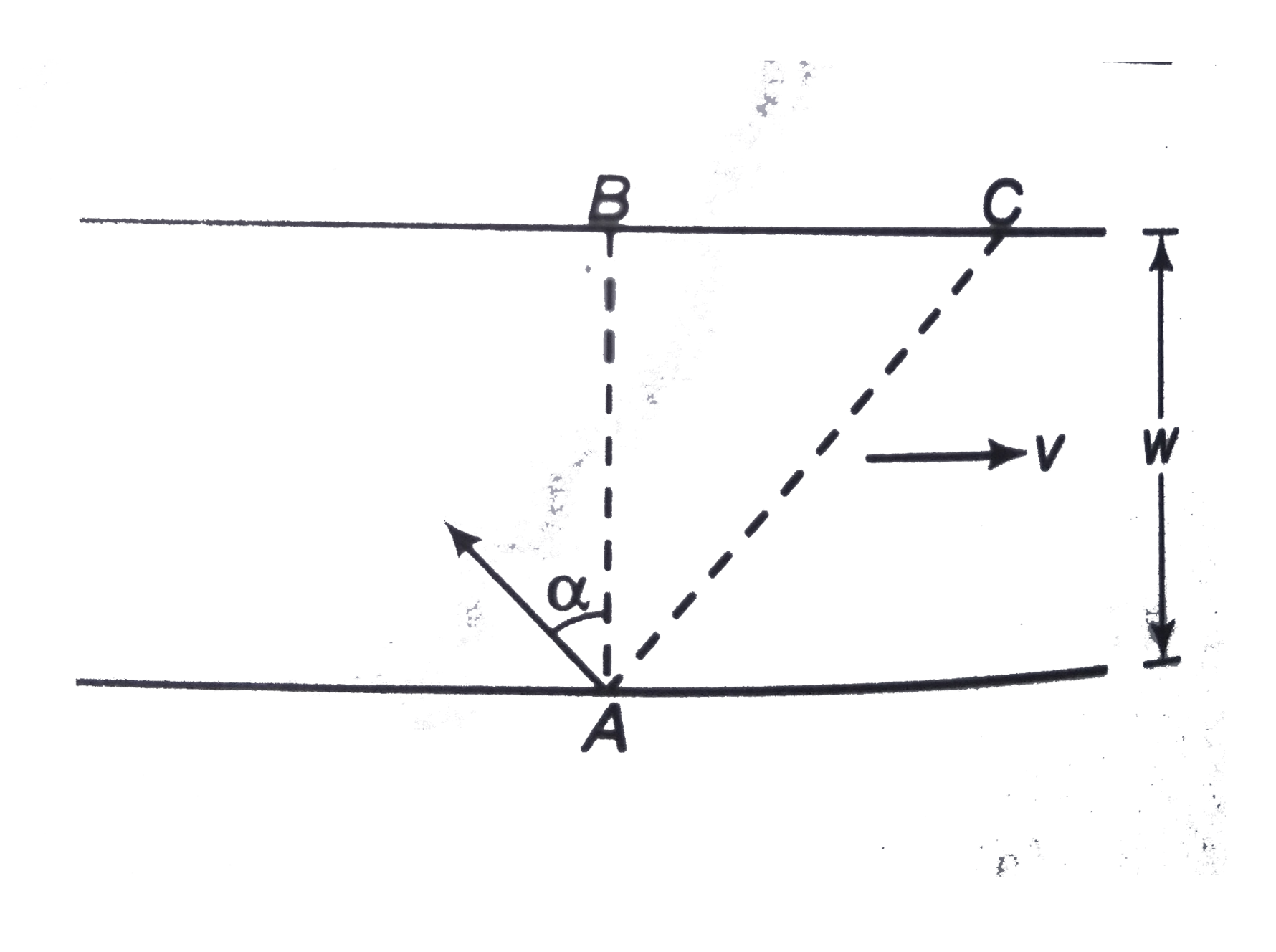
View SolutionA man in a boat crosses a river from point A. If he rows perpendicular to the banks he reaches point C (BC=120m) in 10 min. If the man heads at a certain angle α to the straight line AB (AB is perpendicular to the banks) against the current he reaches point B in 12.5 min. Find the width of the river w, the rowing velocity u, the speed of the river current v and the angle α. Assume the velocity of the boat relative to water to be constant and the same magnitude in both cases.
View Solution
Two ports, A and B, on a north-south line ar separated by a river of width D. The river flows east with speed u. A boat crosses the river starting from port A. The speed of the boat relative to the river is v. Assume v=2u
Q. Suppose the boat wants to cross the river from A to the other side in the shortest possible time. Then what should be the direction of the velocity of boat relative to river?
View SolutionA boat can be rowed at 5 m/s on still water. It is used to cross a 200m wide river from the south bank to the north bank. The river current has uniform velocity of 3 m/s due east.
(a) In which direction must it be steered to cross the river perpendicular to current ?
(b) How long will it take to cross the river in a direction perpendicular to the river flow ?
(c) In which direction must the boat be steered to cross the river in minimum time ? How far will it drift ?
View SolutionThe current velocity of river grows in proportion to the distance from its bank and reaches the maximum value v0 in the middle. Near the banks the velocity is zero. A boat is moving along the river in such a manner that the boatman rows his boat always perpendicular to the current. The speed of the boat in still water is u. Find the distance through which the boat crossing the river will be carried away by the current, if the width of the river is c. Also determine the trajectory of the boat.
View SolutionA man can swim in still water at a speed of 6 kmph and he has to cross the river and reach just opposite point on the other bank. If the river is flowing at a speed of 3 kmph, and the width of the river is 2 km, the time taken to cross the river is (in hours)
View Solutionman wants to swim across a river of which 200 m along the shortest path . If the speed of river stream is 3kmh−1 and speed of swimmer in still water is 5kmh−1 , then the time of crossing the river is
View SolutionA boat which has a speed of 6km/h in still water crosses a river of width 1 km along the shortest possible path in 20 min. the velocity of the river water in km/h is
View SolutionA man wishes to cross a river of width 120 m by a motorboat. His rowing speed in still water is 3m/s and his maximum walking speed is 1m/s. The river flows with velocity of 4m/s.
(a) Find the path which he should take to get to the point directly opposite to his starting point in the shortest time.
(b) Also, find the time which he takes to reach his destination.
View Solution
