A massive ball moving with speed v collieds with a tiny ball which is intially at rest having a mass very much smaller than the mass of the first ball. The collision is elastic, then immediately after the impact, the second ball will move with a speed approximately equal to :-
v
2v
v/2
∞
The correct Answer is:B
To solve the problem of a massive ball colliding elastically with a tiny ball initially at rest, we will use the principles of conservation of momentum and the properties of elastic collisions. Here’s a step-by-step solution:
Step 1: Define the masses and velocities
Let:
- M = mass of the massive ball (initially moving)
- m = mass of the tiny ball (initially at rest)
- v = initial speed of the massive ball
- V1 = final speed of the massive ball after the collision
- V2 = final speed of the tiny ball after the collision
Step 2: Write the conservation of momentum equation
Since momentum is conserved in the collision, we can write:
M⋅v+m⋅0=M⋅V1+m⋅V2
This simplifies to:
M⋅v=M⋅V1+m⋅V2(1)
Step 3: Write the equation for elastic collision
For an elastic collision, the relative velocity of separation is equal to the relative velocity of approach. This can be expressed as:
V2−V1=v(2)
Step 4: Substitute V1 from equation (2) into equation (1)
From equation (2), we can express V1 in terms of V2:
V1=V2−v
Substituting this into equation (1):
M⋅v=M⋅(V2−v)+m⋅V2
Expanding this gives:
M⋅v=M⋅V2−M⋅v+m⋅V2
Rearranging the terms:
M⋅v+M⋅v=(M+m)⋅V2
This simplifies to:
2M⋅v=(M+m)⋅V2(3)
Step 5: Solve for V2
Now we can solve for V2:
V2=2M⋅vM+m
Step 6: Simplify V2 using the condition m≪M
Since m is very much smaller than M (i.e., m≪M), we can neglect m in the denominator:
V2≈2M⋅vM=2v
Conclusion
Thus, the speed of the tiny ball after the collision is approximately:
V2≈2v
Final Answer
The tiny ball will move with a speed approximately equal to 2v.
---
Let:
- M = mass of the massive ball (initially moving)
- m = mass of the tiny ball (initially at rest)
- v = initial speed of the massive ball
- V1 = final speed of the massive ball after the collision
- V2 = final speed of the tiny ball after the collision
Since momentum is conserved in the collision, we can write:
M⋅v+m⋅0=M⋅V1+m⋅V2
This simplifies to:
M⋅v=M⋅V1+m⋅V2(1)
For an elastic collision, the relative velocity of separation is equal to the relative velocity of approach. This can be expressed as:
V2−V1=v(2)
From equation (2), we can express V1 in terms of V2:
V1=V2−v
Substituting this into equation (1):
M⋅v=M⋅(V2−v)+m⋅V2
Expanding this gives:
M⋅v=M⋅V2−M⋅v+m⋅V2
Rearranging the terms:
M⋅v+M⋅v=(M+m)⋅V2
This simplifies to:
2M⋅v=(M+m)⋅V2(3)
Now we can solve for V2:
V2=2M⋅vM+m
Since m is very much smaller than M (i.e., m≪M), we can neglect m in the denominator:
V2≈2M⋅vM=2v
Thus, the speed of the tiny ball after the collision is approximately:
V2≈2v
The tiny ball will move with a speed approximately equal to 2v.
Topper's Solved these Questions
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (ROTATIONAL MOTION)
72 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (GRAVITATION)
25 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (CIRCULAR MOTION)
17 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
A heavy ball moving with speed v collides with a tiny ball. The collision is elastic, then immediately after the impact, the second ball will move with a speed approximately equal to
View SolutionA ball of mass M moving with speed v collides perfectly inelastically with another ball of mass m at rest. The magnitude of impulse imparted to the first ball is
View SolutionA ball of mass M moving with speed v collides perfectly inelastically with another ball of mass m at rest. The magnitude of impulse imparted to the first ball is
View SolutionA ball of mass M moving with speed v collides perfectly inelastically with another ball of mass m at rest. The magnitude of impulse imparted to the first ball is
View SolutionA small ball of mass m moving with speed v collides elastically with a simple pendulum with bob of mass m at rest. The maximum height attained by the bob after collision is
View SolutionA ball of mass m moving at a speed v collides with another ball of mass 3m at rest. The lighter block comes to rest after collisoin. The coefficient of restitution is
View SolutionA particle of mass m moving with speed V collides elastically with another particle of mass 2m. Find speed of smaller mass after head on collision
View SolutionA ball is moving with velocity 2m/s towards a heavy wall moving towards the ball with speed 1m/s as shown in figure. Assuming collision to be elastic, find the velocity of ball immediately after the collision.
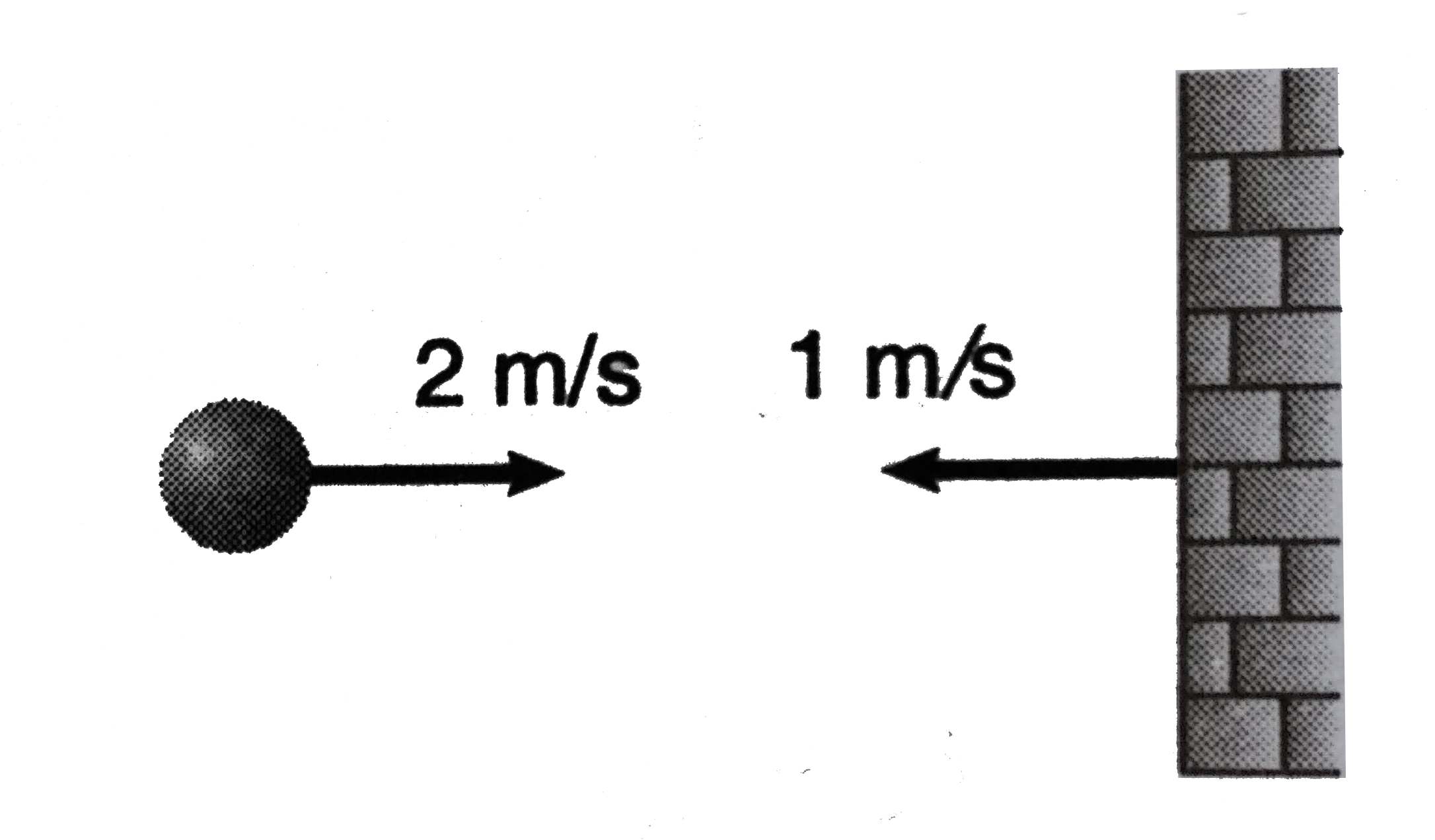
View SolutionA ball is moving with velocity 2m/s towards a heavy wall moving towards the ball with speed 1m/s as shown in figure. Assuming collision to be elastic, find the velocity of ball immediately after the collision.

View SolutionA ball of mass m moving at a speed v makes a head-on collision with an identical ball at rest. The kinetic energy of the balls after the collision is three fourths of the original. Find the coefficient of restitution.
View Solution
