A tiny sphere of mass m and density x is dropped in a jar of glycerine of density y. When the sphere aquires terminal velocity, the magnitude of the viscous force acting on it is :
mgxy
mgyx
mg[1−yx]
mg[1+yx]
The correct Answer is:C
To solve the problem, we need to analyze the forces acting on the tiny sphere when it reaches terminal velocity in the glycerine.
1. Identify the Forces Acting on the Sphere:
- When the sphere is dropped into the glycerine, three forces act on it:
- The gravitational force (weight) acting downwards: Fg=mg
- The buoyant force acting upwards: Fb
- The viscous force acting upwards: Fv
2. Understand Terminal Velocity:
- At terminal velocity, the net force acting on the sphere is zero. This means that the downward force (weight) is balanced by the sum of the upward forces (buoyant force and viscous force):
Fg=Fb+Fv
3. Calculate the Buoyant Force:
- The buoyant force can be calculated using Archimedes' principle:
Fb=density of liquid×volume of sphere×g
- The volume of the sphere can be expressed in terms of its mass and density:
Volume=mx
- Therefore, the buoyant force becomes:
Fb=y×mx×g=mygx
4. Set Up the Equation for Terminal Velocity:
- Substituting the expressions for the forces into the equilibrium equation:
mg=Fb+Fv
- Rearranging gives:
Fv=mg−Fb
- Substituting for Fb:
Fv=mg−mygx
5. Factor Out Common Terms:
- Taking mg as a common factor:
Fv=mg(1−yx)
6. Final Expression for the Viscous Force:
- Thus, the magnitude of the viscous force acting on the sphere when it reaches terminal velocity is:
Fv=mg(1−yx)
Conclusion:
The magnitude of the viscous force acting on the sphere at terminal velocity is mg(1−yx).
- When the sphere is dropped into the glycerine, three forces act on it:
- The gravitational force (weight) acting downwards: Fg=mg
- The buoyant force acting upwards: Fb
- The viscous force acting upwards: Fv
- At terminal velocity, the net force acting on the sphere is zero. This means that the downward force (weight) is balanced by the sum of the upward forces (buoyant force and viscous force):
Fg=Fb+Fv
- The buoyant force can be calculated using Archimedes' principle:
Fb=density of liquid×volume of sphere×g
- The volume of the sphere can be expressed in terms of its mass and density:
Volume=mx
- Therefore, the buoyant force becomes:
Fb=y×mx×g=mygx
- Substituting the expressions for the forces into the equilibrium equation:
mg=Fb+Fv
- Rearranging gives:
Fv=mg−Fb
- Substituting for Fb:
Fv=mg−mygx
- Taking mg as a common factor:
Fv=mg(1−yx)
- Thus, the magnitude of the viscous force acting on the sphere when it reaches terminal velocity is:
Fv=mg(1−yx)
The magnitude of the viscous force acting on the sphere at terminal velocity is mg(1−yx).
Topper's Solved these Questions
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Properties of Matter & Fluid Mechanics)(Hydrostatics)
20 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Properties of Matter & Fluid Mechanics)(Surface Tension)
26 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Properties of Matter & Fluid Mechanics)(Elasticity)
20 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
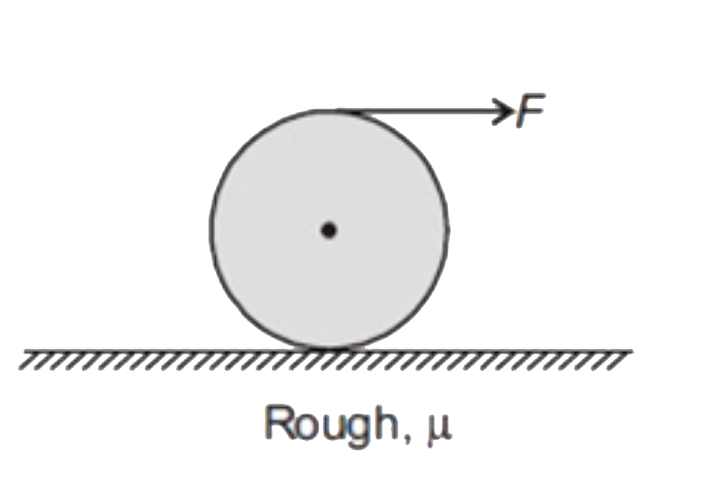
A solid sphere of mass M and radius R is pulled by a force F as shown in figure . If the sphere does not slip over the surface , then frictional force acting on the sphere is
View SolutionThe velocity of small ball of mass M and density (d1= when dropped a container filled with glycerine becomes constant after some time. If the density glycerine is d2, the viscous force acting on ball is
View SolutionA thin hollow sphere of mass m is completely filled with a liquid of mass m. When the sphere rolls with a velocity v kinetic energy of the system is (neglect friction)
View SolutionA small metal ball of diameter 4mm and density 10.5g/cm3 in dropped in glycerine of density 1.5g/cm3. The ball attains a terminal velocity of 8/cms−1. The coefficient of viscosity of glycerine is
View SolutionA small metal ball of diameter 4mm and density 10.5g/cm3 in dropped in glycerine of density 1.5g/cm3. The ball attains a terminal velocity of 8cms−1. The coefficient of viscosity of glycerine is
View SolutionStatement-1 : When two speres of same size but of different densities are dropped in a fluid, sphere of greater density will have greater terminal velocity.
And
Statement-2 : Greater the density and viscosity of the fluid, lesser will be the terminal velocity.
View SolutionA solid sphere of mass M and radius R is in pure rolling as shown in figure. The angular momentum of the sphere about the origin is (linear velocity of centre of mass is v0, in positive x-direction)
View Solution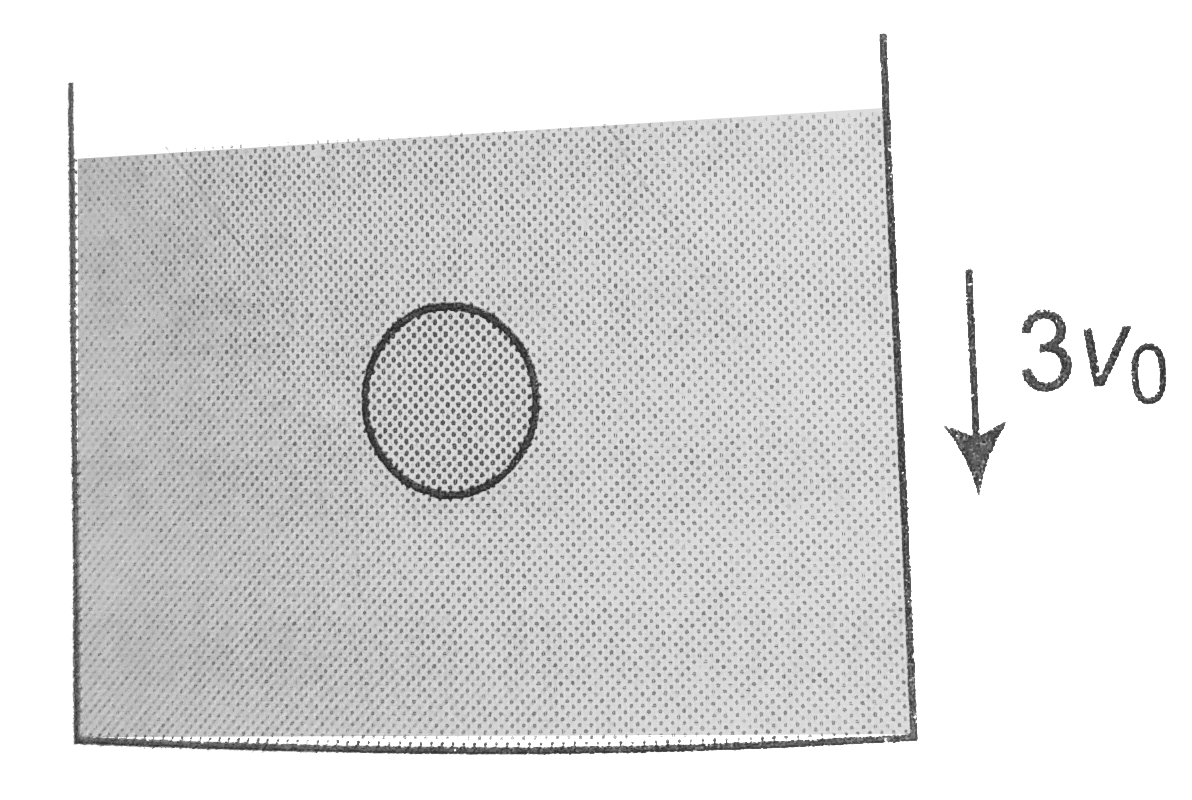
A container filled with viscous liquid is moving vertically downwards with constant speed 3v0. At the instant shown, a sphere of radius r is moving vertically downwards (in liquid) has speed v0. The coefficient of viscosity is η. There is no relative motion between the liquid and the container. Then at the shoen instant, The magnitude of viscous force acting on sphere is
View SolutionTwo copper balls of radius r and 2r are released at rest in a long tube filled with liquid of uniform viscosity. After some time when both the spheres acquire critical velocity (terminal velocity) then ratio of viscous force on the balls is :
View SolutionA sphere of radius R is gently dropped into liquid of viscosity η in a vertical uniform tube. It attains a terminal velocity v. Another sphere of radius 2R when dropped into the same liquid, will attain its teriminal velocity.
View Solution
