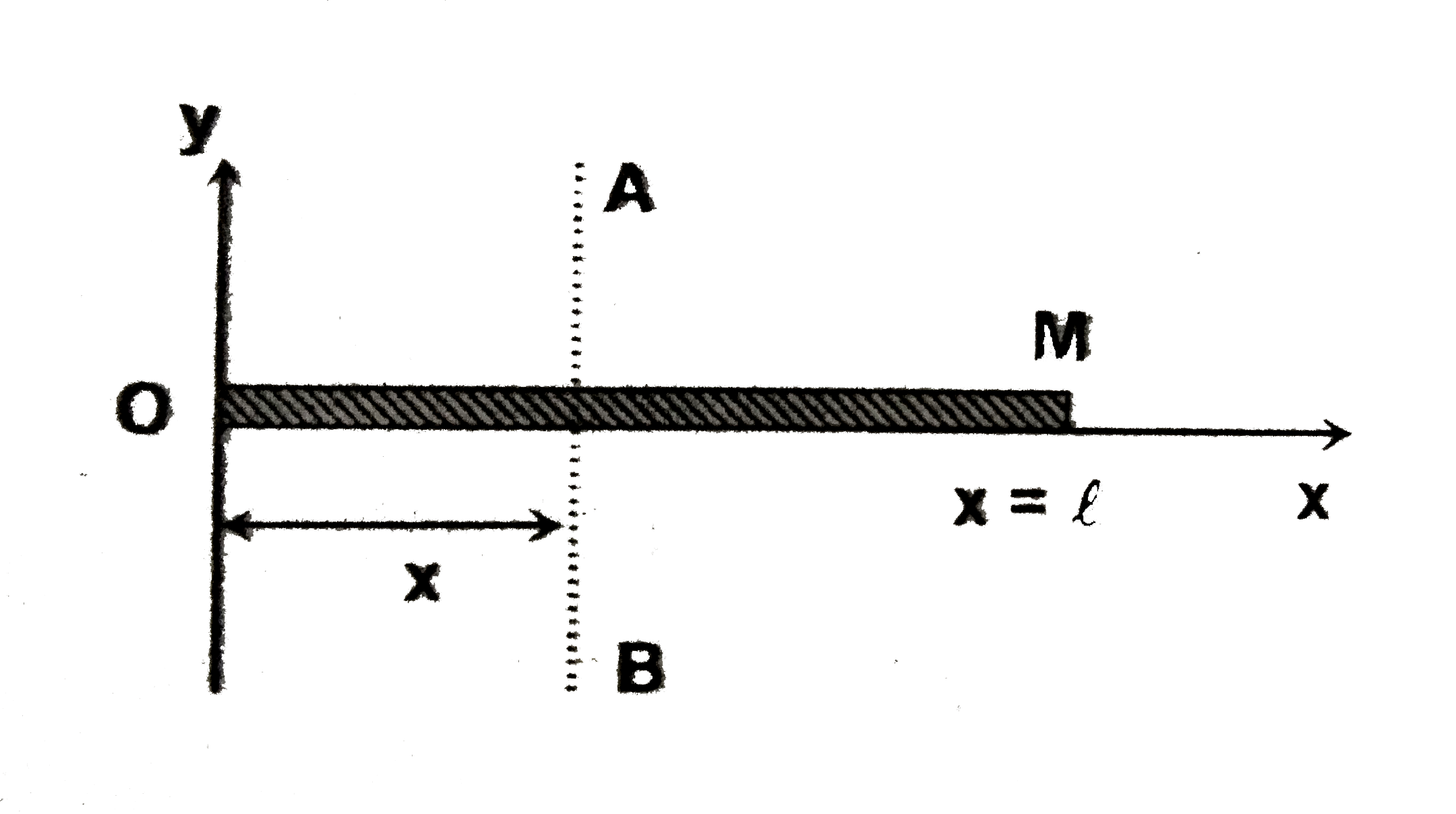
A non uniform rod OM (of length l m) is kept along x-axis and rotating about an axis AB, which is perpendicular to rod as shown in the figure. The rod has linear mass densty that varies with the distance x from left end of the rod according to λ=λ0(x3L3)
Where unit of λ0 is kg/m. What is the value of x so that moment of inertia of rod about axis AB (IAB) is minimum?
7l15
2l5
8l15
4l5
The correct Answer is:D
First method:
IAB=∫y2dm=λ0l3∫l−x−x(y+x)3y2dy
∫(y+x)3y2dy=y2(y+x)44−12y(y+x)4dy
=y2(y+x)24−y10(y+x)5+110(y+x)5dy
=y2(y+x)24−y(x+y)510+(y+x)260
∫(l−x)−x0y2(y+x)3dx=(L−x)2(l)44−(l−x)l510+l660
IAB=λ0[(l−x)24l−110(l−x)l2+160l3]=λ0l[(l−x)24l−110(l−x)+160l2]
dlABdx=0⇒−(l−x)2+l10=0⇒5l−5x=l ⇒5x=4l ⇒ x=4l5
Second method:
As we know that moment of inertial is minimum among all set of parallel axis, if axis is passing through centre of mass, so x must be distance of centre of mass.
XCM=1M∫L0xdm
M=∫dm=λ0l3l∫0x3dx=λ0l4 and l∫0xdx=λ0l3l∫0x4dx=λ0l25
Putting these values in equation (i) we have xCM=4l5
Third method:
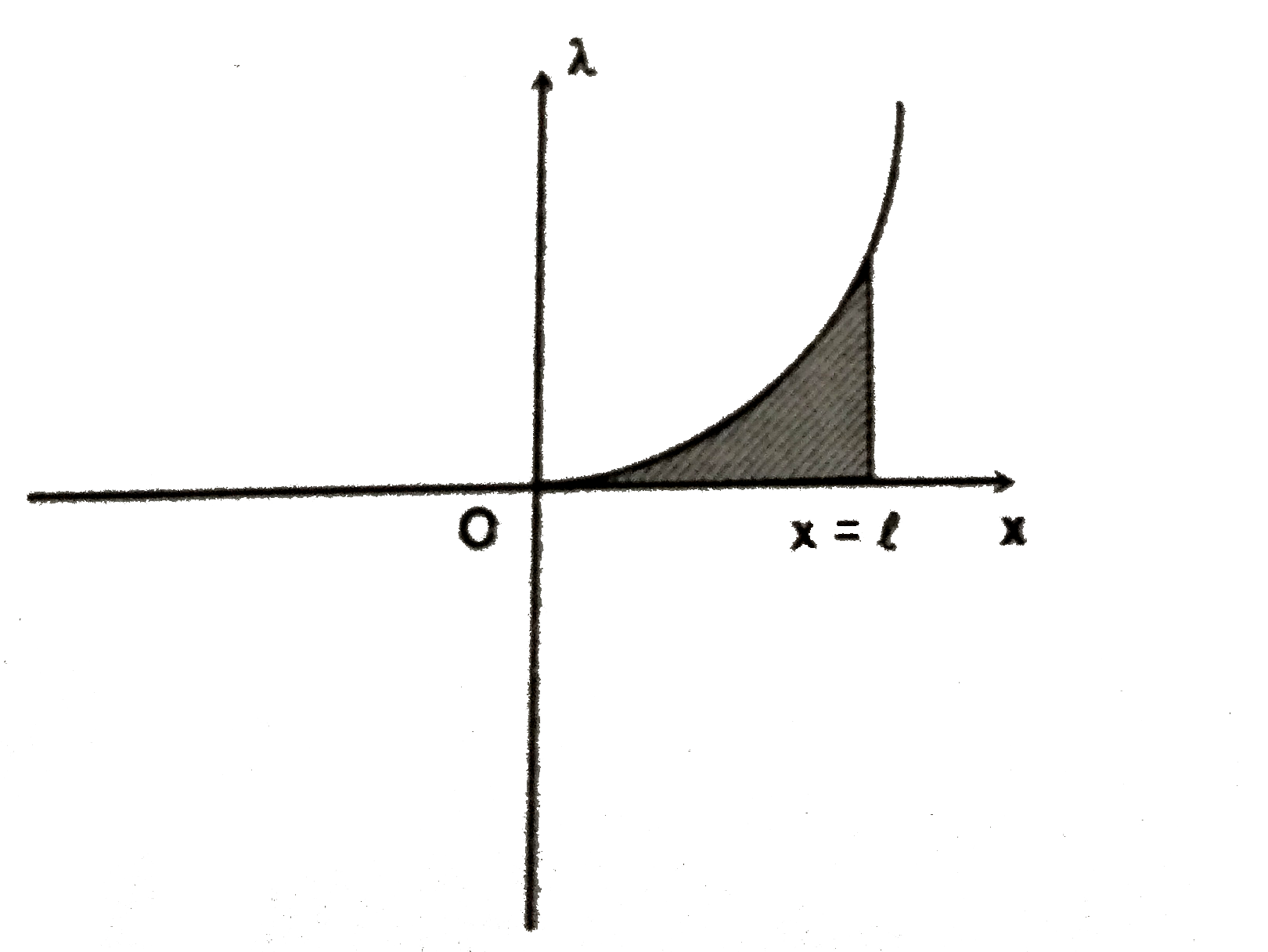
From given funtion it is clear that centre of mass of system must be at distance grater
that l/2. We have only two option 8l15 and 4l5. For graph it is clear that maximum mass existence is nearer to other end, so it can't be 8l15, so right answer will be 4l5
Similar Questions
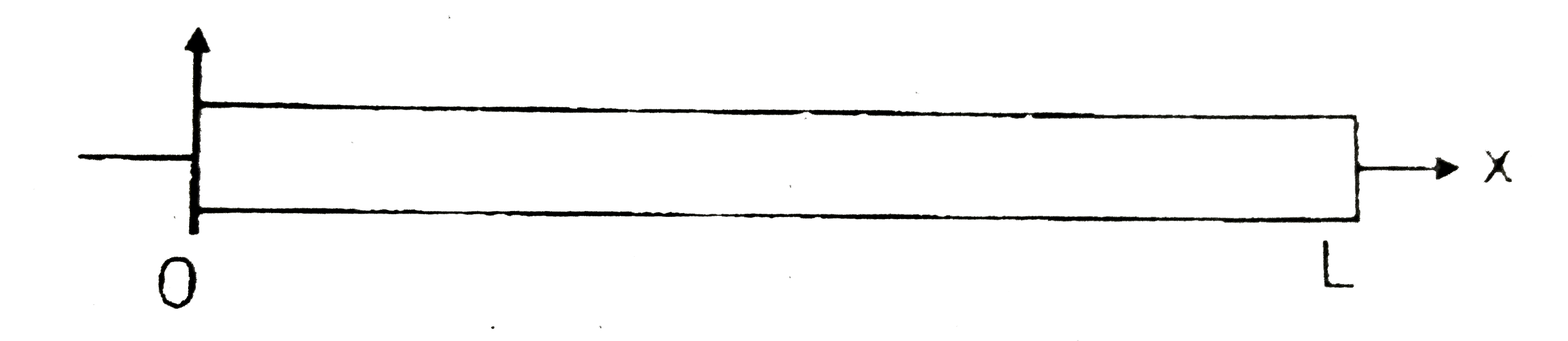
A rod length L and mass M is placed along the x-axis with one end at the origin, as shown in the figure above. The rod has linear mass density λ=2ML2x, where x is the distance from the origin. Which of the following gives the x-coordinate of the rod's center of mass?
 View Solution
View SolutionLinear mass density of a rod AB(of≤n>h10m) varies with distance x from its end A as λ =λ0x3 (λ0 is positive constant) . Distance of centre of mass of the rod , from end B is
View SolutionA non–uniform thin rod of length L is placed along x-axis as such its one of ends at the origin. The linear mass density of rod is λ=λ0x. The distance of centre of mass of rod from the origin is :
View SolutionLinear mass density of a rod AB ( of length 10 m) varied with distance x from its end A as λ=λ0x3 (λ0 is poitive constant). Distance of centre of mass the rod, form end B is
View SolutionA uniform rod AB of length l and mass m is free to rotate about an Axis passing through A and perpendicular to length of the rod. The rod is released from rest as shown in figure. Given that the moment of inertia of the rod about A is ml23 The initial angular acceleration of the rod will be
 View Solution
View SolutionCalculate the moment of inertia of a rod of mass M, and length l about an axis perpendicular to it passing through one of its ends.
View SolutionThe density of a rod of length L varies as ρ=A+Bx where x is the distance from the left end. Locate the centre of mass.
 View Solution
View SolutionThe linear mass density of a thin rod AB of length L varies from A to B as λ(x)=λ0(1+xL). Where x is the distance from A. If M is the mass of the rod then its moment of inertia about an axis passing through A and perpendicualr to the rod is :
View SolutionA metal rod extends on the x−axis from x=0m to x=4m. Its linear density ρ (mass/length) id not uniform and varies with the distance x from the origin according to equation ρ=(1.5+2x)kg/m What is the mass of rod:−
View SolutionThe density of a thin rod of length l varies with the distance x from one end as ρ=ρ0x2l2. Find the position of centre of mass of rod.
View Solution

