A balloon moves up vertically such that if a stone is projected with a horizontal velocity u relative to balloon, the stone always hits the ground at a fixed point at a distance 2u2g horizontally away from it. Find the hight of the balloon as a function of time.
Range =v0×time of flight(t)
⇒t=2v20gv0=2v0g
If y is the height of balloon at any instant t and dydt its velocity then
y=−(dydt)(2v0g)+12g(2v0g)2
⇒dydt+g2v0y−v0=0
dyv0−g2v0y=dt
⇒−2v0gln(v0−g2v0y)=t+c
At t=0, y=0⇒c=−2v0glnv0
Simplfying we get
y=2v20g[1−e−gt/2v0]
Topper's Solved these Questions
Similar Questions
A balloon moves up vertically such that if a stone is thrown from it with a horizontal velocity v0 relative to it the stone always hits the ground at a fixed point 2v20/g horizontally away from it. Find the height of the balloon as a function of time.
View SolutionA ballon moves up with a velocity 5m/s. A stone is thrown from it with a horizontal velocity 2m/s relative to it. The stone hits the ground at a point 10m horizontally away from it. (Take g=10m/s2)
View Solution
Knowledge Check
- Question 1 - Select One
A stone is thrown horizontally with velocity u. The velocity of the stone 0.5 s later is 3u/2. The value of u is
A2.2ms−1B3.3ms−1C4.4ms−1D1.1ms−1
From a ballon rising vertically upwards at 5 m/s, a stone is thrown up at 10 m/s relative to the balloon. Its velocity with respect to ground after 2 sec is - (assume g = 10 m/s2)
View SolutionA ball is thrown vertically upward with a velocity u from balloon descending with a constant velocity v. The vall will pass by the balloon after time
View SolutionA body is projected vertically up with velocity u from a tower. It reaches the ground with velocity nu. The height of the tower is H=u22g(n2−1)
View SolutionA balloon from rest accelerates uniformly upward with 'a' ms−2, for t seconds of time. A stone is released from the balloon. Now, read the following statements to pick the right ones.
(a) The stone's initial velocity is zero, relative to balloon
(b) The stone's initial velocity is nonzero, relative to earth
( c) The time taken to reach the ground from the balloon's frame of reference is inversely proportional to √(a+g)
(d) The time take to reach the ground from earth's frame of reference is directly proportional to √(a+g)View SolutionA ball is thrown vertically upward with a velocity 'u' from the balloon descending with velocity v. After what time, the ball will pass by the balloon ?
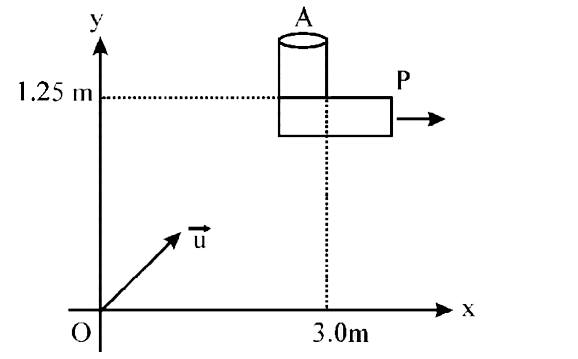
View SolutionAn object A is kept fixed at the point x=3m and y=1.25m on a plank p raised above the ground . At time t=0 the plank starts moving along the +x direction with an acceleration 1.5m/s2. At the same instant a stone is projected from the origin with a velocity →u as shown . A stationary person on the ground observes the stone hitting the object during its downward motion at an angle 45(∘) to the horizontal . All the motions are in the X−Yplane . Find →u and the time after which the stone hits the object . Take g=10m/s
 View Solution
View SolutionAn object A is kept fixed at the point x=3m and y=1.25m on a plank p raised above the ground . At time t=0 the plank starts moving along the +x direction with an acceleration 1.5m/s2. At the same instant a stone is projected from the origin with a velocity →u as shown . A stationary person on the ground observes the stone hitting the object during its downward motion at an angle 45(∘) to the horizontal . All the motions are in the X−Yplane . Find →u and the time after which the stone hits the object . Take g=10m/s
 View Solution
View Solution

