An annular disc of inner radius a and outer radius '2a' is uniformly charged with uniform surface charge density . Find the potential at a distance 'a' from the centre at a point 'P' lying on the axis.
To find the potential at a point P located on the axis of an annular disc with inner radius a and outer radius 2a, uniformly charged with surface charge density σ, we can follow these steps:
Step 1: Define the Geometry
Consider the annular disc with inner radius a and outer radius 2a. The point P is located at a distance a from the center along the axis perpendicular to the plane of the disc.
Step 2: Consider a Differential Element
Take a differential ring element at a distance r from the center of the disc with thickness dr. The area of this ring element is given by:
dA=2πrdr
The charge dq on this differential area is:
dq=σdA=σ⋅2πrdr
Step 3: Calculate the Distance to Point P
The distance r′ from the differential ring to the point P is given by:
r′=√a2+r2
Step 4: Calculate the Potential Contribution from the Differential Element
The potential dV at point P due to the differential charge dq is given by:
dV=dq4πϵ0r′=σ⋅2πrdr4πϵ0√a2+r2=σrdr2ϵ0√a2+r2
Step 5: Integrate Over the Annular Disc
To find the total potential VP at point P, integrate dV from r=a to r=2a:
VP=∫2aadV=∫2aaσrdr2ϵ0√a2+r2
Step 6: Perform the Integration
To solve the integral, we can use the substitution t2=a2+r2, which gives dt=rdr√a2+r2. The limits change as follows:
- When r=a, t=√2a2=a√2
- When r=2a, t=√5a2=a√5
Thus, the integral becomes:
VP=σ2ϵ0∫a√5a√2dt=σ2ϵ0(t)a√5a√2=σ2ϵ0(a√5−a√2)
Step 7: Final Expression for Potential
Thus, the potential at point P is:
VP=σa2ϵ0(√5−√2)
Consider the annular disc with inner radius a and outer radius 2a. The point P is located at a distance a from the center along the axis perpendicular to the plane of the disc.
Take a differential ring element at a distance r from the center of the disc with thickness dr. The area of this ring element is given by:
dA=2πrdr
The charge dq on this differential area is:
dq=σdA=σ⋅2πrdr
The distance r′ from the differential ring to the point P is given by:
r′=√a2+r2
The potential dV at point P due to the differential charge dq is given by:
dV=dq4πϵ0r′=σ⋅2πrdr4πϵ0√a2+r2=σrdr2ϵ0√a2+r2
To find the total potential VP at point P, integrate dV from r=a to r=2a:
VP=∫2aadV=∫2aaσrdr2ϵ0√a2+r2
To solve the integral, we can use the substitution t2=a2+r2, which gives dt=rdr√a2+r2. The limits change as follows:
- When r=a, t=√2a2=a√2
- When r=2a, t=√5a2=a√5
VP=σ2ϵ0∫a√5a√2dt=σ2ϵ0(t)a√5a√2=σ2ϵ0(a√5−a√2)
Thus, the potential at point P is:
VP=σa2ϵ0(√5−√2)
Similar Questions
A charged disc of radius 3 m has charge density σ. Electric field at a distance of 4 m from its centre on axis is _______
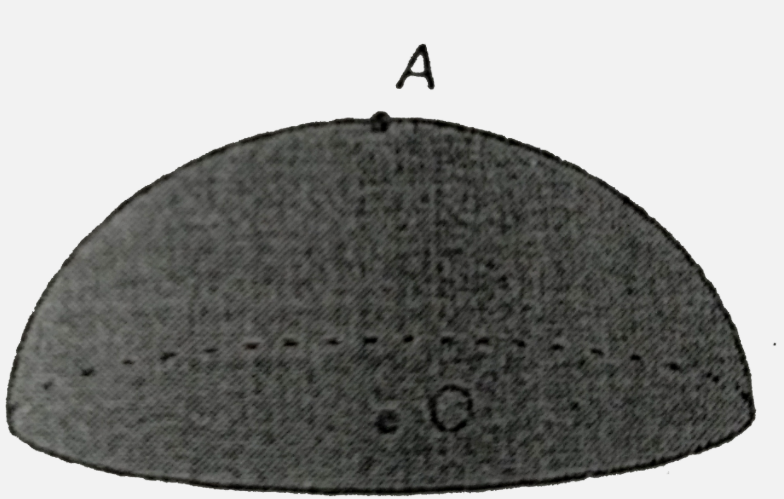
View SolutionA hemispherical bowl of radius R carries a uniform surface charge density σ. Find the potential at the topmost point A, taking potential at infinity to be zero.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Consider a thin spherical shell of radius R consisting of uniform surface charge density σ. The electric field at a point of distance x from its centre and outside the shell is
Ainversely proportional to σBdirectly proportional to x2Cdirectlr proportional to RDinversely proportional to x2
The magnitude of the electric field on the surface of a sphere of radius r having a uniform surface charge density σ is
View SolutionA charge of 5 nC is uniformly distributed on a ring of radius 4 cm. Find the potential at the axis at a distance of 3 cm from the center.
View SolutionFind the electric field caused by a disc of radius a with a uniform surfce charge density σ (charge per unit area) at a point along the axis of the disc a distance x from its centre.
View SolutionSphere of inner radius a and outer radius b is made of p uniform resistivity find resistance between inner and outer surface
View SolutionSphere of inner radius a and outer radius b is made of p uniform resistivity find resistance between inner and outer surface
View Solution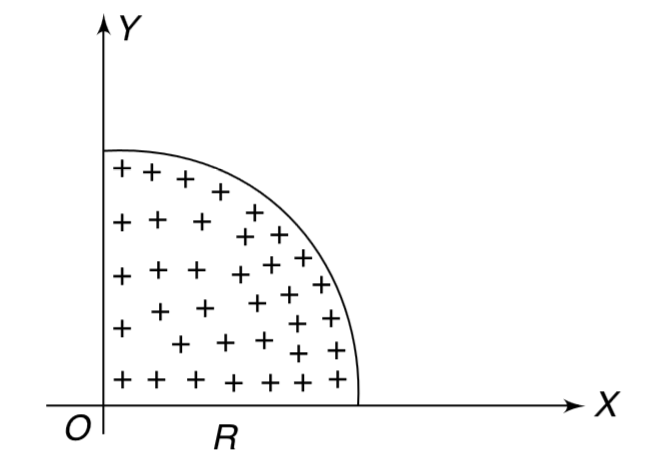
The quarter disc of radius R (see figure) has a uniform surface charge density σ.
(a) Find electric potential at a point (O,O,Z)
(b). Find the Z component of electrif field at (O,O,Z)View SolutionThe electrostatic potential of a uniformly charged thin spherical shell of charge Q and radius R at a distance r from the centre
View Solution

