If net flux through a Gaussian surface is zero, then find whether the following statements are correct or not.
The net charge inside the surface is zero.
To determine whether the statement "The net charge inside the surface is zero" is correct when the net flux through a Gaussian surface is zero, we can follow these steps:
Step 1: Understand Gauss's Law
Gauss's Law states that the electric flux (Φ) through a closed surface is proportional to the charge (Q) enclosed within that surface. Mathematically, it is expressed as:
Φ=Qencϵ0
where:
- Φ is the electric flux through the surface,
- Qenc is the net charge enclosed within the surface,
- ϵ0 is the permittivity of free space.
Step 2: Analyze the Given Condition
The problem states that the net flux through the Gaussian surface is zero:
Φ=0
Step 3: Apply Gauss's Law
Using Gauss's Law, we can substitute the zero flux into the equation:
0=Qencϵ0
Step 4: Solve for the Enclosed Charge
From the equation above, we can multiply both sides by ϵ0:
0=Qenc
This implies that:
Qenc=0
Step 5: Conclusion
Since we have determined that the net charge enclosed within the Gaussian surface is zero, we can conclude that the statement "The net charge inside the surface is zero" is indeed correct.
Final Answer
The statement is correct: The net charge inside the Gaussian surface is zero.
---
Gauss's Law states that the electric flux (Φ) through a closed surface is proportional to the charge (Q) enclosed within that surface. Mathematically, it is expressed as:
Φ=Qencϵ0
where:
- Φ is the electric flux through the surface,
- Qenc is the net charge enclosed within the surface,
- ϵ0 is the permittivity of free space.
The problem states that the net flux through the Gaussian surface is zero:
Φ=0
Using Gauss's Law, we can substitute the zero flux into the equation:
0=Qencϵ0
From the equation above, we can multiply both sides by ϵ0:
0=Qenc
This implies that:
Qenc=0
Since we have determined that the net charge enclosed within the Gaussian surface is zero, we can conclude that the statement "The net charge inside the surface is zero" is indeed correct.
The statement is correct: The net charge inside the Gaussian surface is zero.
Similar Questions
If net flux through a Caussian surface is zero, then find whether the following statements are correct or not.
There are no changes inside the surface.
View SolutionIf net flux through a Caussian surface is zero, then find whether the following statements are correct or not.
The number of field lines entering the surface equals to the number of field lines leaving the surface.
View SolutionIf net flux through a Caussian surface is zero, then find whether the following statements are correct or not.
The electric field just outside a charged conductor is perpendicular to the surface of the conductor and has a magnitude of σ/2ε0 where σ is the charge per unit area at that point.
View SolutionFind out flux through the given Gaussian surface.
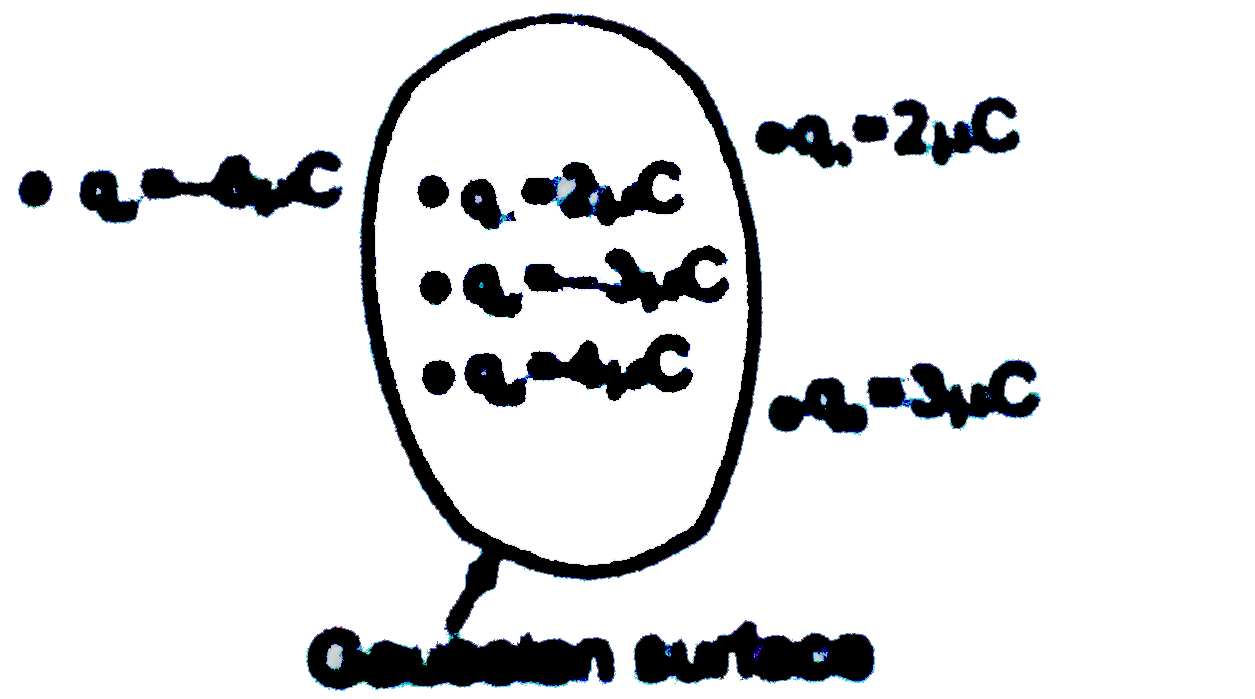
View SolutionIf net force on a system of particles is zero, then match the following.

View SolutionIn which of the following cases the net force is not zero ?
View SolutionFind flux through the hemispherical surface
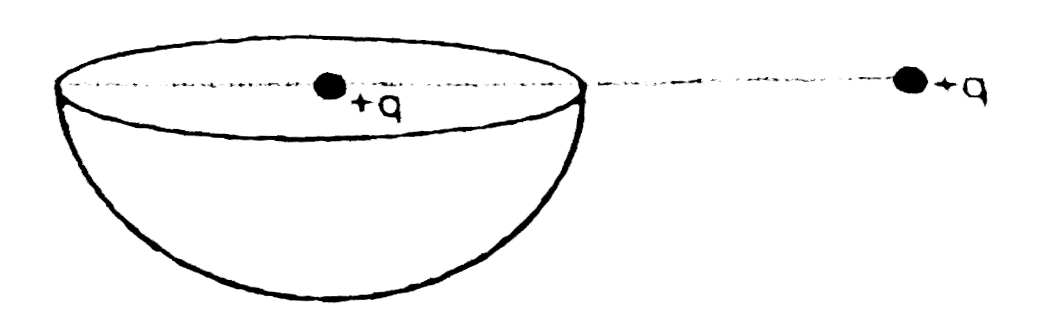
View SolutionGauss's law and Coulomb's law , although expressed in different forms , are equivalent ways of describing the relation between charge and electric field in static conditions . Gauss's law is ε0ϕ=qencl,when q(encl) is the net charge inside an imaginary closed surface called Gaussian surface. The two equations hold only when the net charge is in vaccum or air .
If the charge q3 and q4 are displaced (always remaining outside the Gaussian surface), then consider the following two statements :
A: Electric field at each point on the Gaussian surface will remain same .
B: The value of ∮→E.d→A for the Gaussian surface will remain same.
View SolutionThe net flux passing through a closed surface enclosing unit charge is
View SolutionIf the flux of the electric field through a closed surface is zero,
View Solution
