If net flux through a Caussian surface is zero, then find whether the following statements are correct or not.
There are no changes inside the surface.
To determine whether the statement "There are no charges inside the surface" is correct when the net flux through a Gaussian surface is zero, we can analyze the situation using Gauss's Law.
1. Understanding Gauss's Law:
Gauss's Law states that the electric flux (Φ) through a closed surface (Gaussian surface) is proportional to the charge (Q) enclosed within that surface. Mathematically, it is expressed as:
Φ=Qenclosedϵ0
where ϵ0 is the permittivity of free space.
2. Given Condition:
The problem states that the net flux through the Gaussian surface is zero:
Φ=0
3. Applying Gauss's Law:
From Gauss's Law, if the net flux is zero, we can set up the equation:
0=Qenclosedϵ0
This implies that:
Qenclosed=0
4. Interpreting the Result:
The result Qenclosed=0 indicates that the total charge enclosed by the Gaussian surface is zero. However, this does not necessarily mean that there are no charges inside the surface.
5. Possible Scenarios:
- There could be equal amounts of positive and negative charges inside the surface that cancel each other out, resulting in a net charge of zero.
- There could also be no charges at all inside the surface.
6. Conclusion:
Therefore, the statement "There are no charges inside the surface" is not necessarily true. It is possible to have charges inside the surface that sum to zero.
Final Answer:
The statement is false. There can be charges inside the surface, but their net charge can be zero.
Gauss's Law states that the electric flux (Φ) through a closed surface (Gaussian surface) is proportional to the charge (Q) enclosed within that surface. Mathematically, it is expressed as:
Φ=Qenclosedϵ0
where ϵ0 is the permittivity of free space.
The problem states that the net flux through the Gaussian surface is zero:
Φ=0
From Gauss's Law, if the net flux is zero, we can set up the equation:
0=Qenclosedϵ0
This implies that:
Qenclosed=0
The result Qenclosed=0 indicates that the total charge enclosed by the Gaussian surface is zero. However, this does not necessarily mean that there are no charges inside the surface.
- There could be equal amounts of positive and negative charges inside the surface that cancel each other out, resulting in a net charge of zero.
- There could also be no charges at all inside the surface.
Therefore, the statement "There are no charges inside the surface" is not necessarily true. It is possible to have charges inside the surface that sum to zero.
The statement is false. There can be charges inside the surface, but their net charge can be zero.
Similar Questions
If net flux through a Gaussian surface is zero, then find whether the following statements are correct or not.
The net charge inside the surface is zero.
View SolutionIf net flux through a Caussian surface is zero, then find whether the following statements are correct or not.
The number of field lines entering the surface equals to the number of field lines leaving the surface.
View Solution
Knowledge Check
A drop of oil is placed on the surface of water. Which of the following statement is correct.
Ait will remain on it as a sphereBit will spread as a thin layerCit will partly be as spherical droplets and partly as thin filmDit will float as a distorted drop on the water surface
State whether the following statement is correct or not?
View SolutionIf net flux through a Caussian surface is zero, then find whether the following statements are correct or not.
The electric field just outside a charged conductor is perpendicular to the surface of the conductor and has a magnitude of σ/2ε0 where σ is the charge per unit area at that point.
View SolutionThe electric flux through the surface

View SolutionThe electric flux through the surface

View SolutionThe electric flux through the surface

View SolutionFind out flux through the given Gaussian surface.
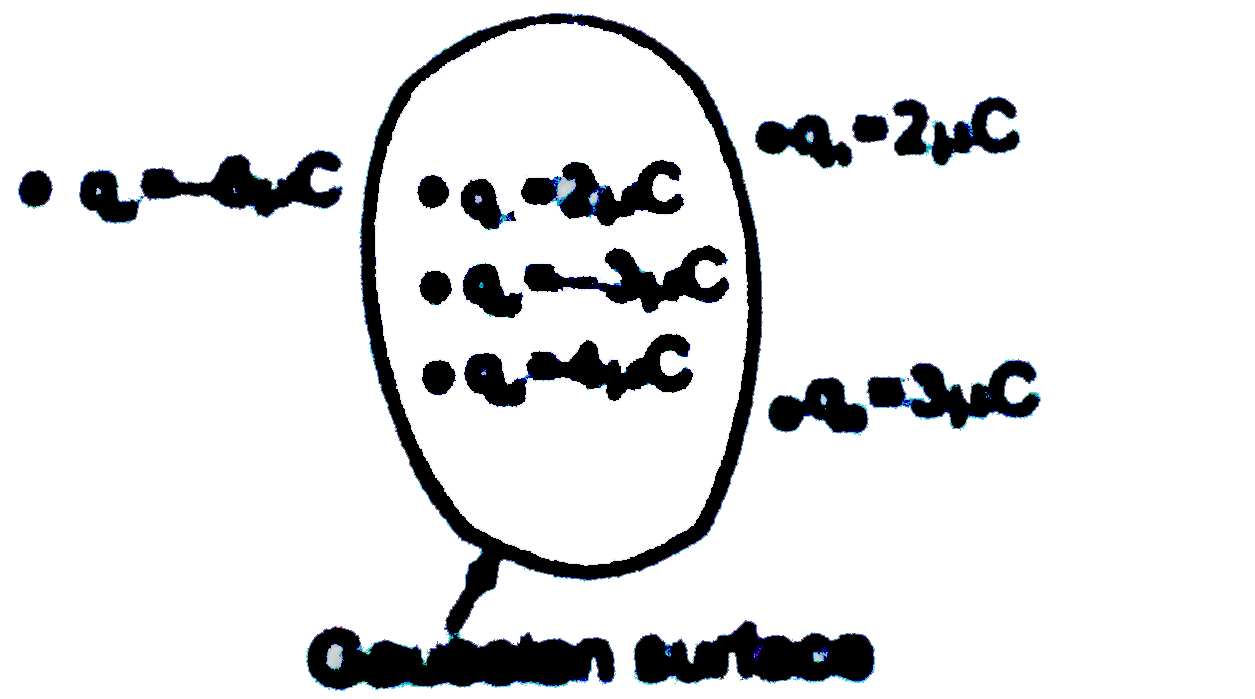
View SolutionFind flux through the hemispherical surface
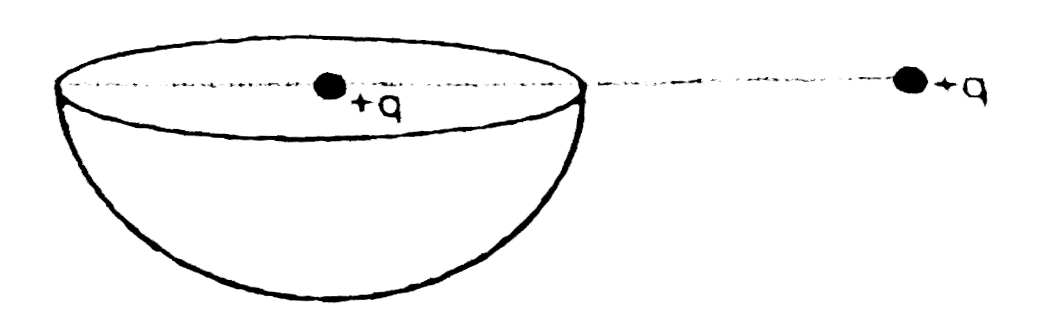
View Solution
