Column - IColumn - II(A)Coordination number(p)FCC(B)9 places of symmetry.(q)Line passing through centres of two diagonal opposite edges(C)2 fold axis of symmetry(r)Decrease with increase in temperature(D)Coordination number = 12(s)SCC
To solve the matching question between Column - I and Column - II, we will analyze each item in Column - I and find the corresponding item in Column - II.
1. Coordination Number (A):
- The coordination number is defined as the number of atoms immediately surrounding a central atom in a crystal structure.
- In the case of an FCC (Face-Centered Cubic) structure, the coordination number is 12.
- Therefore, A matches with (D) Coordination number = 12.
2. 9 Places of Symmetry (B):
- A simple cubic structure has 9 places of symmetry.
- Therefore, B matches with (S) SCC (Simple Cubic Crystal).
3. 2 Fold Axis of Symmetry (C):
- A 2-fold axis of symmetry occurs when a line passes through the centers of two diagonal opposite edges.
- Therefore, C matches with (Q) Line passing through centers of two diagonal opposite edges.
4. Coordination Number = 12 (D):
- As previously established, the coordination number of 12 corresponds to the FCC structure.
- Therefore, D matches with (P) FCC.
5. Decrease with Increase in Temperature (R):
- As temperature increases, the average energy of the particles increases, causing them to move apart, which leads to a decrease in coordination number.
- Therefore, R matches with (A) Coordination number.
Final Matches:
- A → D (Coordination number = 12 for FCC)
- B → S (9 places of symmetry for SCC)
- C → Q (2 fold axis of symmetry)
- D → P (Coordination number = 12 for FCC)
- R → A (Coordination number decreases with increase in temperature)
Summary of Matches:
- A → D
- B → S
- C → Q
- D → P
- R → A
- The coordination number is defined as the number of atoms immediately surrounding a central atom in a crystal structure.
- In the case of an FCC (Face-Centered Cubic) structure, the coordination number is 12.
- Therefore, A matches with (D) Coordination number = 12.
- A simple cubic structure has 9 places of symmetry.
- Therefore, B matches with (S) SCC (Simple Cubic Crystal).
- A 2-fold axis of symmetry occurs when a line passes through the centers of two diagonal opposite edges.
- Therefore, C matches with (Q) Line passing through centers of two diagonal opposite edges.
- As previously established, the coordination number of 12 corresponds to the FCC structure.
- Therefore, D matches with (P) FCC.
- As temperature increases, the average energy of the particles increases, causing them to move apart, which leads to a decrease in coordination number.
- Therefore, R matches with (A) Coordination number.
- A → D (Coordination number = 12 for FCC)
- B → S (9 places of symmetry for SCC)
- C → Q (2 fold axis of symmetry)
- D → P (Coordination number = 12 for FCC)
- R → A (Coordination number decreases with increase in temperature)
- A → D
- B → S
- C → Q
- D → P
- R → A
Similar Questions
A solid sphere is rotating about an axis shown in figure. An insect follows the dotted path on the circumference of sphere as shown in the figure.
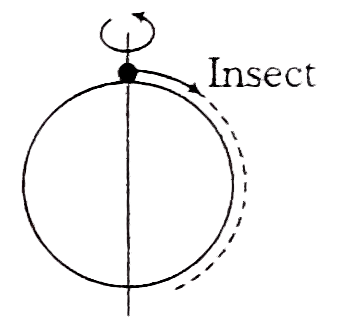
Column-IColumn-II(A)Moment of inertia(p)Will remain constant(B)Angular velocity(q)Will first increase, then decrease(C)Angular momentum(r)Will first decrease then increase(D)Rotational kinetic energy(s)Will continuously decrease(t)Will continuously increaseView SolutionColumn-IColumn-II(A)74% occupancy of space(p)cubic close packing of identical spheres(B)Coordination number =6(q)hexagonal close packing of identical spheres.(C)68% occupancy of space(r)body centered cubic packing of identical spheres.(D)Coordination number =12(s)simple cubic packing of identical spheres(t)AB AB AB ....type of close packing of identical spheres
View SolutionIf net force on a system of particles is zero. Then
Column IColumn II(A)Acceleration of centre of mass(p)Constant(B)Velocity of centre of mass(q)Zero(C)Momentum of centre of mass(r)May be zero(D)Velocity of an individual particle of the system(s)May be constantView SolutionColumn IColumn II(A)Dimensional variable(p)π(B)Dimensionless variable(q)Force(C)Dimensional constant(r)Angle(D)Dimensionless constant(s)Gravitational constant
View SolutionColumn IColumn II(A)Stress(p)Pressure(B)Strain(q)Energy density(C)Modulus of elasticity(r)Angle(D)Torque(s)Energy
View SolutionIn elliptical orbit of a planet, as the planet moves from apogee position to perigee position to perigee position, match the following columns
Column-IColumn-II(A)Speed of planet(p)Remains same(B)Distance of planet from centre of sun(q)Decreases(C)Potential energy(r)Increase(D)Angular momentum about centre of sun(s)Cannot sayView SolutionIf earth decreases its rotational speed. Match the following columns
Column-IColumn-II(A)Value of g at pole(p)Will remain same(B)Value of g at equator(q)Will increase(C)Distance of geostationary(r)Will decrease(D)Energy of geostationary satellite(s)Cannot sayView SolutionColumn-IColumn-II(a)Crypts of Liberkuhn (p)Loop of duodenum(b)Pancreas(q)Stomach(c)Adrenal gland(r)Intestine(d)Gastric glands (s)Kidney
View SolutionThe straight lines in the figure depict the variations in temperature ΔT as a function of the amount of heat supplied Q is different process involving the change of state of a monoatomic and a diatomic ideal gas . The initial states, (P,V,T) of the two gases are the same . Match the processes as described, with the straight lines in the graph as numbered.
_E01_155_Q01.png)
Column-IColumn-II(A)Isobaric process of monoatomic gas.(p)1(B)Isobaric process of diatomic gas(q)2(C)Isochoric process of monoatomic gas(r)Minimum at section B(D)Isochoric process of diatomic gas(s)x-axis (i.e.'Q' axis)View SolutionColumn-IColumn-II(A)Cathode rays(p)Helium nuclei(B)dumb-bell(q)Uncertainty principle(C)Alpha particles(r)Electromagnetic radiation(D)Moseley(s)p-orbital(E)Heisenberg(t)Atomic number(F)X-ray(u)electrons
View Solution

