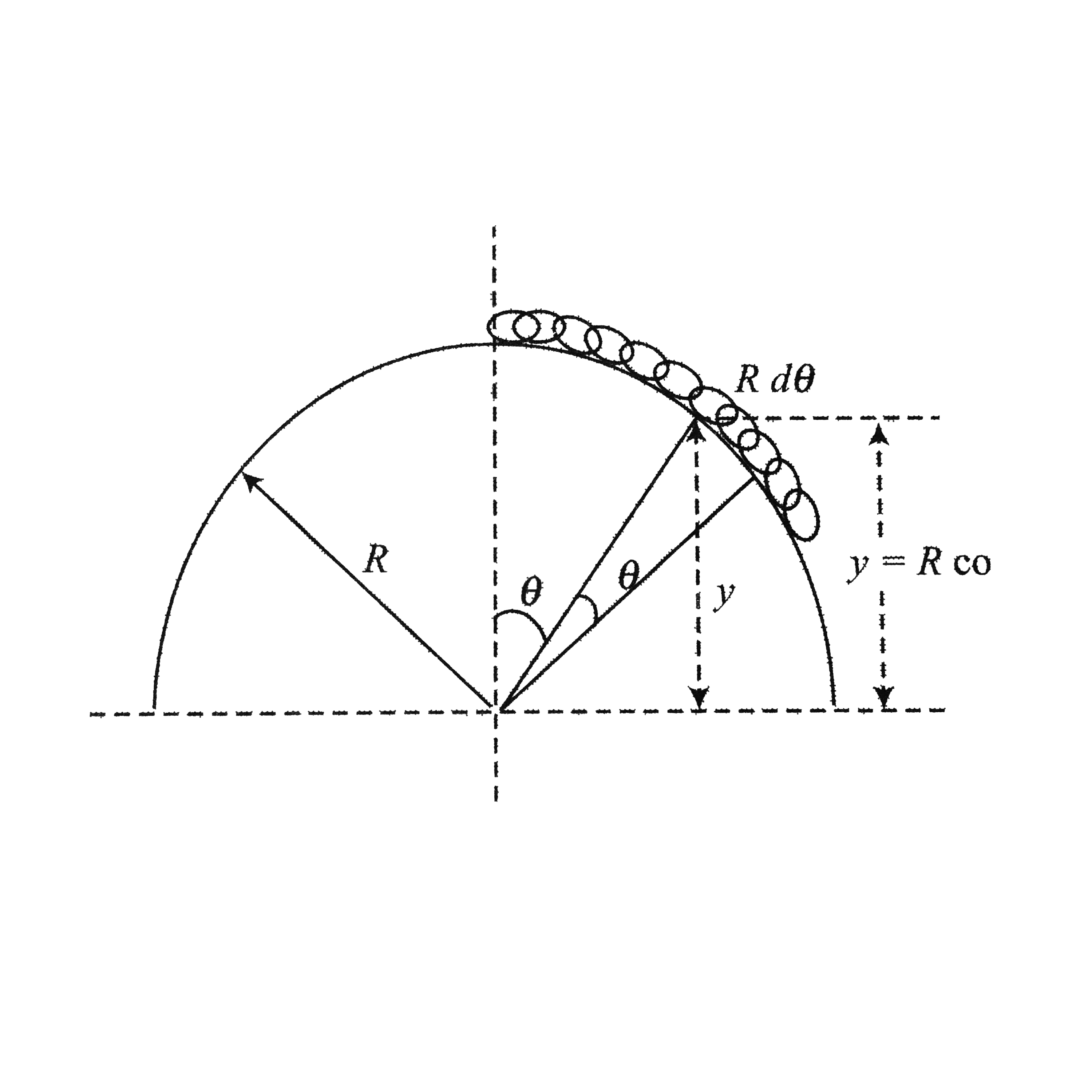
A chain of length l is placed on a smooth spherical surface of radius R with one of its ends fixed at the top of the sphere. What will be the acceleration w of each element of the chain when its upper end is released? It is assumed that the length of the chain l<12πR.
The correct Answer is:(Rg/l)/{1−cos(l/R)}
To solve the problem of determining the acceleration w of each element of the chain when its upper end is released, we will follow these steps:
Step 1: Define the Setup
We have a chain of length l placed on a smooth spherical surface of radius R, with one end fixed at the top of the sphere. The chain can slide down the surface when released. We are given that l<πR2.
Step 2: Determine Mass per Unit Length
Let the mass of the chain be m. The mass per unit length λ of the chain is given by:
λ=ml
Step 3: Consider a Small Element of the Chain
Consider a small element of the chain of length dl at an angle θ from the vertical. The mass of this small element dm is:
dm=λ⋅dl=ml⋅dl
Step 4: Calculate the Force Acting on the Element
The force acting on this small element due to gravity is:
dF=dm⋅g⋅sin(θ)
Substituting for dm:
dF=(ml⋅dl)⋅g⋅sin(θ)
Step 5: Integrate the Force
To find the total force acting on the chain as it slides down, we need to integrate this force from 0 to a (where a is the angle corresponding to the length of the chain):
F=∫a0dF=∫a0(ml⋅g⋅sin(θ)⋅dl)
Using the relationship dl=R⋅dθ:
F=mgl∫a0Rsin(θ)dθ
Step 6: Evaluate the Integral
The integral ∫sin(θ)dθ evaluates to −cos(θ). Therefore:
F=mgRl(−cos(θ))a0=mgRl(1−cos(a))
Step 7: Relate Force to Acceleration
The net force acting on the chain is equal to the mass of the chain times its acceleration w:
F=m⋅w
Setting the two expressions for force equal gives:
m⋅w=mgRl(1−cos(a))
Dividing both sides by m:
w=gRl(1−cos(a))
Step 8: Substitute for a
Since a=lR (from the geometry of the problem), we can substitute this into our expression for w:
w=gRl(1−cos(lR))
Final Answer
Thus, the acceleration w of each element of the chain when its upper end is released is:
w=gRl(1−cos(lR))
---
We have a chain of length l placed on a smooth spherical surface of radius R, with one end fixed at the top of the sphere. The chain can slide down the surface when released. We are given that l<πR2.
Let the mass of the chain be m. The mass per unit length λ of the chain is given by:
λ=ml
Consider a small element of the chain of length dl at an angle θ from the vertical. The mass of this small element dm is:
dm=λ⋅dl=ml⋅dl
The force acting on this small element due to gravity is:
dF=dm⋅g⋅sin(θ)
Substituting for dm:
dF=(ml⋅dl)⋅g⋅sin(θ)
To find the total force acting on the chain as it slides down, we need to integrate this force from 0 to a (where a is the angle corresponding to the length of the chain):
F=∫a0dF=∫a0(ml⋅g⋅sin(θ)⋅dl)
Using the relationship dl=R⋅dθ:
F=mgl∫a0Rsin(θ)dθ
The integral ∫sin(θ)dθ evaluates to −cos(θ). Therefore:
F=mgRl(−cos(θ))a0=mgRl(1−cos(a))
The net force acting on the chain is equal to the mass of the chain times its acceleration w:
F=m⋅w
Setting the two expressions for force equal gives:
m⋅w=mgRl(1−cos(a))
Dividing both sides by m:
w=gRl(1−cos(a))
Since a=lR (from the geometry of the problem), we can substitute this into our expression for w:
w=gRl(1−cos(lR))
Thus, the acceleration w of each element of the chain when its upper end is released is:
w=gRl(1−cos(lR))
Similar Questions
A chain of length l and mass m lies on the surface of a smooth sphere of radius R>l with one end tied to the top of the sphere.
View SolutionA chain of length l and mass m lies of the surface of a smooth hemisphere of radius R>l with one end tied to the top of the hemisphere. Taking base of the hemisphere as reference line, find the gravitational potential energy of the chain.
View SolutionA chain of length L and mass m is placed upon a smooth surface. The length of BA is (L−b). Calculate the velocity of the chain when its and reaches B
View SolutionA chain AB of length l is located on a smooth horizontal table so that its fraction of length h hangs freely with end B on the table. At a certain moment, the end A of the chain is set free. With what velocity with this end of the chain slip off the table?

View SolutionA uniform chain of length l and mass m is placed on a smooth table with one-fourth of its length hanging over the edge. The work that has to be done to pull the whole chain back onto the table is :
View SolutionA uniform chain of length l is placed on a smooth horizontal table, such that half of its length hangs over one edge. It is releasedfrom rest, the velocity with which it leaves the table is
View SolutionA unifrom flexible chain of length 32 m rests on a fixed smooth sphere of radius R=2π m such that one end A of chain is on the top of the sphere while the other end B is hanging freely. Chain is helpd stationary by a horizontal thread PA as shown . Calculate the acceleration of chain when the horizontal string PA is burnt ( g = 10 m/s2)
View SolutionA chain (AB) of length l loaded in a smooth horizontal table so that its fraction of length h hangs freely and toucher the surface of the table with its end B. At a certain moment ,the end A of the chain is set free. With what velocity will this end the chain slip out of the table ?
 .
.
View SolutionA uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

What is the speed of the chain, when it just loses contact with the table?
View SolutionA chain of mass m and length L is held at rest on smooth horizontal surface such that a part I of the chain is hanging vertically from the table. If the chain is let go, what is its speed as its end just leaves the horizontal surface?
View Solution
