If W1:W2=2:3 and W1:W3=1:2, then W2:W3 is:
3:4
4:3
2:3
4:5
The correct Answer is:A
To solve the problem, we need to find the ratio W2:W3 given the ratios W1:W2=2:3 and W1:W3=1:2.
1. Express the Ratios in Fraction Form:
- From the ratio W1:W2=2:3, we can express this as:
W1W2=23
- From the ratio W1:W3=1:2, we can express this as:
W1W3=12
2. Express W2 and W3 in terms of W1:
- From W1W2=23, we can rearrange it to find W2:
W2=32W1
- From W1W3=12, we can rearrange it to find W3:
W3=2W1
3. Substitute W1 in terms of W2 or W3:
- We can express W1 in terms of W2:
W1=23W2
- Now substitute W1 into the equation for W3:
W3=2(23W2)=43W2
4. Find the Ratio W2:W3:
- Now we can find the ratio W2:W3:
W2:W3=W2:43W2
- This simplifies to:
W2:W3=1:43
- To express this in a simpler form, we can multiply both sides by 3:
W2:W3=3:4
Final Answer:
Thus, the ratio W2:W3 is 3:4.
- From the ratio W1:W2=2:3, we can express this as:
W1W2=23
- From the ratio W1:W3=1:2, we can express this as:
W1W3=12
- From W1W2=23, we can rearrange it to find W2:
W2=32W1
- From W1W3=12, we can rearrange it to find W3:
W3=2W1
- We can express W1 in terms of W2:
W1=23W2
- Now substitute W1 into the equation for W3:
W3=2(23W2)=43W2
- Now we can find the ratio W2:W3:
W2:W3=W2:43W2
- This simplifies to:
W2:W3=1:43
- To express this in a simpler form, we can multiply both sides by 3:
W2:W3=3:4
Thus, the ratio W2:W3 is 3:4.
Topper's Solved these Questions
- RATIO AND PROPORTION Book:KIRAN PUBLICATIONChapter:RATIO AND PROPORTION Exercise:TYPE-IV
Explore 30 Videos
- RATIO AND PROPORTION Book:KIRAN PUBLICATIONChapter:RATIO AND PROPORTION Exercise:TYPE-V
Explore 64 Videos
- RATIO AND PROPORTION Book:KIRAN PUBLICATIONChapter:RATIO AND PROPORTION Exercise:TYPE-II
Explore 49 Videos
- PROFIT AND LOSS Book:KIRAN PUBLICATIONChapter:PROFIT AND LOSS Exercise:TEST YOURSELF
Explore 23 Videos
- SEQUENCE AND SERIES Book:KIRAN PUBLICATIONChapter:SEQUENCE AND SERIES Exercise:TEST YOURSELF
Explore 20 Videos
Similar Questions
If W1:W2=2:3 and W1:W3=1:2 the W2:W3 is 3:4 b. 4:3 c. 2:3 d. 4:5
View SolutionWhich of the following can be the valid assignment of probability for outcomes of sample space, S={W1,W2,W3},{ where W1,W2 and W3 are mutually exclusive events
Assignment
w1w2w3(a)0.40.20.8(b)001(c)−123412(d)121214
View Solution
Knowledge Check
W1,W2 and W3 are the different sizes of windows 1,2, and 3 respectively placed in a vertical plane. A particle is thrown up in the vertical plane. Let t1,t2 and t3 are the time taken to cross the window W1,W2 and W3 respectively and ΔV1,ΔV2 and ΔV3 are the change in speed after respective window cross.
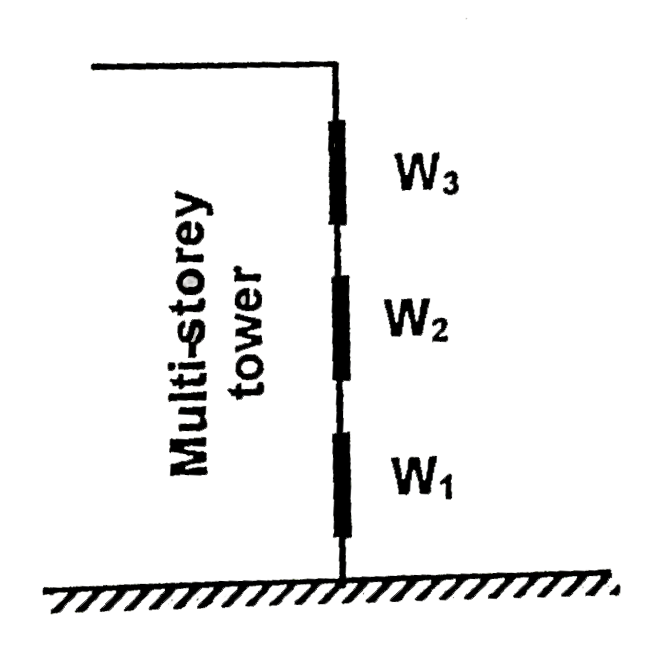 AAverage speed of the particle passing the windows may be equal if W1<W2<W3BAverage speed of the particle passing the windows may be equal if W1>W2>W3CIf W1=W2=WP3, the change in speed of the particle while crossing the windows will satisfy ΔV1<ΔV2<ΔV3DIf W1=W2=W3, the time taken by particle to cross the windows will satisfy t1<t2<t3
AAverage speed of the particle passing the windows may be equal if W1<W2<W3BAverage speed of the particle passing the windows may be equal if W1>W2>W3CIf W1=W2=WP3, the change in speed of the particle while crossing the windows will satisfy ΔV1<ΔV2<ΔV3DIf W1=W2=W3, the time taken by particle to cross the windows will satisfy t1<t2<t3If W1W2,W3andW4 are work done in isothermal, adiabatic, isobaric and isochoric reversible process, the correct order (for expansion) will be
AW1>W2>W3>W4BW3>W2>W1>W4CW3>W2>W4>W1DW3>W1>W2>W4If W1W2 and W3 represent the work done in moving a particle from A to B along three different paths 1.2 and3 respectively (as shown ) in the gravitational fieled of a point mass m, find the correct relation between W_(1) W_(2)andW_(3)
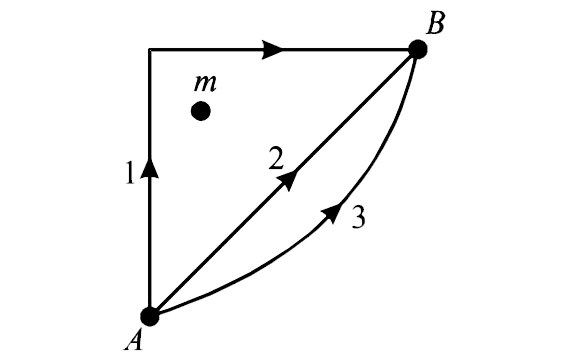 AW1>W2>W3BW1=W2=W3CW1<W2<W3DW2>W1>W3
AW1>W2>W3BW1=W2=W3CW1<W2<W3DW2>W1>W3
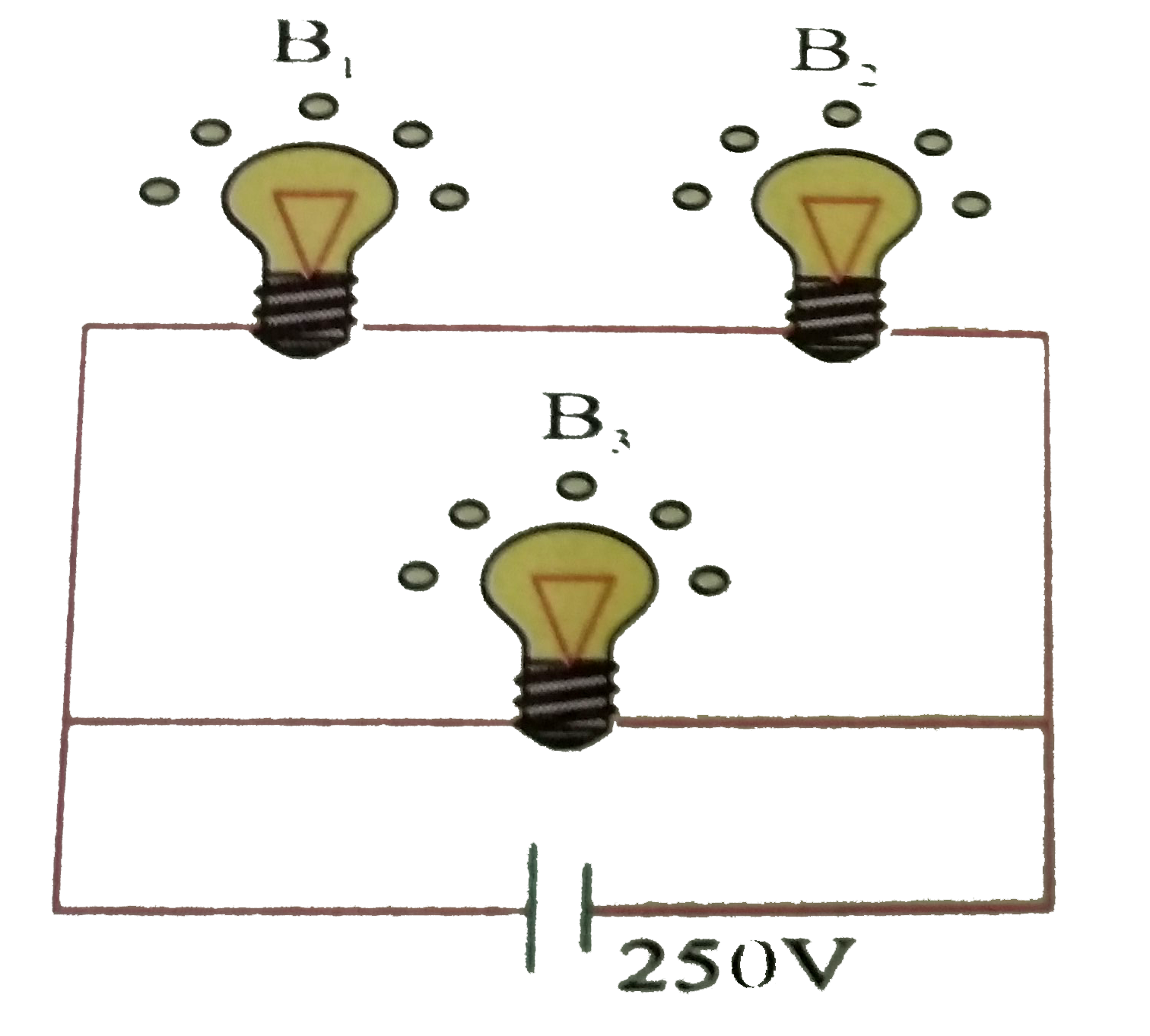
A 100 W bulb B1 and two 60 W bulbs B2 and B3, are connected to a 250V source, as shown in the figure now W1,W2 and W3 are the output powers of the bulbs B1,B2 and B3 respectively then
View Solution[W_(1),W_(2),W_(3)" are apparent "],[" weights of a solid in water at "],[0^(0)C,4^(0)c" and "50^(0)c],[" respectively.If expansion of "],[" solid is negligible,then "],[" A) "W_(1)=W_(2)=W_(3)],[" B) "W_(1)>W_(2)>W_(3)],[" C) "W_(3)>W_(1)>W_(2)],[" D) "W_(3)>W_(2)>W_(1)]
View SolutionThe value of (1+ω2+2ω)3n−(1+ω+2ω)3n is equal to
View SolutionIf ω is a non-real cube root of unity, then Δ=∣∣ ∣ ∣∣a1+b1ωa1ω2+b1a1+b1+c1ω2a2+b2ωa2ω2+b2a2+b2ω+c2ω2a3+b3ωa3ω2+b3a3+b3ω+c3ω2∣∣ ∣ ∣∣ is equal to
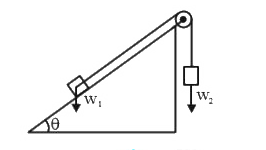
View SolutionIn the accompanying diagram W1 is 5kg weight and W2 is 3 kg weight. If the component of W1 parallel to the incline is equal to W2, then the angle θ is nearly :
View Solution