In an isosceles trapezium ______.
Diagonals are congruent
Opposite sides are parallel
Diagonals are bisectors of each other
Diagonals bisect opposite angles
The correct Answer is:A
To solve the question regarding the properties of an isosceles trapezium, we will analyze the statements provided and prove which one is correct.
1. Identify the Properties of an Isosceles Trapezium:
- In an isosceles trapezium, one pair of opposite sides is parallel (let's say AD || BC) and the other pair of sides (AB and CD) are equal in length.
2. State the Given Information:
- We are given that the trapezium is isosceles, which means that the non-parallel sides (AB and CD) are equal in length.
3. Use the Properties of Angles:
- The base angles of an isosceles trapezium are equal. Therefore, ∠A = ∠B and ∠C = ∠D.
4. Analyze the Diagonals:
- The diagonals of the trapezium are AC and BD. To show that they are congruent, we will use the congruence of triangles.
5. Form Triangles:
- Consider triangles ABC and DBC. We will show that these two triangles are congruent.
- We have:
- AB = CD (since it is an isosceles trapezium)
- ∠A = ∠B (base angles are equal)
- BC = BC (common side)
6. Apply the Side-Angle-Side (SAS) Congruence Criterion:
- Since we have two sides and the included angle of triangle ABC equal to the two sides and included angle of triangle DBC, we can say that:
- Triangle ABC ≅ Triangle DBC (by SAS).
7. Conclude About the Diagonals:
- By the property of congruent triangles, the corresponding parts of congruent triangles are equal. Therefore, we have:
- AC = BD.
8. Final Conclusion:
- Thus, in an isosceles trapezium, the diagonals are congruent.
Final Answer:
In an isosceles trapezium, the diagonals are congruent.
---
- In an isosceles trapezium, one pair of opposite sides is parallel (let's say AD || BC) and the other pair of sides (AB and CD) are equal in length.
- We are given that the trapezium is isosceles, which means that the non-parallel sides (AB and CD) are equal in length.
- The base angles of an isosceles trapezium are equal. Therefore, ∠A = ∠B and ∠C = ∠D.
- The diagonals of the trapezium are AC and BD. To show that they are congruent, we will use the congruence of triangles.
- Consider triangles ABC and DBC. We will show that these two triangles are congruent.
- We have:
- AB = CD (since it is an isosceles trapezium)
- ∠A = ∠B (base angles are equal)
- BC = BC (common side)
- Since we have two sides and the included angle of triangle ABC equal to the two sides and included angle of triangle DBC, we can say that:
- Triangle ABC ≅ Triangle DBC (by SAS).
- By the property of congruent triangles, the corresponding parts of congruent triangles are equal. Therefore, we have:
- AC = BD.
- Thus, in an isosceles trapezium, the diagonals are congruent.
In an isosceles trapezium, the diagonals are congruent.
Topper's Solved these Questions
- BOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XII)90videos
GEOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XIII)70videos
GEOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- X)25videos
GEOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - DISCOUNTEXERCISE - Test Yourself10videos
DISCOUNT
- BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself18videos
LCM AND HCF
Similar Questions
Knowledge Check
- Question 1 - Select One
An isosceles trapezium has
Aequal parallel sidesBequal nonparallel sidesCequal opposite sidesDnone of these - Question 2 - Select One
ABCD is an isosceles trapezium . ¯¯¯¯¯¯AB∣∣¯¯¯¯¯¯CD. AE and BF are the perpendicular drawn to CD. The congruence property used to prove the congurence of triangles AED and BFC is ______
ARHSBSASCSSSDASA - Question 3 - Select One
Which of the following statements is/are correct?
A kite always has two axes of symmetry
A square is both a kite and an isosceles trapezium
Every kite is an orthodiagonal
an isosceles trapezium can have a circumcircle
A kite can always have an incircle
If the sum of two opposite sides is not the same as the sum of the other pair of opposite sides, it can never be a circumscribing quadrilateral.Aall, except (ii) and (iv)Bonly (ii), (iii) and (v)Conly (i), (iii), (v)Dall, except (i)
The number of independent measurement required to construct an isosceles trapezium is three.
View SolutionThe number of independent measurement required to construct an isosceles trapezium is three.
View SolutionIsosceles trapezium a trapezium is said to be an isosceles trapezium if its non-parallel sides are equal.
View SolutionIsosceles trapezium a trapezium is said to be an isosceles trapezium if its non-parallel sides are equal.
View SolutionIn figure, PQRS is an isosceles trapezium. Find x
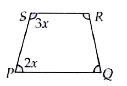 View Solution
View Solution

