ABCD is a rhombus whose side AB=4cmand ∠ABC=120∘, then the length of diagonal BD is equal to :
1 cm
2 cm
3 cm
4 cm
The correct Answer is:D
To find the length of diagonal BD in rhombus ABCD where AB = 4 cm and ∠ABC = 120°, we can follow these steps:
Step 1: Understand the properties of a rhombus
A rhombus has all sides equal and the diagonals bisect each other at right angles. The diagonals also bisect the angles of the rhombus.
Step 2: Draw the rhombus and label the angles
Draw rhombus ABCD with AB = 4 cm. Label the angles:
- ∠ABC = 120°
- Since the diagonals bisect the angles, ∠ABD = ∠ABC / 2 = 120° / 2 = 60°.
Step 3: Find the adjacent angle
The adjacent angle ∠BAD can be found using the property that the sum of adjacent angles in a rhombus is 180°:
- ∠BAD + ∠ABC = 180°
- ∠BAD + 120° = 180°
- ∠BAD = 180° - 120° = 60°.
Step 4: Analyze triangle ABD
Now, we have triangle ABD where:
- ∠ABD = 60°
- ∠BAD = 60°
- Therefore, ∠ADB = 180° - (60° + 60°) = 60°.
Step 5: Conclude that triangle ABD is equilateral
Since all angles in triangle ABD are 60°, triangle ABD is an equilateral triangle. In an equilateral triangle, all sides are equal.
Step 6: Find the length of diagonal BD
Since triangle ABD is equilateral:
- AB = AD = BD
- Given AB = 4 cm, we conclude that BD = 4 cm.
Final Answer
The length of diagonal BD is 4 cm.
---
A rhombus has all sides equal and the diagonals bisect each other at right angles. The diagonals also bisect the angles of the rhombus.
Draw rhombus ABCD with AB = 4 cm. Label the angles:
- ∠ABC = 120°
- Since the diagonals bisect the angles, ∠ABD = ∠ABC / 2 = 120° / 2 = 60°.
The adjacent angle ∠BAD can be found using the property that the sum of adjacent angles in a rhombus is 180°:
- ∠BAD + ∠ABC = 180°
- ∠BAD + 120° = 180°
- ∠BAD = 180° - 120° = 60°.
Now, we have triangle ABD where:
- ∠ABD = 60°
- ∠BAD = 60°
- Therefore, ∠ADB = 180° - (60° + 60°) = 60°.
Since all angles in triangle ABD are 60°, triangle ABD is an equilateral triangle. In an equilateral triangle, all sides are equal.
Since triangle ABD is equilateral:
- AB = AD = BD
- Given AB = 4 cm, we conclude that BD = 4 cm.
The length of diagonal BD is 4 cm.
Topper's Solved these Questions
- BOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XII)90videos
GEOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XIII)70videos
GEOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- X)25videos
GEOMETRY
- BOOK - KIRAN PUBLICATIONCHAPTER - DISCOUNTEXERCISE - Test Yourself10videos
DISCOUNT
- BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself18videos
LCM AND HCF
Similar Questions
Knowledge Check
- Question 1 - Select One
If length of each side of a rhombus ABCD is 16 cm and ∠ABC=120∘ then what is the length ( in cm) of BD ?
A24B12C16D14√3 - Question 2 - Select One
ABCD is a rhombus with ∠ABC=56∘, then ∠ACD is equal to:
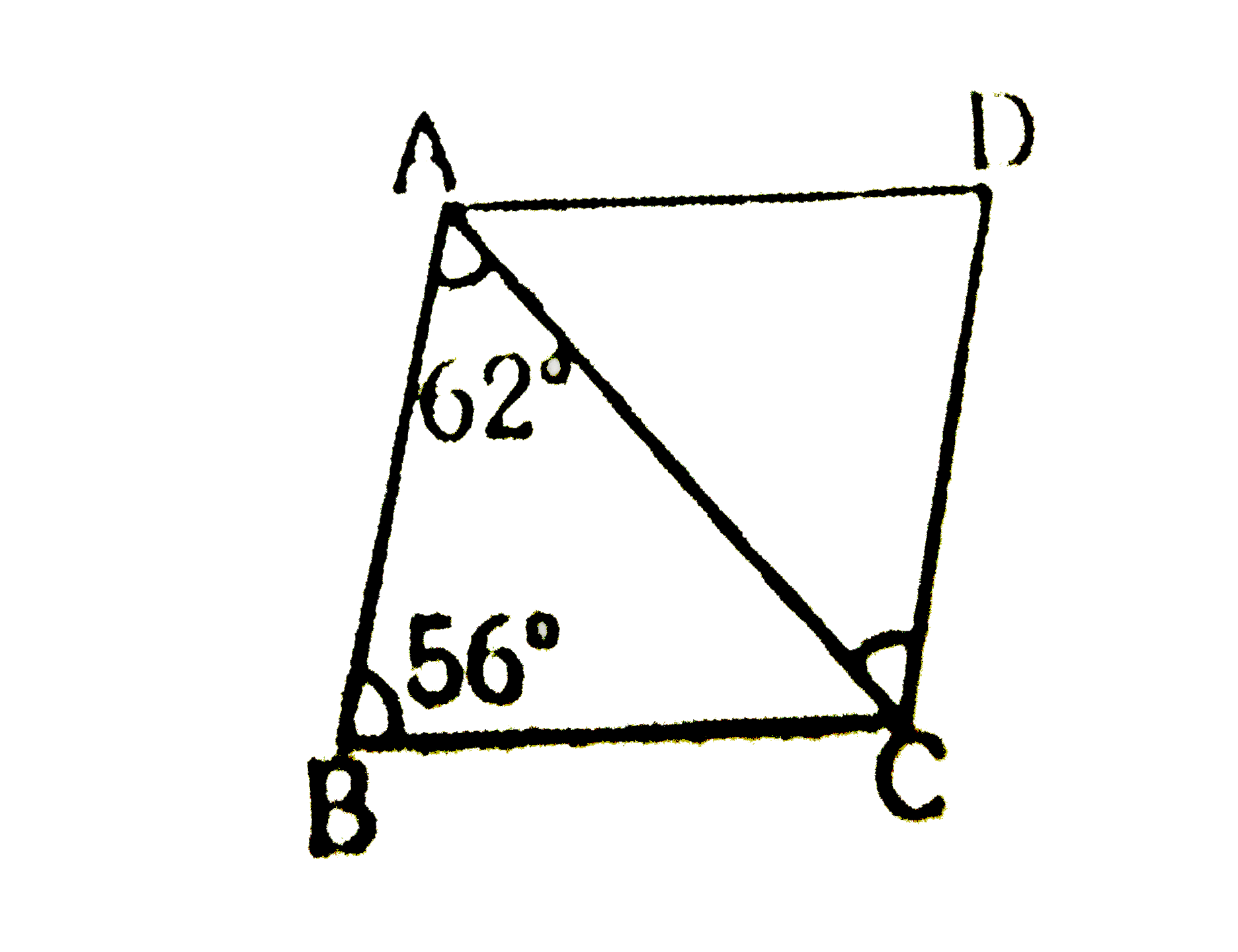 A90∘B60∘C56∘D62∘
A90∘B60∘C56∘D62∘ - Question 3 - Select One
ABCD is a rhombus with side 10 cm and one of its diagonals BD equal to 12 cm. The area of the square constructed on the other diagonal AC as its side is (in cm2)
A144B196C256D400
If ABCD is a rhombus and BO=3 and AO=4cm, then find the length of each side of rhombus.
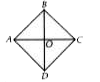 View Solution
View SolutionABCD is a parallelogram with AB and AD as adjacent sides. If ∠A=60∘ and AB = 2AD, then the diagonal BD will be equal to
View SolutionThe diagonals of a rhombus are 8 cm and 6 cm, then the length of each side of the rhombus is
View SolutionABCD is a rhombus with side 6 cm. If one of the diagonals is of 3√3 cm then length of other diagonal is
View SolutionIn a rhombus ABCD, ∠A=60∘, AB = 12cm. Then the diagonal BD is
View Solution

