The internal /external bisector of an angled of a triangle divides the opposite side internally / externally in the ratio of the sides containing the angle .
In the given figure ,Ae is the bisector of the exterior $∠ C A D$ meeting BC produced in E . If AB=15 cm , AC = 8 cm and BC =14 cm , find CE .

A

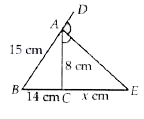
12 cm

B

16 cm

C

20 cm

D

18 cm

Text Solution

Verified by Experts

The correct Answer is:B

|

Updated on:21/07/2023

Class 10 MATHS TRIANGLES

Topper's Solved these Questions

BOOK - MTG IIT JEE FOUNDATIONCHAPTER - TRIANGLES EXERCISE - Exercise (Subjective Problems) (Very Short Answer Type)
10videos
TRIANGLES
BOOK - MTG IIT JEE FOUNDATIONCHAPTER - TRIANGLES EXERCISE - Exercise (Subjective Problems) (Short Answer Type)
10videos
TRIANGLES
BOOK - MTG IIT JEE FOUNDATIONCHAPTER - TRIANGLES EXERCISE - Exercise (Assertion & Reason Type)
5videos
TRIANGLES
BOOK - MTG IIT JEE FOUNDATIONCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - OLYMPIAD/HOTS CORNER
20videos
SURFACE AREAS AND VOLUMES

Similar Questions

Knowledge Check

Question 1 - Select One
In the figure AD is the external bisector of $∠ E A C$ , intersects BC produced to D. IF $A B = 12 c m, A C = 8 c m and B C = 4 c m$ , find CD :

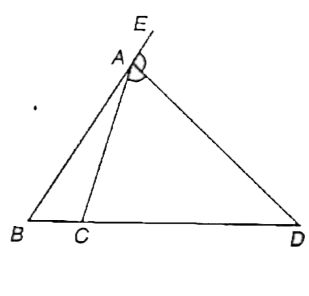A10 cm
B6 cm
C8 cm
D9 cm
Question 2 - Select One
In the given figure , Ad is the bisector of $∠ B A C$ . If AB = 10 cm , AC = 14 cm and BC = 8 cm , find BD and DC respectively .

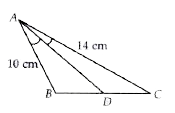A $4.7 c m, 3.3 c m$
B $3.3 c m, 4.7 c m$
C $4.3 c m, 3, 5 c m$
D $3.5 c m, 4.3 c m$

MTG IIT JEE FOUNDATION-TRIANGLES - Exercise (Comprehension Type)

Home
Ask Doubt
Profile