If AD, BE and CF are medians of triangle ABC then prove that median AD divides line segment EF.
To prove that the median AD divides the line segment EF into equal parts, we can follow these steps:
1. Identify the Medians:
Given triangle ABC with medians AD, BE, and CF, we know that:
- D is the midpoint of BC (BD = DC)
- E is the midpoint of AC (AE = EC)
- F is the midpoint of AB (AF = BF)
2. Use the Midpoint Theorem:
Since F and E are midpoints of sides AB and AC respectively, by the Converse of the Midpoint Theorem, we can conclude that line segment EF is parallel to side BC.
3. Consider Triangles AFG and ABD:
In triangles AFG and ABD:
- Angle A is common to both triangles.
- Since EF is parallel to BC, angle F = angle B (corresponding angles).
Therefore, by AA similarity, triangle AFG is similar to triangle ABD.
4. Set Up Ratios from Similar Triangles:
From the similarity of triangles AFG and ABD, we can write the ratio of corresponding sides:
FGBD=AFAB
Since F is the midpoint of AB, we have:
AF=12AB
Thus, the ratio simplifies to:
FGBD=1/2⋅ABAB=12
5. Consider Triangles AGE and ADC:
Similarly, in triangles AGE and ADC:
- Angle A is common.
- Angle E = angle C (corresponding angles).
Therefore, triangle AGE is similar to triangle ADC.
6. Set Up Ratios from Similar Triangles:
From the similarity of triangles AGE and ADC, we can write:
GEDC=AEAC
Since E is the midpoint of AC, we have:
AE=12AC
Thus, the ratio simplifies to:
GEDC=1/2⋅ACAC=12
7. Equate the Ratios:
From the two ratios we derived:
FGBD=12andGEDC=12
Since BD = DC (D is the midpoint of BC), we can equate the two ratios:
FGBD=GEBD
This implies:
FG=GE
8. Conclusion:
Since FG = GE, we have shown that the median AD divides the line segment EF into equal parts.
Given triangle ABC with medians AD, BE, and CF, we know that:
- D is the midpoint of BC (BD = DC)
- E is the midpoint of AC (AE = EC)
- F is the midpoint of AB (AF = BF)
Since F and E are midpoints of sides AB and AC respectively, by the Converse of the Midpoint Theorem, we can conclude that line segment EF is parallel to side BC.
In triangles AFG and ABD:
- Angle A is common to both triangles.
- Since EF is parallel to BC, angle F = angle B (corresponding angles).
Therefore, by AA similarity, triangle AFG is similar to triangle ABD.
From the similarity of triangles AFG and ABD, we can write the ratio of corresponding sides:
FGBD=AFAB
Since F is the midpoint of AB, we have:
AF=12AB
Thus, the ratio simplifies to:
FGBD=1/2⋅ABAB=12
Similarly, in triangles AGE and ADC:
- Angle A is common.
- Angle E = angle C (corresponding angles).
Therefore, triangle AGE is similar to triangle ADC.
From the similarity of triangles AGE and ADC, we can write:
GEDC=AEAC
Since E is the midpoint of AC, we have:
AE=12AC
Thus, the ratio simplifies to:
GEDC=1/2⋅ACAC=12
From the two ratios we derived:
FGBD=12andGEDC=12
Since BD = DC (D is the midpoint of BC), we can equate the two ratios:
FGBD=GEBD
This implies:
FG=GE
Since FG = GE, we have shown that the median AD divides the line segment EF into equal parts.
Topper's Solved these Questions
- CENTRE OF TRIANGLEBOOK - LUCENT PUBLICATIONCHAPTER - CENTRE OF TRIANGLEEXERCISE - EXERCISE-6A
59 Videos
- CENTRE OF TRIANGLEBOOK - LUCENT PUBLICATIONCHAPTER - CENTRE OF TRIANGLEEXERCISE - EXERCISE-6B
8 Videos
- ALGEBRAIC IDENTITIES BOOK - LUCENT PUBLICATIONCHAPTER - ALGEBRAIC IDENTITIES EXERCISE - Exercise - 1B
28 Videos
- CIRCLE AND ITS TANGENT LINESBOOK - LUCENT PUBLICATIONCHAPTER - CIRCLE AND ITS TANGENT LINESEXERCISE - EXERCISE 8B
27 Videos
Similar Questions
Knowledge Check
If AD, BE and CF are medians of ΔABC, then which one of the following statements is correct ?
A(AD+BE+CF)<AB+BC+CABAD+BE+CF>AB+BC+CACAD+BE+CF=AB+BC+CADAD+BE+CF=√2(AB+BC+CA)If AD , BE and CF are medians of ΔABC, then ¯¯¯¯¯¯AD+¯¯¯¯¯¯BE+¯¯¯¯¯¯CF =
A¯¯¯¯¯¯BD+¯¯¯¯¯¯EA+¯¯¯¯¯¯FBB0C¯¯¯¯¯¯AE+¯¯¯¯¯¯DB+¯¯¯¯¯¯FAD¯0Let BE and CF be the two medians of a△ABC and G be the intersection. Also, let EF cut AG at O, then AO : OG is
A1:1B1:2C2:1D3:1
P is a point on the median AD of the triangle ABC. Prove that triangle APB = triangle APC.
View SolutionThe sides AB and BC and the median AD of triangle ABC are equal to the sides PQ and QR and the median PM of triangle PQR respectively. Prove that the triangles ABC and PQR are congruent.
View SolutionAD, BE and CF asre the medians of a triangle ASBC intersectiing in G. Show that △AGB=△BGC=△CGA=13△ABC.
View SolutionIn Figure, AD, and BE are medians of ABC and BE∣∣DF. Prove that CF=14AC.
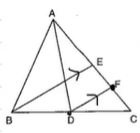
View SolutionLet AD be a median of the ΔABC. If AE and AF are medians of the triangle ABD and ADC, respectively, and AD = m1,AE=m2,AF=m3, then a2/8 is equal to
View Solution
