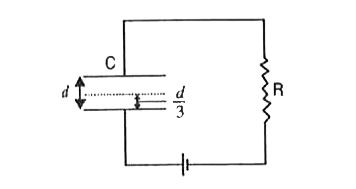
A parallel plate capacitor C with plates of unit area and separation d is filled with a liquid of dielectric constant K = 2 . The level of liquid is d/3 initially . Suppose the liquid level decreases at a constant speed v , the time constant as a function of time is :
6∈0R5d+3vt
(15d+9vt)ε0R2d2−3dvt−9v2t2
6ε0R5d−3vt
(15d−9vt)ε0R2d2−3dvt−9v2t2
The correct Answer is:A
Let the level of liquid at an instant of time .t. be x
Then v=−dxdt⇒dx=−vdt
⇒ ∫xd3dx=v∫tadt
⇒x−d3=−vt
⇒x=d3−vt

Also , the capacitance can be considered as an equivalent of two capacitaces in seies such that
1Ceq=1C1+1C2
⇒ 1Ceq=1∈0Ad−x+1∈0AKx
=d−x∈0−A+x∈0AK
∴ −1Ceq=−1∈0A[d−x1+xK]
=1∈0A[Kd−Kx+xK]
=Kd+x(1−K)∈0AK
∴ Ceq=∈0AKkd+x(1−K)
But A=1 , K = 2
and x=d3−vt
∴ Ceq=∈0×1×22d+[d3−vt](1−2)
Topper's Solved these Questions
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - LEVEL-III
23 Videos
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - RECENT COMPETITIVE QUESTIONS
39 Videos
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - RECENT COMPETITIVE QUESTIONS
39 Videos
- ELECTRONS & PHOTONSBOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - RECENT COMPETITIVE QUESTIONS
20 Videos
- GRAVITATIONBOOK - MODERN PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - Recent Competitive Questions
8 Videos
Similar Questions
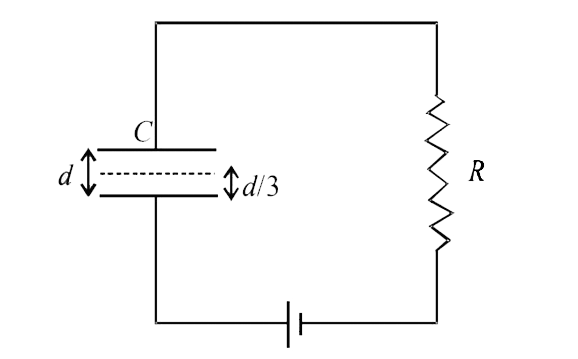
A parallel plate capacitor C with plates of unit area and separation d is filled with a liquid of dielectric constant K=2. The level of liquid is d/3 initially. Suppose the liquid level decreases at a constant speed v, the time constant as a function of time t is-
View SolutionA parallel plate capacitor, with plate area A and plate separation d, is filled with a dielectric slabe as shown. What is the capacitance of the arrangement ?
View Solution
Knowledge Check
A parallel plate capacitor C with plates of unit area and separation d is filled with a liquid of dielectric constant K=2. The level of liquid is d/3 initially. Suppose the liquid level decreases at a constant speed v, the time constant as a function of time t is-
 A(a) 6eπslon0R5d+3vtB(b) (15d+9vt)ε0R2d2−3dvt−9v2t2C(c) 6ε0R5d−3vtD(d) (15d−9vt)ε0R2d2−3dvt−9v2t2
A(a) 6eπslon0R5d+3vtB(b) (15d+9vt)ε0R2d2−3dvt−9v2t2C(c) 6ε0R5d−3vtD(d) (15d−9vt)ε0R2d2−3dvt−9v2t2A capacitor of plate area A and separation d is filled with two dielectrics of dielectric constant K1=6andK2=4. New capacitance will be
 A4Aε0dB4.8Aε0dC5Aε0dD2.4Aε0d
A4Aε0dB4.8Aε0dC5Aε0dD2.4Aε0dA parallel plate capacitor of plate area A and separation d is filled with two materials each of thickness d2 and dielectric constants ∈1 and ∈2 respectively. The equivalent capacitance will be
 A∈0Ad(∈1+∈2)B∈0Ad(∈1+∈2)∈1∈2C2∈0Ad∈1∈2(∈1+∈2)D2∈0Ad(∈1+∈2)∈1∈2
A∈0Ad(∈1+∈2)B∈0Ad(∈1+∈2)∈1∈2C2∈0Ad∈1∈2(∈1+∈2)D2∈0Ad(∈1+∈2)∈1∈2
A capacitor of plate area A and separation d is filled with two dielectrics of dielectric constant K1=6andK2=4. New capacitance will be

View SolutionA parallel plate capacitor with plate area A & plate separation d is filled with a dielectric material of dielectric constant given byK=K0(1+αx).. Calculate capacitance of system: (given αd<<1).

View SolutionA parallel plate capacitor with plate area A & plate separation d is filled with a dielectric material of dielectric constant given byK=K0(1+αx).. Calculate capacitance of system: (given αd<<1).

View SolutionA parallel plate capacitor with plate area A & plate separation d is filled with a dielectric material of dielectric constant given byK=K0(1+αx).. Calculate capacitance of system: (given αd<<1).

View SolutionA parallel plate capacitor with plate area A & plate separation d is filled with a dielectric material of dielectric constant given byK=K0(1+αx).. Calculate capacitance of system: (given αd<<1).

View Solution
