A length-scale (l) depends on the permittivity (ε) of a dielectric material, Boltzmann constant (kB), the absolute temperature (T), the number per unit volume (n) of certain charged particles, and the charge (q) carried by each of the particles. Which of the following expression for l is dimensionally correct?
l=√nq2εkBT
l=√εkBTnq2
l= ⎷q2εn23kBT
l=√q2εkBT
The correct Answer is:B
By the principle of homogeneity, a/V2 is of the dimensions of pressure and b is of the dimensions of volume.
[a] = [pressure] [V2]=[ML−1T−2][L6]=[ML5T−2]
[b] =[V]=L3
Topper's Solved these Questions
- NATURE OF PHYSICAL WORLD AND MEASUREMENTBOOK - NCERT TAMILCHAPTER - NATURE OF PHYSICAL WORLD AND MEASUREMENTEXERCISE - EXERCISE (II. Short Answer Type Questions)
5 Videos
- NATURE OF PHYSICAL WORLD AND MEASUREMENTBOOK - NCERT TAMILCHAPTER - NATURE OF PHYSICAL WORLD AND MEASUREMENTEXERCISE - EXERCISE (III. Long Answer Type Questions)
8 Videos
- NATURE OF PHYSICAL WORLD AND MEASUREMENTBOOK - NCERT TAMILCHAPTER - NATURE OF PHYSICAL WORLD AND MEASUREMENTEXERCISE - EXERCISE (V. Conceptual Questions)
5 Videos
- MOTION OF SYSTEM OF PARTICLES AND RIGID BODIES BOOK - NCERT TAMILCHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODIES EXERCISE - EXERCISE (V. NUMERICAL PROBLEMS)
6 Videos
- OSCILLATIONSBOOK - NCERT TAMILCHAPTER - OSCILLATIONSEXERCISE - EVALUATION(IV. NUMERICAL PROBLEMS)
4 Videos
Similar Questions
The two ends of a uniform rod of thermal conductivity k are maintained at different but constant temperatures. The temperature gradientat any point on the rod is dθdl(equal to the difference in temperature per unit length). The heat flow per unit time per unit cross-section of the rod is l then which of the following statements is/are correct:
View SolutionThe vander waal's equation for a gas is (p+aV2)(V−b)=nRT where p, V, R, T and n represent the Pressure, Volume, universal gas constant, absolute temperature and number of moles of a gas respectively, where a and b are constants. The ratioba will have the following dimensional formula
View Solution
Knowledge Check
A length - scale (l) depends on the permittivity (ε) of a dielctric material. Boltzmann constant (kB), the absolute tempreture (T), the number per unit volume (n) of certain charged particles, and the charge (q) carried by each of the partcles. which of the following expression (s) for I is (are) dimensionally correct?
A(a) l=√(nq2εKBT)B(b)l=√(εKBTnq2)C( c ) l=√(q2εn2/3KBT)D( d ) l=√(q2εn1/3KBT)A conductor of length of l and area of cross section A has n number of electrons per unit volume of the conductor. The total charge carried by the conductor is
AnA/eBenAlCnAleDn⋅eThe two ends of a uniform rod of thermal conductivity k are maintained at different but constant temperatures. The temperature gradientat any point on the rod is dθdl(equal to the difference in temperature per unit length). The heat flow per unit time per unit cross-section of the rod is l then which of the following statements is/are correct:
Adthηdl is the same for all points on the rodBI will decrease as we move from higher to lower temperatureCI∝k.dthηdlDAll the above options are incorrect
A velocity filter uses the properties of electric and magnetic field to select particles that are moving with a specifice velocity. Charged particles with varying speeds are directed into the filter as shown. The filter consistt of an electric field E and a magnetic field B, each of constant magnitude, directed perpendicular to each other as shown, The charge particles will experience a force due to electric field given by F = qE. If positively charged particles are used, this force is towards right.
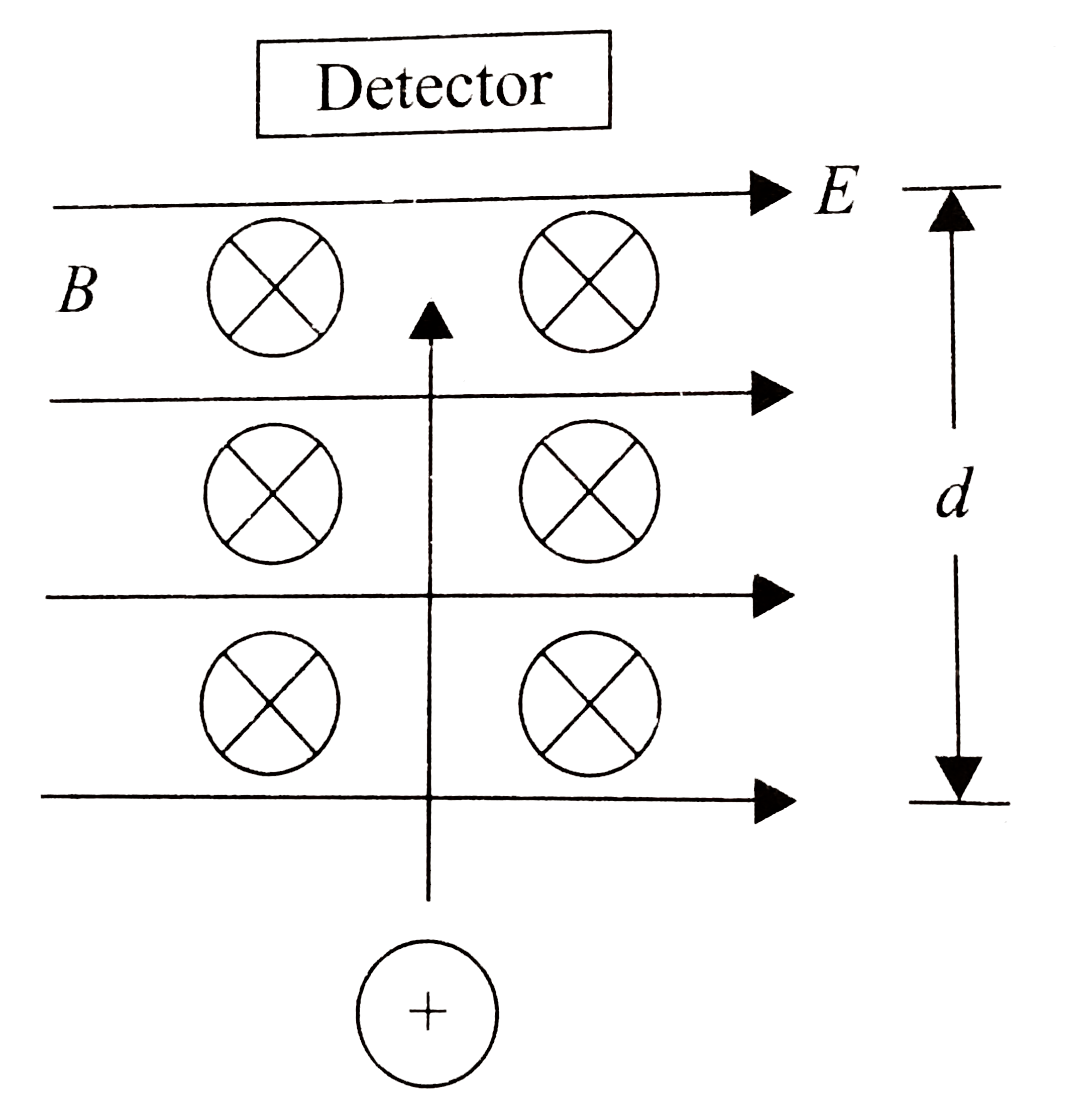
The moving particle will also experience a force due to magnetic field given by F=qvB. When forces due to the two fields are of equal magnitude, the net force on the particle will be zero, the particle will pass through centre with its path unaltered. The electric and magnetic fields can be adjusted t choose the specific velocity to be filtered. The efect of gravity can be neglected.
The electric and magnetic fields are adjusted to detect particles with positive charge q of certain speed v0. which of the following expressions is equal to this speed?
View SolutionThe van der Waal's equation of state for some gases can be expressed as :
(P+aV2)(V−b)=RT
Where P is the pressure , V is the molar volume , and T is the absolute temperature of the given sample of gas and a,b,andR are constants.
Which of the following does not have the same dimensional formula as that for RT?
View SolutionA velocity filter uses the properties of electric and magnetic field to select particles that are moving with a specifice velocity. Charged particles with varying speeds are directed into the filter as shown. The filter consistt of an electric field E and a magnetic field B, each of constant magnitude, directed perpendicular to each other as shown, The charge particles will experience a force due to electric field given by F = qE. If positively charged particles are used, this force is towards right.

The moving particle will also experience a force due to magnetic field given by F=qvB. When forces due to the two fields are of equal magnitude, the net force on the particle will be zero, the particle will pass through centre with its path unaltered. The electric and magnetic fields can be adjusted t choose the specific velocity to be filtered. The efect of gravity can be neglected.
The electric and magnetic fields are adjusted to detect particles with positive charge q of certain speed v0. which of the following expressions is equal to this speed?
View SolutionThe van der Waal's equation of state for some gases can be expressed as :
(P+aV2)(V−b)=RT
Where P is the pressure , V is the molar volume , and T is the absolute temperature of the given sample of gas and a,b,andR are constants.
Which of the following does not have the same dimensional formula as that for RT?
View SolutionThe current in a copper wire is increased by increasing the potential difference between its end. Which one of the following statements regarding n, the number of charge carriers per unit volume in the wire and v the drift velocity of the charge carriers is correct -
View Solution
