In the above problem, minimum value of t for which the intensity at point P on the screen exactly in front of the upper slit becomes maximum.
0.167s
0.5 s
0.012 s
0.3 s
The correct Answer is:A
To solve the problem regarding the minimum value of time t for which the intensity at point P on the screen exactly in front of the upper slit becomes maximum, we need to analyze the conditions for constructive interference in a double-slit experiment.
1. Understanding the Setup:
- In a double-slit experiment, light waves from two slits interfere with each other. The intensity at a point on the screen depends on the path difference between the two waves reaching that point.
2. Condition for Maximum Intensity:
- For maximum intensity (constructive interference) at point P, the path difference Δx between the two waves must be an integer multiple of the wavelength λ:
Δx=nλ(n=0,1,2,…)
3. Path Difference Calculation:
- The path difference can be expressed in terms of the distance traveled by the light from the slits to point P. If the distance from the slits to point P is d and the angle of incidence is θ, then:
Δx=dsinθ
4. Time Calculation:
- The time t taken for light to travel a distance d is given by:
t=dv
where v is the speed of light.
5. Finding Minimum Time:
- To find the minimum time t for which the intensity at point P becomes maximum, we need to ensure that the path difference corresponds to the first order maximum (i.e., n=1):
dsinθ=λ
- Rearranging gives:
d=λsinθ
- Substituting this into the time equation:
t=λsinθv=λvsinθ
6. Conclusion:
- Therefore, the minimum value of time t for which the intensity at point P becomes maximum is:
t=λvsinθ
- In a double-slit experiment, light waves from two slits interfere with each other. The intensity at a point on the screen depends on the path difference between the two waves reaching that point.
- For maximum intensity (constructive interference) at point P, the path difference Δx between the two waves must be an integer multiple of the wavelength λ:
Δx=nλ(n=0,1,2,…)
- The path difference can be expressed in terms of the distance traveled by the light from the slits to point P. If the distance from the slits to point P is d and the angle of incidence is θ, then:
Δx=dsinθ
- The time t taken for light to travel a distance d is given by:
t=dv
where v is the speed of light.
- To find the minimum time t for which the intensity at point P becomes maximum, we need to ensure that the path difference corresponds to the first order maximum (i.e., n=1):
dsinθ=λ
- Rearranging gives:
d=λsinθ
- Substituting this into the time equation:
t=λsinθv=λvsinθ
- Therefore, the minimum value of time t for which the intensity at point P becomes maximum is:
t=λvsinθ
Topper's Solved these Questions
- WAVE OPTICSBOOK - AAKASH SERIESCHAPTER - WAVE OPTICSEXERCISE - Additional Practice Exercise Level - II (Lecture Sheet (Advanced) More Than One Correct Answer Type Questions
4 Videos
- WAVE OPTICSBOOK - AAKASH SERIESCHAPTER - WAVE OPTICSEXERCISE - Additional Practice Exercise Level - II (Lecture Sheet (Advanced) Linked Comprehension Type Questions)
2 Videos
- WAVE OPTICSBOOK - AAKASH SERIESCHAPTER - WAVE OPTICSEXERCISE - Additional Practice Exercise (Level - I) (Main) Straight objective type questions
16 Videos
- WAVE MOTION AND SOUNDBOOK - AAKASH SERIESCHAPTER - WAVE MOTION AND SOUNDEXERCISE - PROBLEMS (LEVEL - II)
97 Videos
- WAVESBOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-III (Doppler effect :)
15 Videos
Similar Questions
In young's double-slit experiment set up, sources S of wavelength 50 nm illumiantes two slits S1 and S2 which act as two coherent sources. The sources S oscillates about its own position according to the equation y=0.5sinπt, where y is in nm and t in seconds. The minimum value of time t for which the intensity at point P on the screen exaclty in front of the upper slit becomes minimum is
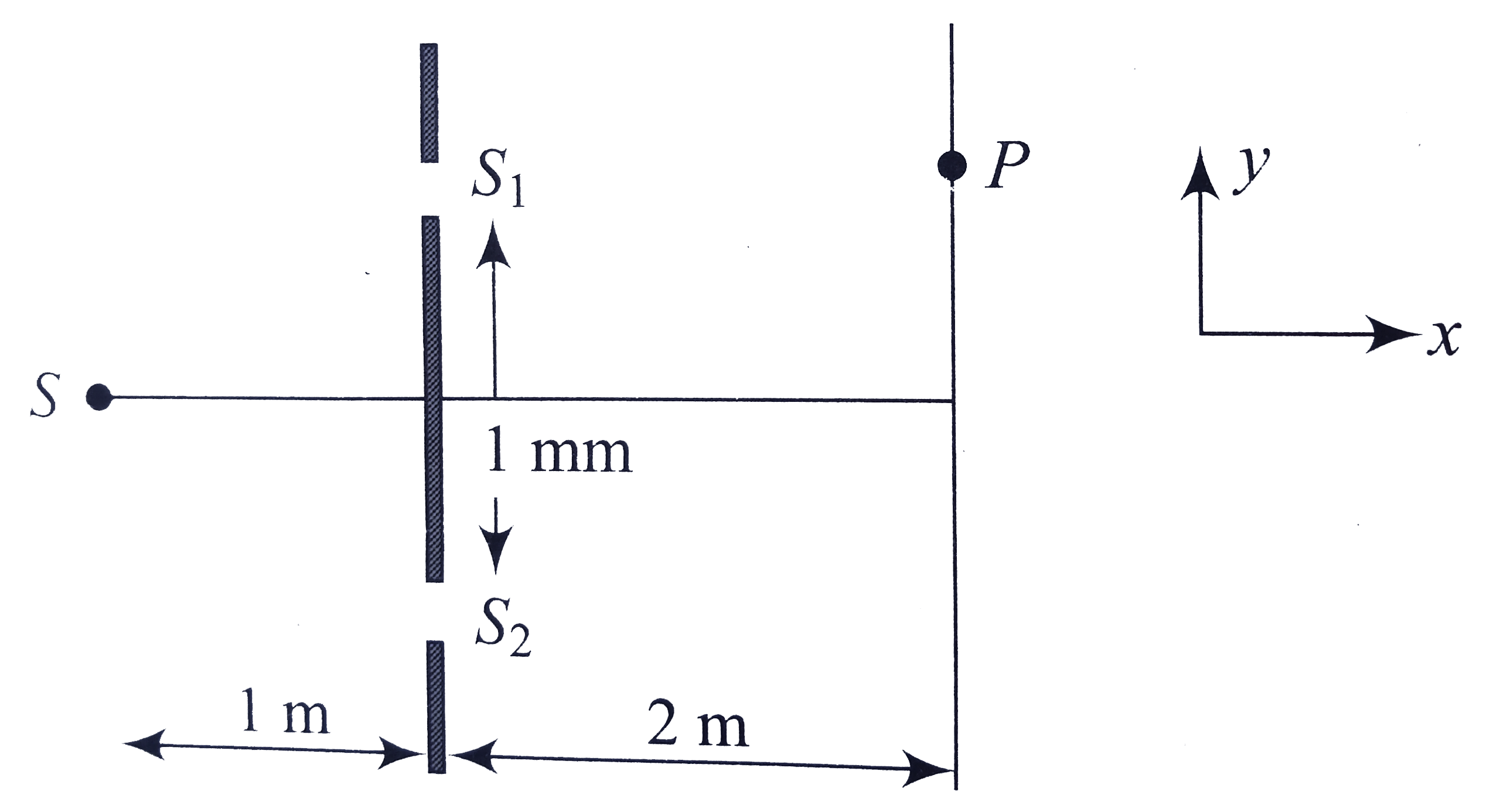
View SolutionIn a YDSE using monochromatic visible light, the distance between the plate of slits and the screen is 1.7 m. At point P on the screen which is directly in front of the upper slit, maximum path is observed. Now, the screen is moved 50 cm closer to the plane of slits. Point P now lies between third and fouth minima above the central maxima and the intensity at P in one-fourth of the maxima intensity on the screen.
Find the value of n.
View SolutionIn a YDSE using monochromatic visible light, the distance between the plate of slits and the screen is 1.7 m. At point P on the screen which is directly in front of the upper slit, maximum path is observed. Now, the screen is moved 50 cm closer to the plane of slits. Point P now lies between third and fouth minima above the central maxima and the intensity at P in one-fourth of the maxima intensity on the screen. ltbr> Find the wavelength of light if the separation of slits is 2 mm.
View SolutionAt a point on the screen directly in front of one of the slits, find the missing wavelengths.
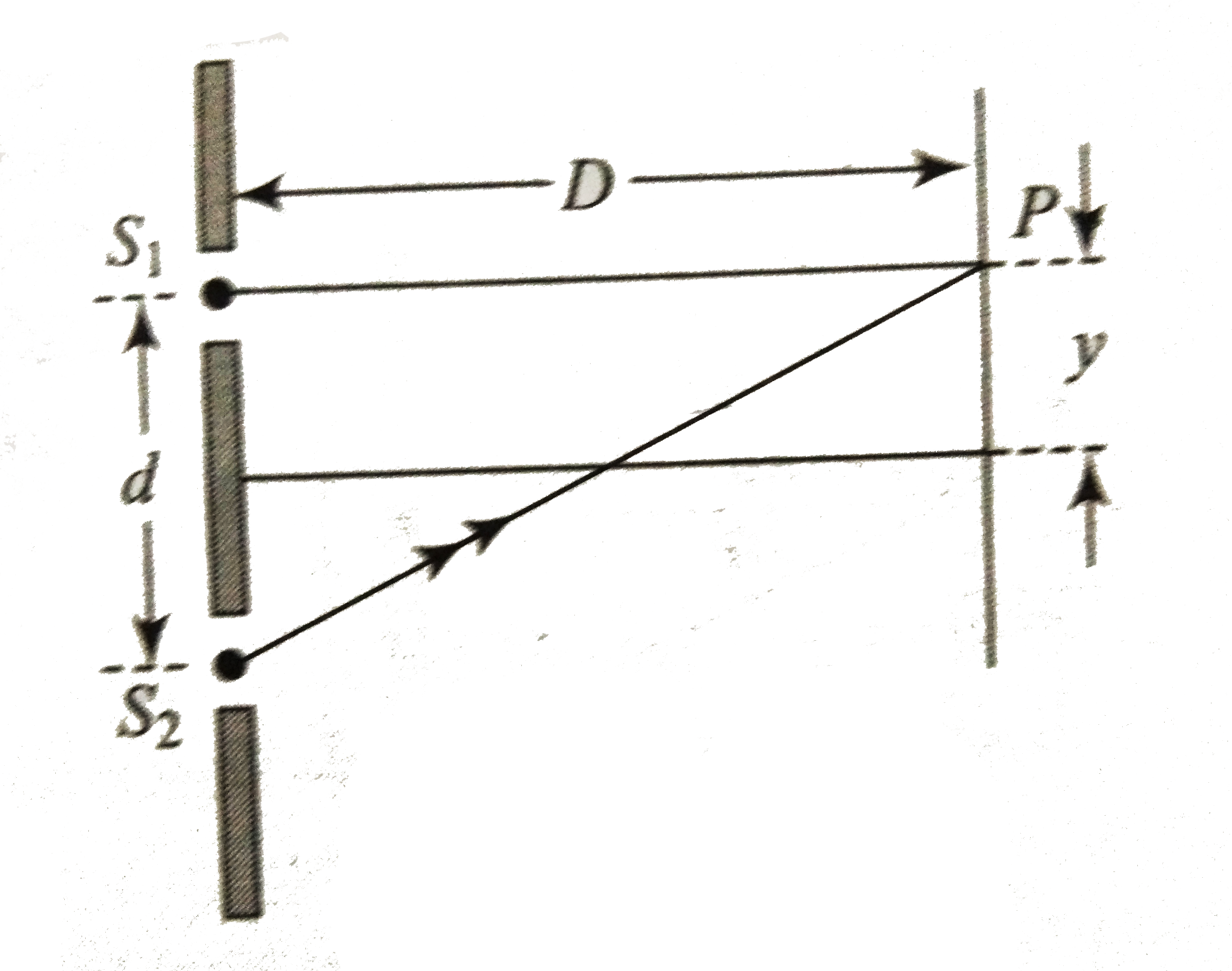
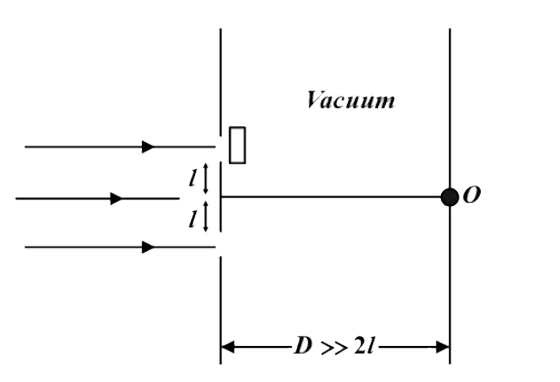
View SolutionIn young's double-slit experiment, both the slits produce equal intensities on a screen. A 100% transparent thin film of refractive index μ=1.5 is kept in front of one of the slits, due to which the intensity at the point O on the screen becomes 75% of its initial value. If the wavelength of monochromatic light is 720 nm, then what is the minimum thickness (in nm) of the film?
View SolutionWhite light is used to illuminate the two slits in a Young's
double slit experiment. The separation between the slits is b and the screen is at
a distance d(>>b) from the slits. At a point on the screen directly in front of
one of the slits, certain wavelengths are missing. Some of these missing
wavelength are
View SolutionWhite light is used to illuminate the two slits in a Young's double slit experiment. The separation between the slits is b and the screen is at a distance d(>>b) from the slits At a point on the screen directly in front of one of the slits, certain wavelengths are missing some of these missing wavelengths are
View SolutionWhite light is used to illuminate the two slits in a Young's double slit experiment. The separation between the slits is b and the screen is at a distance d(>>b) from the slits At a point on the screen directly in front of one of the slits, certain wavelengths are missing some of these missing wavelengths are
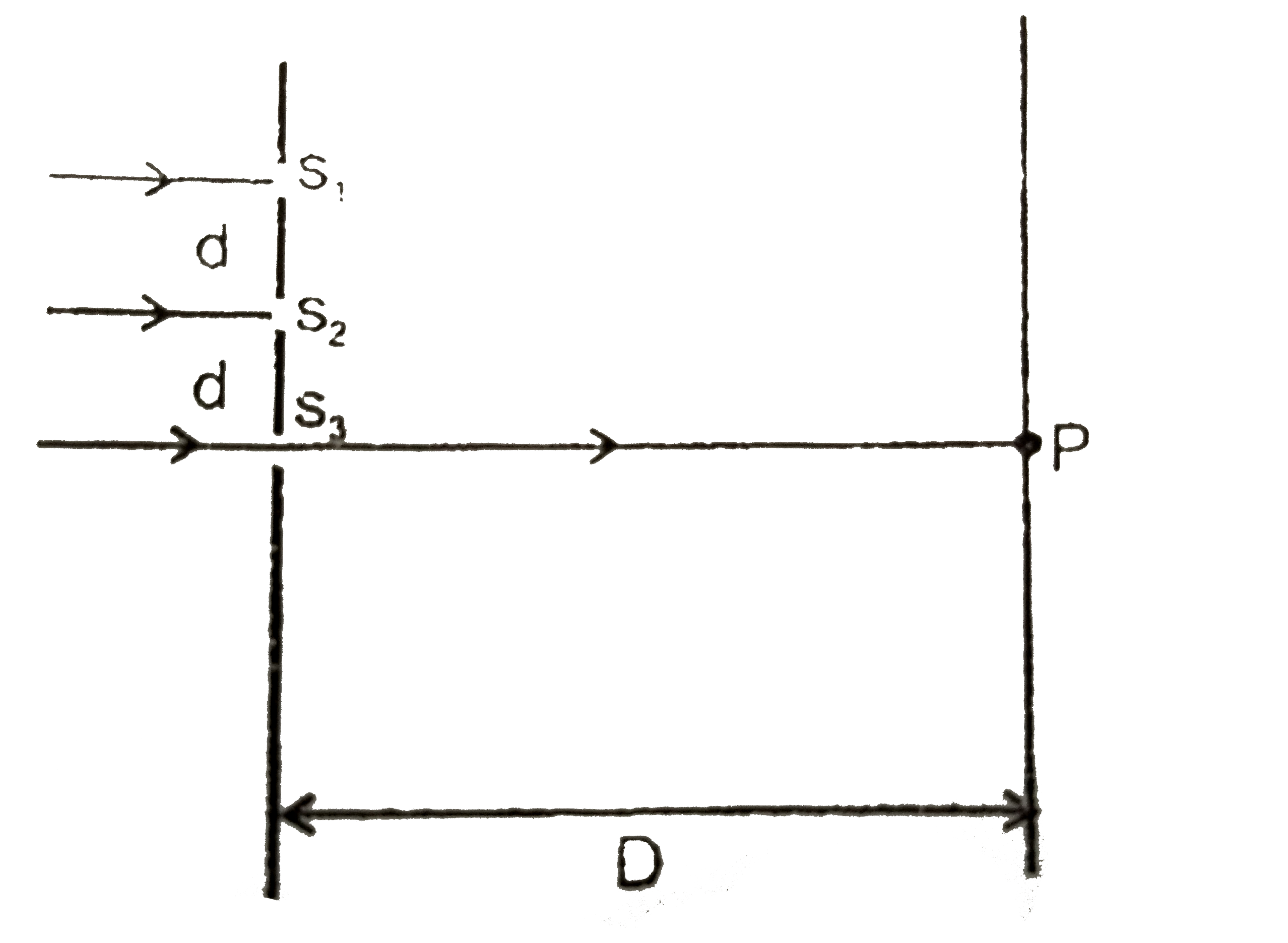
View SolutionIn the figure shown three slits s1,s2 and s3 are illuminated with of wavelength λ.λ<<d and D>>d. Each slit prouduces same intensity I on the screen. If intensity at the point on screen directly infront of s2 is 3I then the maximum value of λ is nd22D Find value of n.
View SolutionThe distance between the tew slits in a Young's double slit experiment is d and the distance of the screen from the plane of the slits is b, P is a point on the screen directly infront of one of the slits. The path difference between the waves arriving at P from the two slits is
View Solution
