If ABCD is a square then show that the points, A,B, C and D are concyclic.
To show that the points A, B, C, and D of a square ABCD are concyclic, we can follow these steps:
1. Understand the Definition of Concyclic Points:
- Points are said to be concyclic if they lie on the same circle.
2. Draw the Square ABCD:
- Visualize or sketch a square ABCD. Label the vertices A, B, C, and D in clockwise order.
3. Identify the Diagonals:
- The diagonals of the square are AC and BD.
4. Show that the Diagonals are Equal:
- In a square, the diagonals are equal in length. Therefore, AC = BD.
5. Show that the Diagonals are Perpendicular:
- The diagonals of a square bisect each other at right angles. This means that they intersect at a right angle (90 degrees).
6. Find the Center of the Square:
- Let O be the point where the diagonals intersect. This point O is the center of the square.
7. Determine the Distance from O to Each Vertex:
- Since O is the midpoint of both diagonals, the distance from O to A (OA), O to B (OB), O to C (OC), and O to D (OD) is the same. Let this distance be r.
8. Conclude that A, B, C, and D are Equidistant from O:
- Since OA = OB = OC = OD = r, all four points A, B, C, and D are equidistant from the point O.
9. Draw the Circle:
- A circle can be drawn with center O and radius r that passes through points A, B, C, and D.
10. Conclude that A, B, C, and D are Concyclic:
- Since all four points lie on the same circle, we conclude that points A, B, C, and D are concyclic.
- Points are said to be concyclic if they lie on the same circle.
- Visualize or sketch a square ABCD. Label the vertices A, B, C, and D in clockwise order.
- The diagonals of the square are AC and BD.
- In a square, the diagonals are equal in length. Therefore, AC = BD.
- The diagonals of a square bisect each other at right angles. This means that they intersect at a right angle (90 degrees).
- Let O be the point where the diagonals intersect. This point O is the center of the square.
- Since O is the midpoint of both diagonals, the distance from O to A (OA), O to B (OB), O to C (OC), and O to D (OD) is the same. Let this distance be r.
- Since OA = OB = OC = OD = r, all four points A, B, C, and D are equidistant from the point O.
- A circle can be drawn with center O and radius r that passes through points A, B, C, and D.
- Since all four points lie on the same circle, we conclude that points A, B, C, and D are concyclic.
Similar Questions
In Figure, ABC is a triangle in which AB=AC. Point DandE are points on the sides ABandAC respectively such that AD=AE. Show that the points B,C,EandD are concyclic.
View SolutionIf BM and CN are the perpendiculars drawn on the sides AC and BC of the ΔABC, prove that the points B, C, M and N are concyclic.
View Solution
Knowledge Check
In the rectangular coordinate system shown, ABCD is a parallogram. If the coordinates of the points A,B,C and D are (0,2),(a,b),(a,2) and (0,0), respectively, then b =
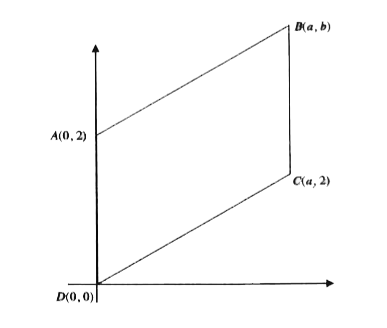 A0B2C3D4
A0B2C3D4
Statement 1 :If the lines 2x+3y+19=0 and 9x+6y−17=0 cut the x-axis at A,B and the y-axis at C,D, then the points, A,B,C,D are concyclic. Statement 2 : Since OAxOB=OCxOD, where O is the origin, A,B,C,D are concyclic.
View SolutionABC is an isosceles triangle in which AB=AC. If D and E are the mid-points of AB and AC respectively, Prove that the points B, C, D and E are concyclic.
View SolutionThere are five points A,B,C,D and E. no three points are collinear and no four are concyclic. If the line AB intersects of the circles drawn through the five points. The number of points of intersection on the line apart from A and B is
View SolutionABCD is a parallelogram. A circle through A, B is so drawn that it intersects AD at P and BC at Q. Prove that P, Q, C and D are concyclic.
View SolutionIf A, B, C and D are four points such that ∠BAC=45∘and∠BDC=45∘, then A, B, C and D are concyclic.
View SolutionC1:x2+y2=r2andC2:x216+y29=1 interset at four distinct points A,B,C, and D. Their common tangents form a peaallelogram A'B'C'D'.
If A'B'C'D' is a square, then the ratio of the area of circle C1 to the area of circumcircle of ΔA'B'C' is
View SolutionIn a square ABCD, show that AC2=2AB2.
View Solution
