Forces acting on a particle moving in a straight line varies with the velocity of the particle as F=αυ where α is constant. The work done by this force in time interval Δt is :
The correct Answer is:C
To find the work done by the force acting on a particle moving in a straight line, where the force varies with the velocity of the particle as F=αv, we can follow these steps:
1. Identify the Relationship Between Force and Power:
The power P delivered by a force F acting on a particle moving with velocity v is given by:
P=F⋅v
2. Substitute the Expression for Force:
Substitute F=αv into the power equation:
P=(αv)⋅v=α
This shows that the power is constant and equal to α.
3. Relate Power to Work Done:
The power can also be expressed as the rate of change of work done W:
P=dWdt
Therefore, we can equate the two expressions for power:
dWdt=α
4. Integrate to Find Work Done:
To find the total work done W over a time interval Δt, we integrate both sides:
W=∫Δt0αdt
5. Evaluate the Integral:
The integral of a constant α over the time interval from 0 to Δt is:
W=α∫Δt0dt=α(t)Δt0=α(Δt−0)=αΔt
6. Final Result:
Thus, the work done by the force in the time interval Δt is:
W=αΔt
The power P delivered by a force F acting on a particle moving with velocity v is given by:
P=F⋅v
Substitute F=αv into the power equation:
P=(αv)⋅v=α
This shows that the power is constant and equal to α.
The power can also be expressed as the rate of change of work done W:
P=dWdt
Therefore, we can equate the two expressions for power:
dWdt=α
To find the total work done W over a time interval Δt, we integrate both sides:
W=∫Δt0αdt
The integral of a constant α over the time interval from 0 to Δt is:
W=α∫Δt0dt=α(t)Δt0=α(Δt−0)=αΔt
Thus, the work done by the force in the time interval Δt is:
W=αΔt
Similar Questions
Force acting on a particle of mass m moving in straight line varies with the velocity of the particle as F=K/V K is constant then speed of the particle in time t
View SolutionKinetic energy of a particle moving in a straight line varies with time t as K=4t2. The force acting on the particle
View SolutionThe power supplied by a force acting on a particle moving in a straight line is constant. The velocity of the particle varies with the displacement x as :
View SolutionIf average velocity of particle moving on a straight line is zero in a time interval, then
View SolutionThe velocity-time graph of a particle moving in a straight line is shown in figure. The mass of the particle is 2kg. Work done by all the forces acting on the particle in time interval between t=0 to t=10s is
 View Solution
View SolutionThe force F acting on a particle is moving in a straight line as shown in figure. What is the work done by the force on the particle in the 4 m of the trajectory?
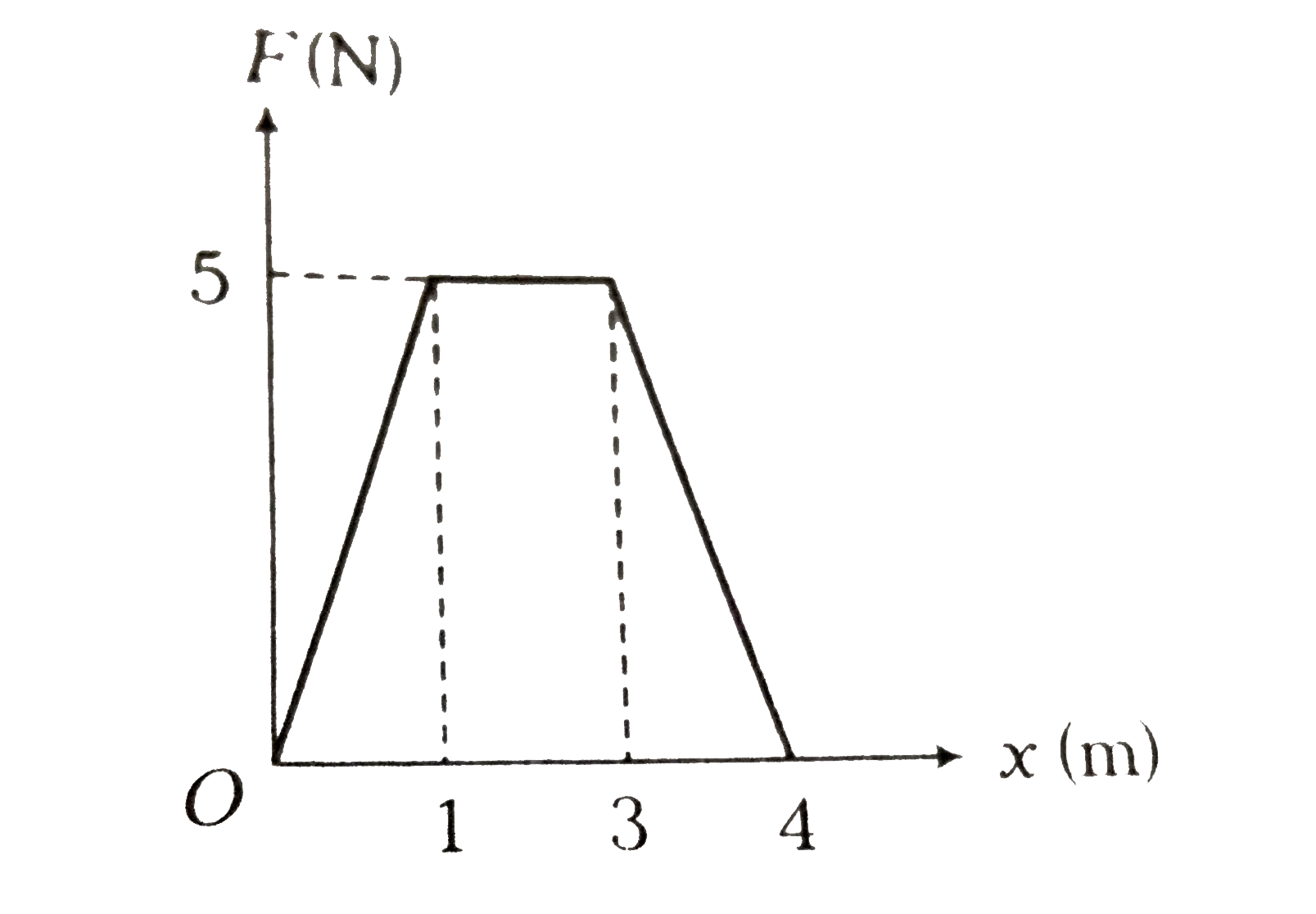 View Solution
View SolutionForce F on a particle moving in a straight line varies with distance d as shown in the figure. The work done on the particle during its displacement of 12m is
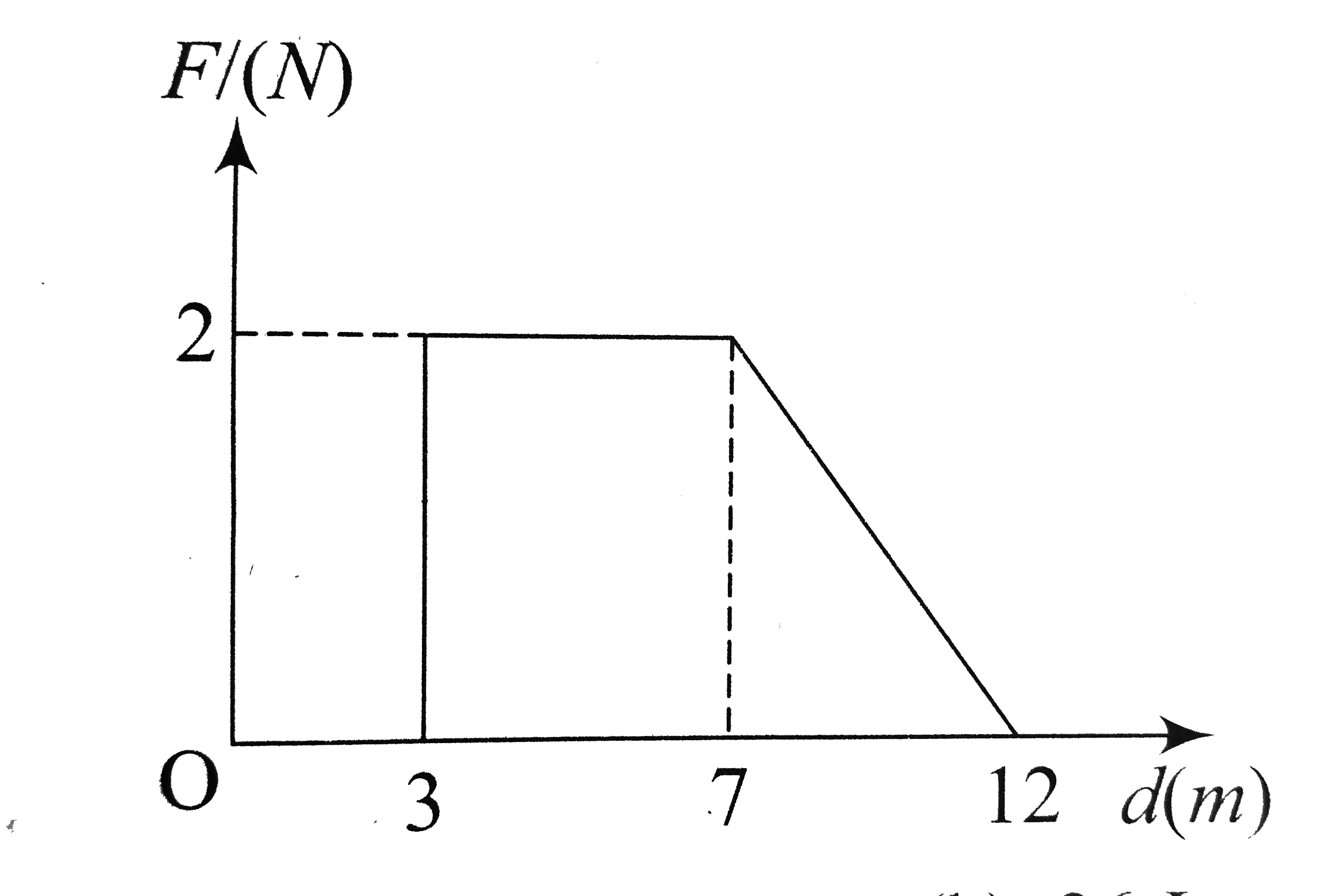 View Solution
View SolutionThe power supplied by a force acting on a particle moving in a straight line is constant. The velocity of the particle varies with displacement as x1K. Find the value of K.
View SolutionKinetic energy of a particle moving in a straight line is proportional to the time t. The magnitude of the force acting on the particle is :
View SolutionThe force acting on a particle moving along a straight line varies with time as shown in diagram.
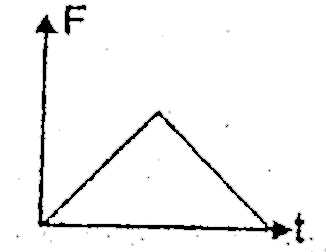
Which of the following graphs is best representative of its speed and time graphs. Initial velocity the particle is zero.View Solution

