A particle of mass 'm' is attached to one end of a string of length 'l' while the other end is fixed to a point 'h' above the horizontal table, the particle is made to revolve in a circle on the table, so as to make P revolution per sec. The maximum value of P if the particle is to be contact with the table is
2π√gh
√gh
2π√hg
12π√gh
The correct Answer is:D
To solve the problem, we need to analyze the forces acting on the particle as it revolves in a horizontal circle while being attached to a string fixed at a height above the table. Here's a step-by-step breakdown of the solution:
Step 1: Understand the Setup
We have a particle of mass m attached to a string of length l. The other end of the string is fixed at a height h above the horizontal table. The particle revolves in a horizontal circle, and we need to find the maximum frequency P (in revolutions per second) at which the particle can revolve while remaining in contact with the table.
Step 2: Identify Forces Acting on the Particle
When the particle is in circular motion, the following forces are acting on it:
1. Tension (T) in the string, acting towards the center of the circle.
2. Weight (mg) of the particle, acting downwards.
3. Normal force (N) from the table, acting upwards.
Step 3: Analyze the Forces
For the particle to remain in contact with the table, the normal force N must be zero at the maximum frequency. Thus, we can set up the following equations based on the forces:
1. In the vertical direction:
Tcosθ=mg
where θ is the angle between the string and the vertical.
2. In the horizontal direction (providing the centripetal force):
Tsinθ=mv2r
where r is the horizontal radius of the circular path.
Step 4: Relate θ to h and l
Using the geometry of the situation:
sinθ=rlandcosθ=hl
From the right triangle formed by the string, height, and radius, we can express r as:
r=√l2−h2
Step 5: Substitute T from Vertical Force Equation
From the vertical force equation, we can express the tension T:
T=mgcosθ=mglh
Step 6: Substitute T into the Horizontal Force Equation
Now substituting T into the horizontal force equation:
mglhsinθ=mv2r
Substituting sinθ=rl:
mglh⋅rl=mv2r
This simplifies to:
mgrh=mv2r
Cancelling m and rearranging gives:
v2=gr2h
Step 7: Relate Linear Velocity to Angular Velocity
The linear velocity v is related to the angular velocity ω by:
v=rω
Substituting this into the equation gives:
(rω)2=gr2h
This simplifies to:
ω2=gh
Step 8: Convert Angular Velocity to Frequency
The frequency P in revolutions per second is related to the angular velocity ω by:
P=ω2π
Substituting ω=√gh:
P=12π√gh
Final Answer
Thus, the maximum value of P is:
P=12π√gh
We have a particle of mass m attached to a string of length l. The other end of the string is fixed at a height h above the horizontal table. The particle revolves in a horizontal circle, and we need to find the maximum frequency P (in revolutions per second) at which the particle can revolve while remaining in contact with the table.
When the particle is in circular motion, the following forces are acting on it:
1. Tension (T) in the string, acting towards the center of the circle.
2. Weight (mg) of the particle, acting downwards.
3. Normal force (N) from the table, acting upwards.
For the particle to remain in contact with the table, the normal force N must be zero at the maximum frequency. Thus, we can set up the following equations based on the forces:
Tcosθ=mg
where θ is the angle between the string and the vertical.
Tsinθ=mv2r
where r is the horizontal radius of the circular path.
Using the geometry of the situation:
sinθ=rlandcosθ=hl
From the right triangle formed by the string, height, and radius, we can express r as:
r=√l2−h2
From the vertical force equation, we can express the tension T:
T=mgcosθ=mglh
Now substituting T into the horizontal force equation:
mglhsinθ=mv2r
Substituting sinθ=rl:
mglh⋅rl=mv2r
This simplifies to:
mgrh=mv2r
Cancelling m and rearranging gives:
v2=gr2h
The linear velocity v is related to the angular velocity ω by:
v=rω
Substituting this into the equation gives:
(rω)2=gr2h
This simplifies to:
ω2=gh
The frequency P in revolutions per second is related to the angular velocity ω by:
P=ω2π
Substituting ω=√gh:
P=12π√gh
Thus, the maximum value of P is:
P=12π√gh
Similar Questions
A particle of maas m is attched to a light string of length l, the other end of which is fixed. Initially the string is kept horizontal and the particle is given an upwrd velocity v. The particle is just able to complete a circle
View SolutionA particle of mass 200g is attached to an ideal string of length 1.30 m whose upper end is fixed to ceiling. The particle is made to revolve in a horizontal circle of radius 50 cm. The tension in the string is (g=10ms2)
View SolutionA particle of mass m1 is fastened to one end of a string and one of m2 to the middle point, the other end of the string being fastened to a fixed point on a smooth horizontal table The particles are then projected, so that the two portions of the string are always in the same straight line and describes horizontal circles find the ratio of tensions in the two parts of the string

View SolutionA block of mass 1kg is tied to a string of length 1m the other end of which is fixed The block is moved on a smooth horizontal table with constant speed 10ms−1. Find the tension in the string
View SolutionA ball of mass m is attached to one end of a light rod of length l, the other end of which is hinged. What minimum velocity v should be imparted to the ball downwards, so that it can complete the circle ?

View SolutionA particle of mass m is fixed to one end of a light rigid rod of length l and rotated in a vertical ciorcular path about its other end. The minimum speed of the particle at its highest point must be
View SolutionA particle of mass 200g is attached to an ideal string of length 1.30 m whose upper end is fixed to ceiling. The particle is made to revolve in a horizontal circle of radius 50 cm. The tension in the string is (g=10ms2)
View SolutionA particle of mass m and charge q is fastened to one end of a string of length. The other end of the string is fixed to the point O. The whole sytem liles on as frictionless horizontal plane. Initially, the mass is at rest at A. A uniform electric field in the direction shown in then switfched on. Then
View SolutionA particle of mass m and charge q is fastened to one end of a string of length. The other end of the string is fixed to the point O. The whole sytem liles on as frictionless horizontal plane. Initially, the mass is at rest at A. A uniform electric field in the direction shown in then switfched on. Then

View SolutionA particle of mass m attached to the end of string of length l is released from the horizontal position. The particle rotates in a circle about O as shown When it is vertically below O, the string makes contact with a nail N placed directly below O at a distance h and rotates around it. For the particle to swing completely around the nail in a circle.
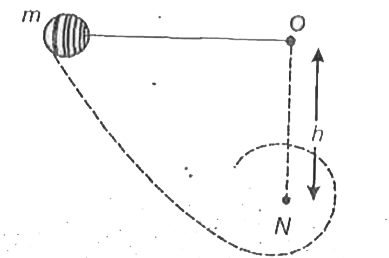
View Solution
