Two small-sized identical equally charged spheres, each having mass 1 mg are hanging in equilibrium as shown in the figure. The length of each string is 10 cm and the angle θ is 7° with the vertical. Calculate the magnitude of the charge in each sphere.
(Take g=10 ms−2 )
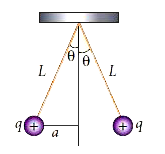
If the two spheres are neutral, the angle between them will be 0∘ when hanged vertically. Since they are positively charged spheres, there will be a repulsive force between them and they will be at equilibrium with each other at an angle of 7° with the vertical. At equilibrium, each charge experiences zero net force in each direction. We can draw a free body diagram for one of the charged spheres and apply Newton’s second law for both vertical and horizontal directions.
The free body diagram is shown below.
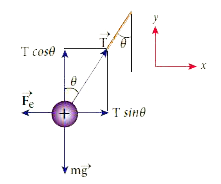
In the x-direction, the acceleration of the charged sphere is zero.
Using Newton’s second law (→Ftot=m→a), we have
Tsinθˆi−Feˆi=0
Tsinθ=Fe (1)
Here T is the tension acting on the charge due to the string and Fe is the electrostatic force between the two charges.
In the y-direction also, the net acceleration experienced by the charge is zero.
Tcosθˆj−mgˆj=0
Therefore, Tcosθ=mg (2)
By dividing equation (1) by equation (2),
tanθ=Femg (3)
Since they are equally charged, the magnitude of the electrostatic force is
Fe=kq2r2 where k=14πε0
Here r=2a=2Lsinθ. By substituting these values in equation (3),
tanθ=kq2mg(2Lsinθ)2 (4)
Rearranging the equation (4) to get q
q=2Lsinθ√mgtanθk
=2×0.1×sin7∘×√10−3×10×tan7∘9×109
q=8.9×10−9C=8.9nC
Topper's Solved these Questions
- ELECTROSTATICSBook:NCERT TAMILChapter:ELECTROSTATICSExercise:EVALUATION (Multiple choice questions)
Explore 15 Videos
- ELECTROSTATICSBook:NCERT TAMILChapter:ELECTROSTATICSExercise:Exercises
Explore 23 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:NCERT TAMILChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:ADDITIONAL EXERCISES
Explore 1 Video
- MAGNETISM AND MAGNETIC EFFECTS OF ELECTRIC CURRENTBook:NCERT TAMILChapter:MAGNETISM AND MAGNETIC EFFECTS OF ELECTRIC CURRENTExercise:EVALUATION (IV. Numerical problems)
Explore 10 Videos
Similar Questions
Two identical small charged spheres, each having a mass m, hang in equilibrium as shown in fig. the ligth of each string is l, and the angle made by any string with the vertical is θ. Find the magnitude of the charge on each sphere.
View SolutionTwo identical, small, charged spheres, each having a mass of 4.0×10−2kg hang in equilibrium as shown in Fig. 22-10a. The length of each string is 1.5m and the angle θ is 37.0∘. Find the magnitude of the charge on each sphere.
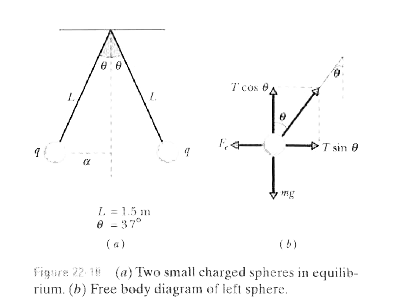
View SolutionTwo identical small charged spheres, each having a mass m, hang in equilibrium the length of each string is l, and the angle made by any string with the vertical is θ. Find the magnitude of the charge on each sphere.
View SolutionTwo identical helium filled balloons A and B fastened to a weight of 5 g by threads floats in equilibrium as shown in figure. Calculate the charge on each balloons, assuming that they carry equal charge.
View SolutionTwo identical helium filled balloons A and B fastened to a weight of 5 g by threads floats in equilibrium as shown in figure. Calculate the charge on each balloons, assuming that they carry equal charge.
View SolutionThree identically charged, small spheres each of mass m are suspended from a common point by insulated light strings each of length I. The spheres are always on vertices of an equilateral triangle of length of the sides x(<<l). Calculate the rate dq/ dt with which charge on each sphere increases if length of the sides of the equilateral triangle increases slowly according to law dxdt=a√x
View SolutionTwo small spheres, each having a mass of 20 g, are suspended form a common point by two insulating strings of length 40 cm each. The spheres are identically charged and the speration between the balls at equilibrium is found to be 4 cm . Find the charge on each sphere.
View SolutionTwo small spheres, each having a mass of 20 g, are suspended form a common point by two insulating strings of length 40 cm each. The spheres are identically charged and the speration between the balls at equilibrium is found to be 4 cm . Find the charge on each sphere.
View SolutionTwo small spheres each of mass m are suspended from a common point by threads 0.5 m long. They are equally charged and repel each other to a distance of 0.28m. If =g10ms−2, what is the charge on each sphere?
View SolutionTwo identical charges repel each other with a force equal to 1mg wt when they are 0.6m apart in air.The value of each charge is (g=10ms−2 )
View Solution
