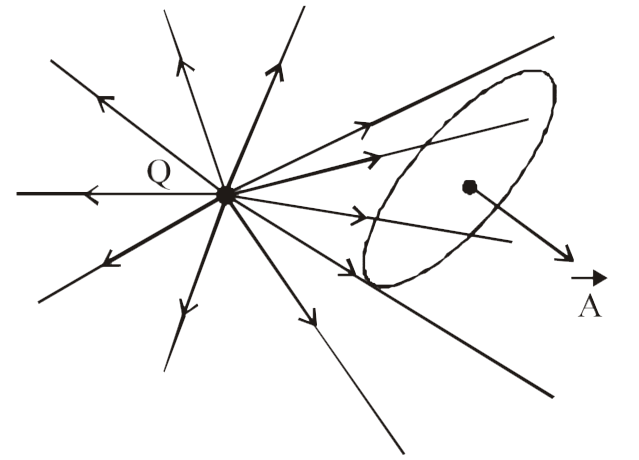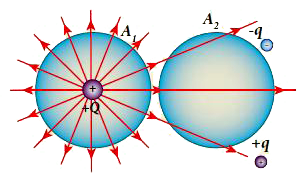
In figure , calculate the electric flux through the closed areas A1andA2.
In figure (a), the area A1 encloses the charge Q. So electric flux through this closed surface A1 is Qεo. But the closed surface A2 contains no charges inside, so electric flux through A2 is zero.
Topper's Solved these Questions
- ELECTROSTATICSBook:NCERT TAMILChapter:ELECTROSTATICSExercise:EVALUATION (Multiple choice questions)
Explore 15 Videos
- ELECTROSTATICSBook:NCERT TAMILChapter:ELECTROSTATICSExercise:Exercises
Explore 23 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:NCERT TAMILChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:ADDITIONAL EXERCISES
Explore 1 Video
- MAGNETISM AND MAGNETIC EFFECTS OF ELECTRIC CURRENTBook:NCERT TAMILChapter:MAGNETISM AND MAGNETIC EFFECTS OF ELECTRIC CURRENTExercise:EVALUATION (IV. Numerical problems)
Explore 10 Videos
Similar Questions
Knowledge Check
The total electric flux ϕ through the entire area of a closed surface is given by
Aϕ=∮→E×→dsBϕ=∮→E⋅→dsCboth 'a' and 'b'Dnone of theseThe electric flux through a closed surface area S enclosing charge Q is ϕ If the surface area is doubled, then the flux is
A2ϕBϕ/2Cϕ/4DϕThe capacitance of the capacitors of plate areas A1 and A2(A1<A2) at a distance d is
 Aε0A1dBε0A2dCε0(A1+A2)2dDε0√A1A2d
Aε0A1dBε0A2dCε0(A1+A2)2dDε0√A1A2d
If A1 is the area of the parabola y2=4ax lying between vertex and the latusrectum and A2 is the area between the latusrectum and the double ordinate x=2a, then A1A2 is equal to
View SolutionIf the equation of the locus of a point equidistant from the points (a1,b1) and (a2,b2) is (a1−a2)x+(b1−b2)y+c=0 , then the value of c is aa2−a22+b12−b22 √a12+b12−a22−b22 12(a12+a22+b12+b22) 12(a22+b22−a12−b12)
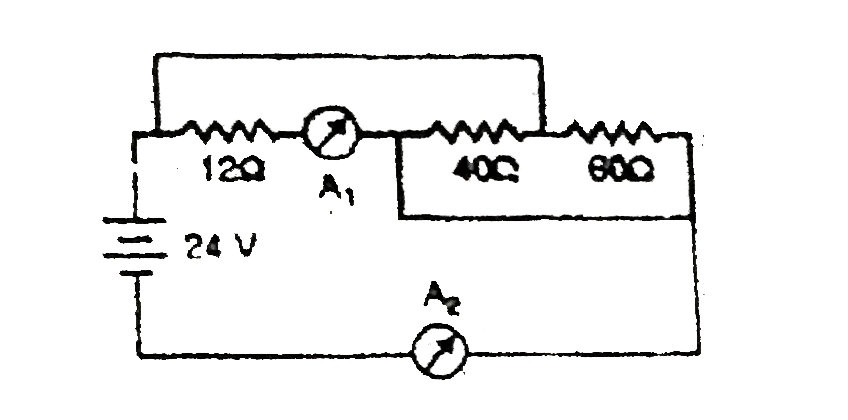
View SolutionIn the circuit of figure A1 and A2 are ideal ammeters. Then the reading in ammeter A2 is
View SolutionIf the area of the circle is A1 and the area of the regular pentagon inscribed in the circle is A2, then find the ratio A1A2.
View SolutionIn the circuit of figure A1 and A2 are ideal ammeters. Then the reading in ammeter A2 is

View Solution