A gas expands from V1 to V2 along three different processes i.e. isothermal, adiabatic and isobaric. If W1, W2, W3 are the works done resp. in the mentioned processes, then which of the following will be the correct order?
W3>W1>W2
W3>W2>W1
W2>W1>W3
W1>W3>W2
To determine the correct order of work done during the expansion of a gas from V1 to V2 along three different processes (isothermal, adiabatic, and isobaric), we can analyze each process step by step.
Step 1: Understand the Work Done in Each Process
1. Isothermal Process:
- In an isothermal process, the temperature remains constant. For an ideal gas, the work done W1 during isothermal expansion can be calculated using the formula:
W1=nRTln(V2V1)
- Here, n is the number of moles, R is the universal gas constant, and T is the absolute temperature.
2. Adiabatic Process:
- In an adiabatic process, there is no heat exchange with the surroundings. The work done W2 can be expressed as:
W2=P1V1−P2V2γ−1
- Where γ is the heat capacity ratio Cp/Cv. The work done in adiabatic expansion is less than that in isothermal expansion because some energy is used to do work against the internal energy change.
3. Isobaric Process:
- In an isobaric process, the pressure remains constant. The work done W3 is given by:
W3=P(V2−V1)
- Since pressure is constant, the work done is directly proportional to the change in volume.
Step 2: Compare the Work Done
Now, we need to compare W1, W2, and W3:
- Isothermal Work W1: The work done is significant because the gas expands at a constant temperature, allowing for maximum energy transfer.
- Adiabatic Work W2: The work done is less than in the isothermal case because some energy is used to change the internal energy of the gas.
- Isobaric Work W3: The work done is generally less than the isothermal work but can be more than the adiabatic work depending on the specific conditions.
Conclusion: Order of Work Done
From the analysis, we can conclude that:
W1>W3>W2
Thus, the correct order of work done in the three processes is:
W1>W3>W2
- In an isothermal process, the temperature remains constant. For an ideal gas, the work done W1 during isothermal expansion can be calculated using the formula:
W1=nRTln(V2V1)
- Here, n is the number of moles, R is the universal gas constant, and T is the absolute temperature.
- In an adiabatic process, there is no heat exchange with the surroundings. The work done W2 can be expressed as:
W2=P1V1−P2V2γ−1
- Where γ is the heat capacity ratio Cp/Cv. The work done in adiabatic expansion is less than that in isothermal expansion because some energy is used to do work against the internal energy change.
- In an isobaric process, the pressure remains constant. The work done W3 is given by:
W3=P(V2−V1)
- Since pressure is constant, the work done is directly proportional to the change in volume.
- Adiabatic Work W2: The work done is less than in the isothermal case because some energy is used to change the internal energy of the gas.
- Isobaric Work W3: The work done is generally less than the isothermal work but can be more than the adiabatic work depending on the specific conditions.
W1>W3>W2
Thus, the correct order of work done in the three processes is:
W1>W3>W2
Topper's Solved these Questions
Similar Questions
A gas is expanded to double its volume by two different processes. One is isobaric and the other is isothermal. Let W1 and W2 be the respective work done, then find W1 and W2
View SolutionA gas is expanded from volume V1 to V2 through three different process:
a. Reversible adiabatic
b. Reversible isothermal
c. Irreversible adiabatic (against a constant external pressure Pex)
The correct option is
View Solution
Knowledge Check
Two moles of a gas is expanded to double its volwne by two different processes. One is isobaric and the other is isothermal. If W1 and W2are the works done respectively, then
Aw2=W1ln2BW2=W1CW2=W1ln2DW21=W2ln2A gas expands from i to f along the three paths indicated. The work done along the three paths denoted by W1,W2andW3 have the relationship
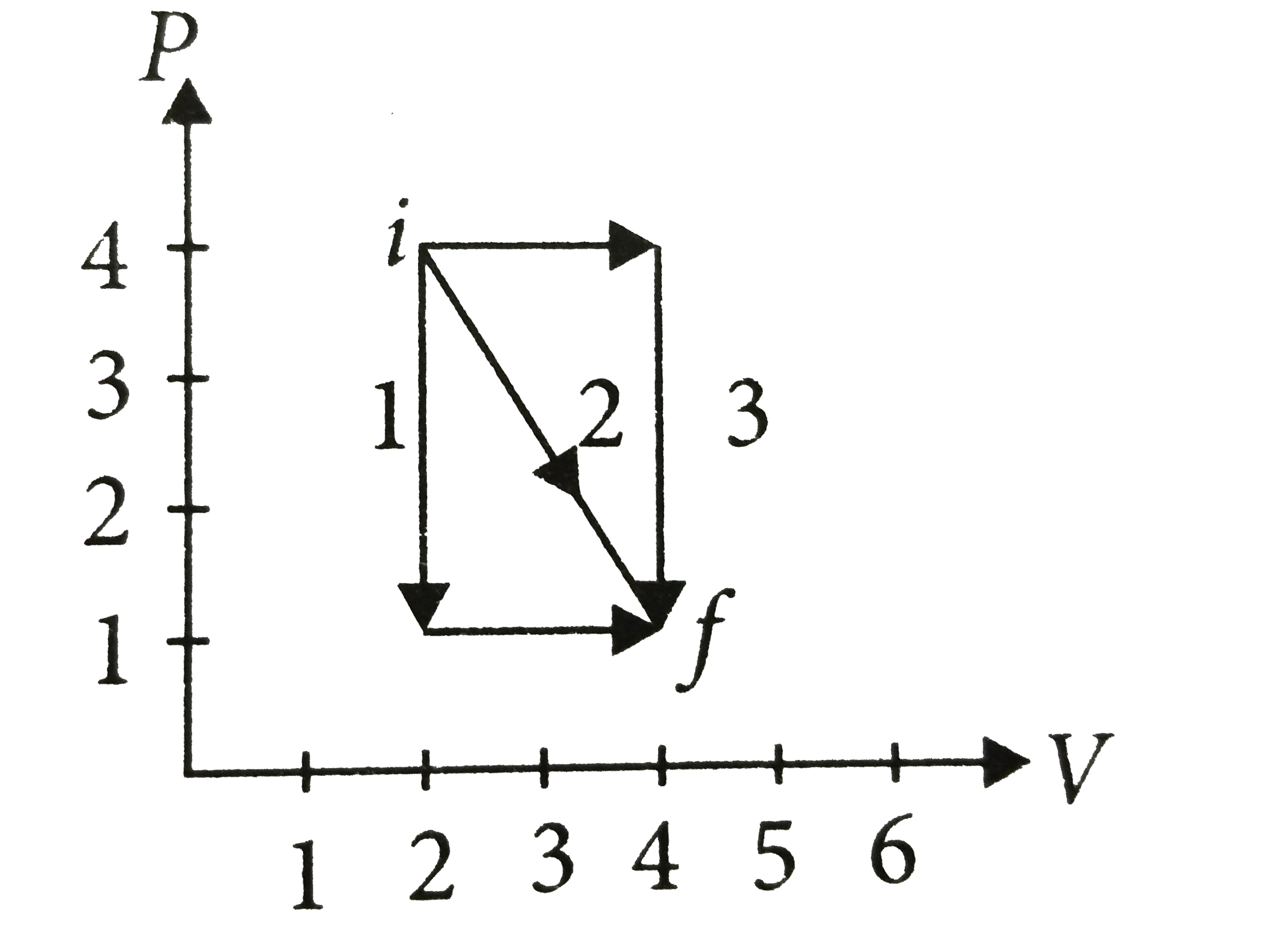 AW1<W2<W3BW2<W1=W3CW2<W1<W3DW1>W2>W3
AW1<W2<W3BW2<W1=W3CW2<W1<W3DW1>W2>W3An ideal gas is initially at P1,V1 is expands to P2,V2 and then compressed adiabatically to the same volume V1 and pressure P3. If W is the net work done by the gas in complete process which of the following is true.
AW>0,P3>P1BW<0,P3>P1CW>0,P3<P1DW<0,P3<P1
An ideal monatomic gas expands to twice its volume. If the process is isothermal, the magnitude of work done by the gas is W1. If the process is adiabatic, the magnitude of work done by the gas is Wa . Which of the following is true ?
View SolutionIf w1,w2,w3 and w4 for an ideal gas are magnitude of work done in isothermal, adiabatic, isobaric and isochoric reversible expansion processes, the correct order will be :
View SolutionAn ideal gas expands from V to 2V in 4 separate processes. Where process 1 is isothermal process 2 is adiabatic, process 3 is free expansion and process 4 is isobaric. If E1,E2,E3 and E4 are the changes in average kinetic energy of the molecules in the isothermal, adiabatic free expansion and isobaric process respectively then choose the correct options.
View SolutionIf during an isothermal process certain gas is expanded from V1 to V2 . Then, which of the following would be true?
View SolutionThe given diagram shows four processes i.e., isochoric, isobaric, isothermal and adiabatic. The correct assignment of the processes, in the same order is given by:
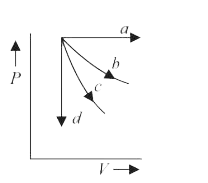
View Solution
