A series LCR circuit is connected to an AC source of 220V,50Hz.The circuit contains a resistance R= 80Ω,an inductor of inductive reactance XL=70Ω,and a capacitor of capacitive reactance XC=130Ω. The power factor of circuit is x10.The value of x is:
To solve the problem, we need to find the power factor of a series LCR circuit connected to an AC source. The given values are:
- Resistance, R=80Ω
- Inductive reactance, XL=70Ω
- Capacitive reactance, XC=130Ω
The power factor (PF) is defined as:
Power Factor=cosϕ=RZ
where Z is the impedance of the circuit, calculated using the formula:
Z=√R2+(XL−XC)2
Step 1: Calculate the reactance difference
First, we need to calculate the difference between the inductive and capacitive reactance:
XL−XC=70Ω−130Ω=−60Ω
Step 2: Calculate the impedance Z
Now we can substitute R and (XL−XC) into the impedance formula:
Z=√R2+(XL−XC)2=√802+(−60)2
Calculating 802 and (−60)2:
802=6400
(−60)2=3600
Now, substituting these values into the impedance formula:
Z=√6400+3600=√10000=100Ω
Step 3: Calculate the power factor
Now we can calculate the power factor:
Power Factor=cosϕ=RZ=80100=0.8
Step 4: Relate the power factor to x
According to the problem, the power factor is given as x10. Therefore, we have:
0.8=x10
Step 5: Solve for x
To find x, we can rearrange the equation:
x=0.8×10=8
Final Answer
Thus, the value of x is:
8
- Inductive reactance, XL=70Ω
- Capacitive reactance, XC=130Ω
(−60)2=3600
Topper's Solved these Questions
Similar Questions
A series RLC circuit , driven with Erms=120 V at frequency fd=60.0 Hz, contains a resistance R=200Ω, and inductance with inductive reactance XL=80.0Ω, and a capacitance with capacitive reactance XC=150Ω.
(a) What are the power factor cos ϕ and phase constant ϕ of the circuit ?View SolutionAn ideal inductor of inductance 50μH is connected to an AC source of 220V, 50 Hz. Find the inductive reactance.
View Solution
Knowledge Check
- Question 1 - Select One
In L-R circuit, resistance is 8Ω and inductive reactance is 6Ω then impedance is:
A2ΩB14ΩC4ΩD10Ω - Question 2 - Select One
In an experiment, 200V AC is applied at the end of an LCR circuit. The circuit consists of an inductive reactance
(XL) =50Ω, capacitive reactance
(XcL=50Ω, and capacitives resistance
(R)=10Ω, The impendance of the circuit isA10ΩB20ΩC30ΩD40Ω - Question 3 - Select One
A series LR circuit is connected to an ac source of frequency ω and the inductive reactance is equal to 2R. A capacitance of capacitive reactance equal to R is added in series with L and R. The ratio of the new power factor to the old one is
A√23B√25C√32D√52
In a seris LCR circuit, the inductive reactance (XL) is 10Ω and the capacitive reactance (XC) is 4Ω. The resistance (R) in the circuit is 6Ω. The power factor of the circuit is :
View SolutionA series RLC circuit is connected to an AC supply 220 V, 50 Hz. The value of R is 10 Ω . The value of capacitive reactance of capacitor and incuctive reactance of inductor are 15 Ω and 20Ω respectively . Calculate the value of current in the circuit.
View SolutionA series LCR circuit is connected top an AC power source of 220 V , 50 Hz. The values of L and R are 0.41 H and 80Ω respectively. What is the value of C if current and voltage are in phase ?
View SolutionThe impedance of a circuit consister of 3Ωresistance and 4Ω reactance. The power factor of the circuit is
View SolutionA series circuit connected across a 200V,60Hz line consists of a capacitive reactance 30Ω non inductive resistor of 44Ω and a coil of inductive reactance 90Ω and resistance 36Ω as shown in the diagram
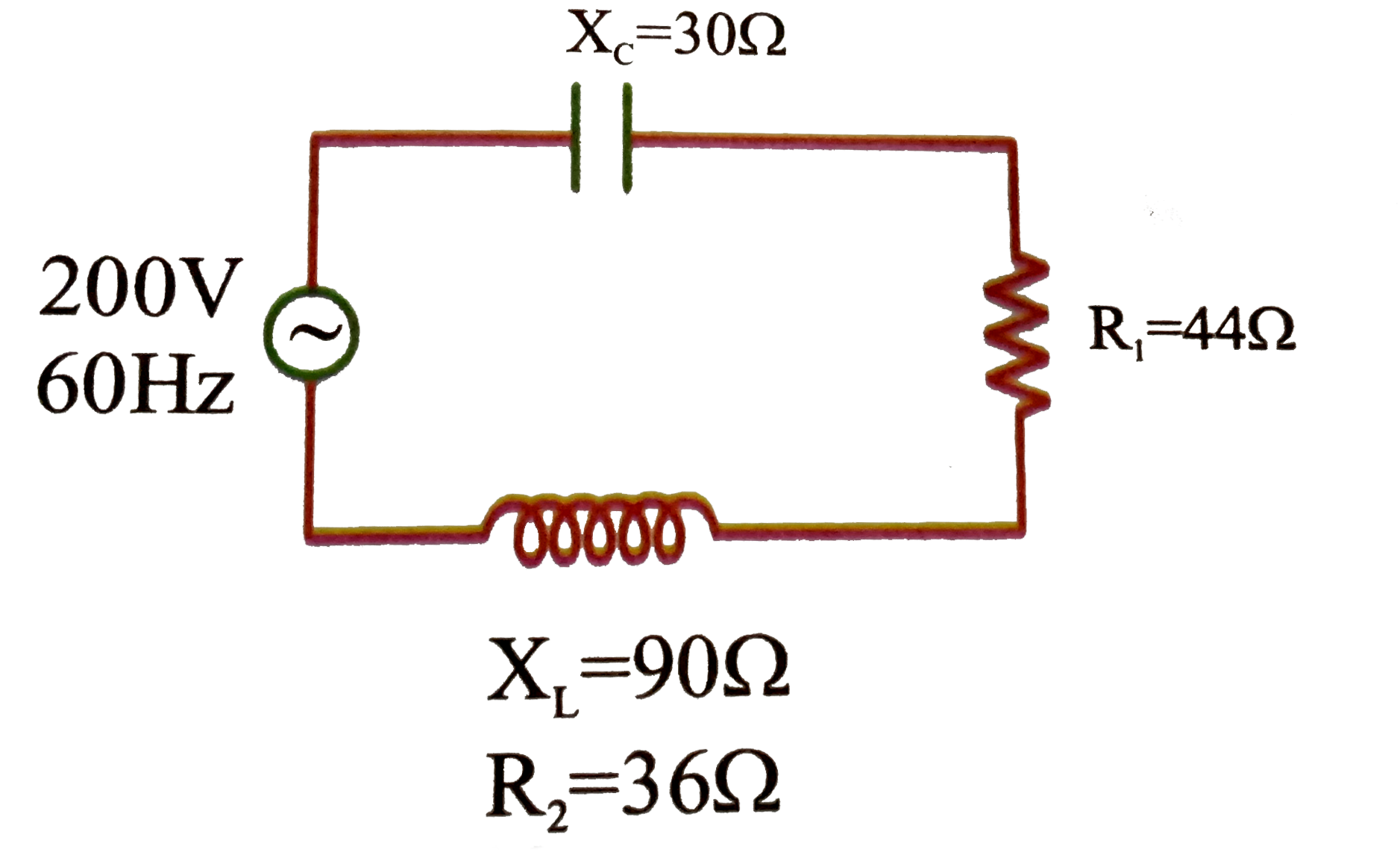
The power used in the circuit isView Solution

