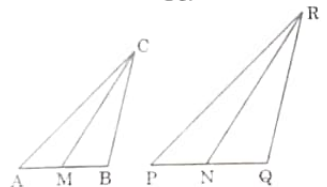
In the given figure, CM and RN are respectively the medians of ΔABC and ΔPQR . If ΔABC~ΔPQR, then prove that ΔAMC~ΔPNR
Step by step video solution for In the given figure, CM and RN are respectively the medians of Delta ABC and DeltaPQR . If DeltaABC ~ Delta PQR, then prove that Delta AMC ~ Delta PNR by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
If ΔABC~ΔPQR and 4A(ΔABC)=25A(ΔPQR) then AB : PQ = ?
A4:25B2:5C5:2D25:4In the given figure, Δ ____ ≅ΔPQR
 AQRDBDRQCRQDDboth (b) and (c)
AQRDBDRQCRQDDboth (b) and (c)
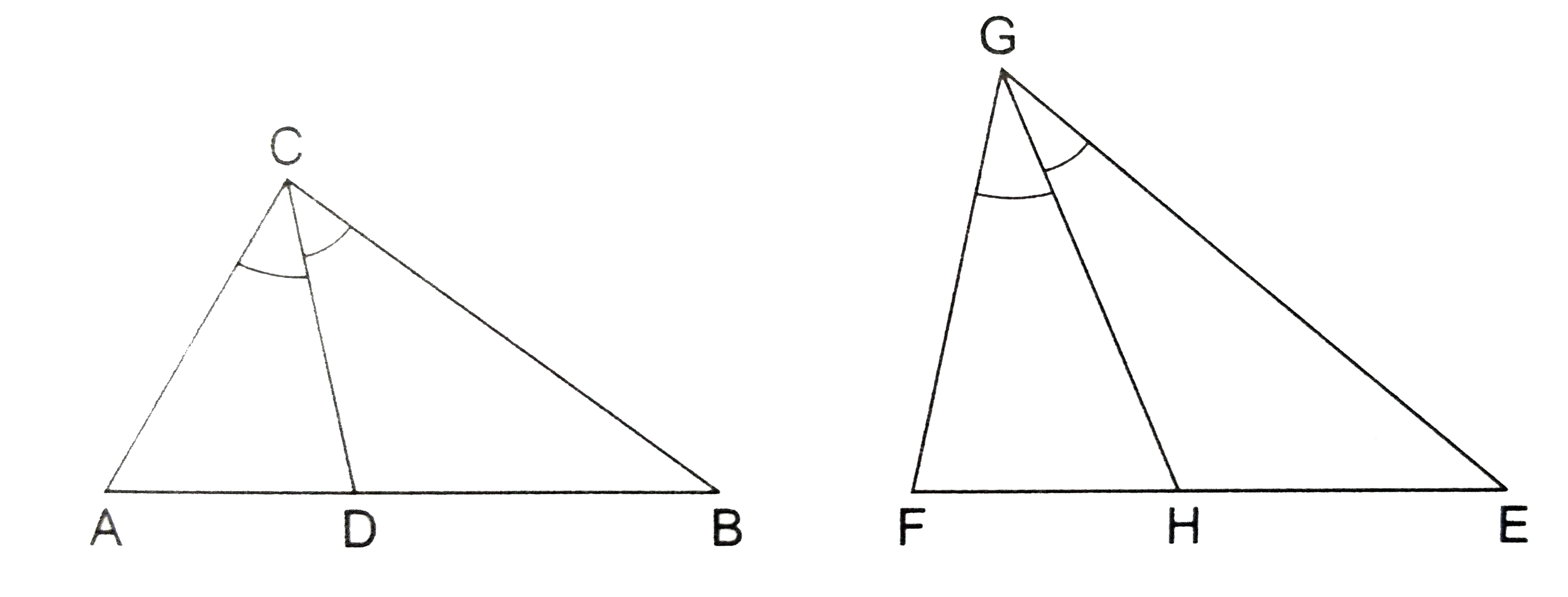
In the given figure, CD and GH are respectively the bisectors of ∠ACBand∠FGEofΔABCandΔEFG respectively . If ΔABC~ΔFEG, prove that :
(a) ΔADC~ΔFHG (b)ΔBCD~ΔEGH (c)CDGH=ACFG
View SolutionIn the given figure, ΔPQR≅Δ ____

View SolutionIn the given figure, ΔPQR≅Δ ____
View SolutionAD and PM are medians of triangles ABC and PQR respectively where ΔABC~ΔPQR. Prove that: ABPQ=ADPM.
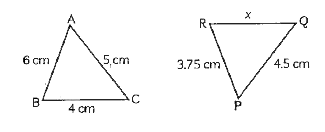
View SolutionIn the given figure ΔABC−ΔPQR. Find the value of x.
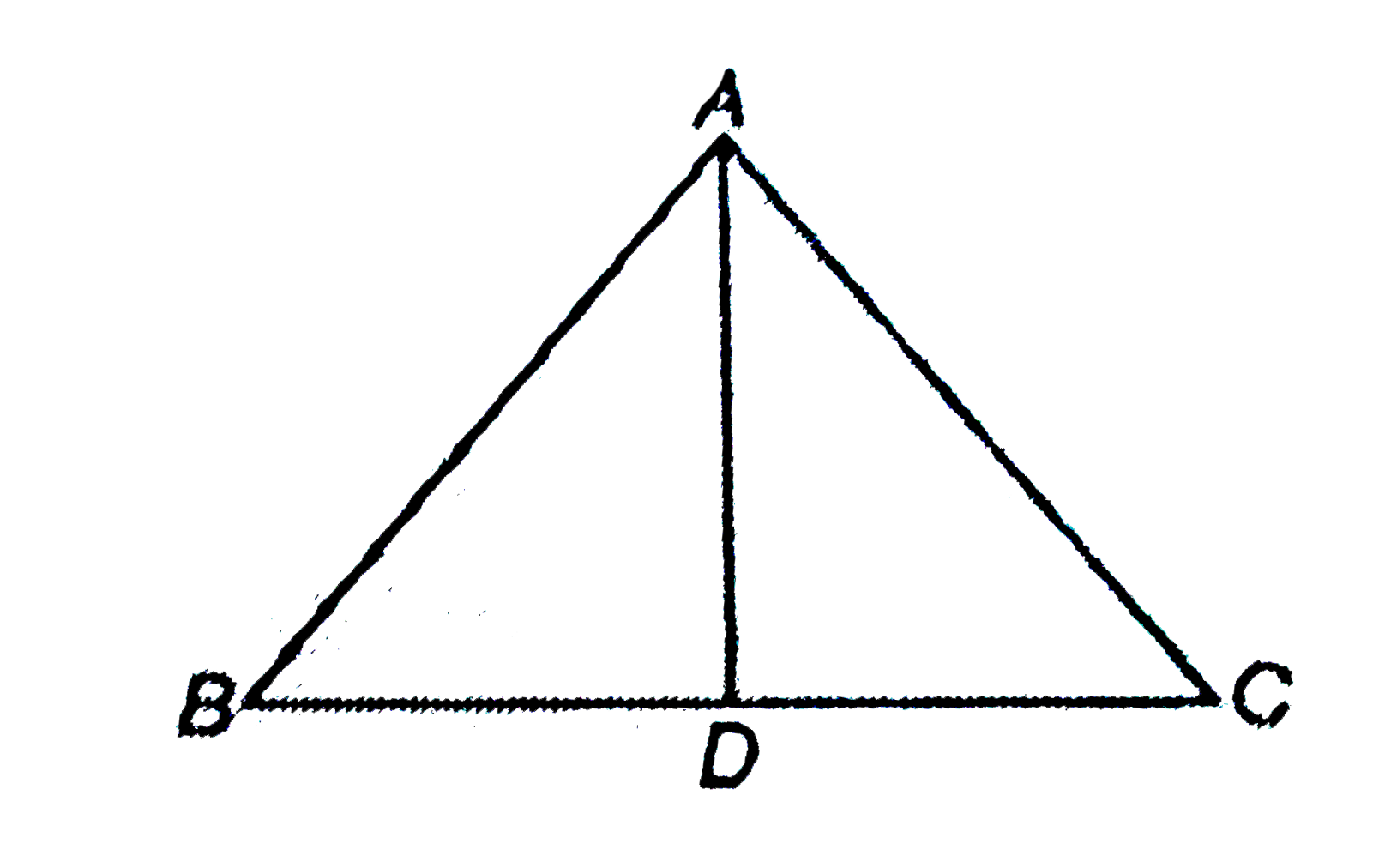
View SolutionIn the given figure AD is the median. If the area of ΔABD=10cm2, find the area of ΔABC
View Solution