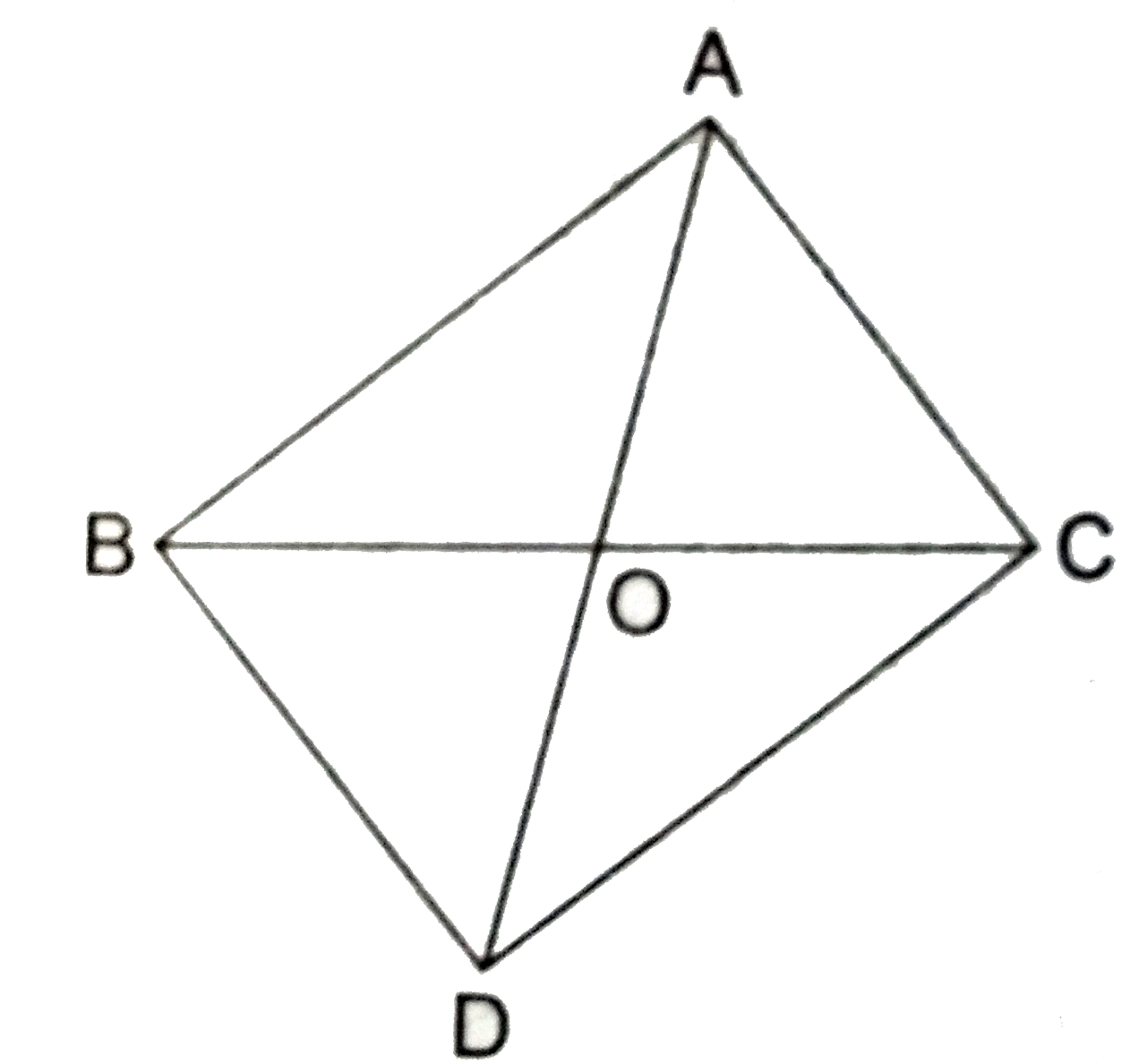
In ΔABC,ADandBE are altitudes. Prove that ar(ΔDEC)ar(ΔABC)=DC2AC2.
Step by step video & image solution for In DeltaABC, AD and BE are altitudes. Prove that (ar(DeltaDEC))/(ar(DeltaABC))= (DC^2)/(AC^2). by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
In ΔABC,AD is the bisector of ∠A . Then , ar(ΔABD)ar(ΔACD)=
AAB2AC2BABACCBMCMDNone of theseIf ΔABC~ΔPQR with BCQR=13,then ar(ΔPRQ)ar(ΔBCA) is equal to
A91B31C13D19
In (ΔABC) , MN∣∣BC and AM:AB=13. Then find the ratio of ar(ΔAMN)ar(ΔABC)
View SolutionIn the ΔABC , MN||BC and AM : : MB = 13.
Then, ar(ΔAMN)ar(ΔABC)=?
View SolutionABCD is a trapezium with AB||DC. A line parallel to AC intersects AB at X and BC at Y. Prove that ar(ΔADX) = ar(ΔACY).
View SolutionIf ΔABC~ΔPQR with BCQR=13,then ar(ΔPRQ)ar(ΔBCA) is equal to
View SolutionA point D is taken on the side BC of a ΔABC such that BD=2DC. Prove that ar(ΔABD)=2ar(ΔADC)
View SolutionIn the figure, ABC and BDE are two equilateral triangle such that D is the mid-point of BC. If AE intersects BC at F, show that:
(i) ar(ΔBDE)=14ar(ΔABC) (ii) ar(ΔBDE)=12ar(ΔBAE)
(iii) ar(ΔABC)=2ar(ΔBEC) (iv) ar(ΔBFE)=ar(ΔAFD)
(v) ar(ΔBFE)=2ar(ΔFED) (vi) ar(ΔFED)=18ar(ΔAFC)
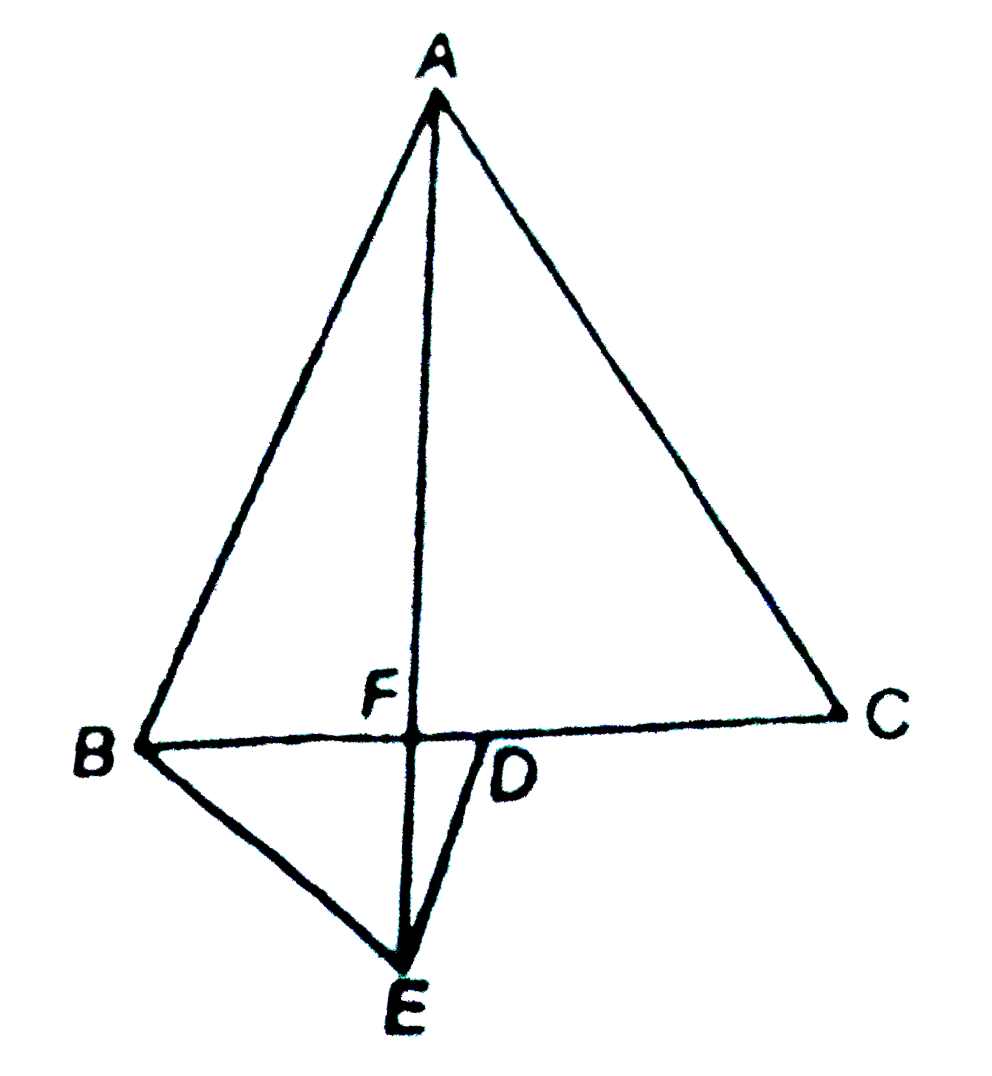
View Solution