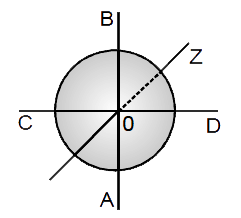
Find the moment of inertia of a uniform rign of mass M and radius R about a diameter.

Video Solution

Text Solution

Verified by Experts

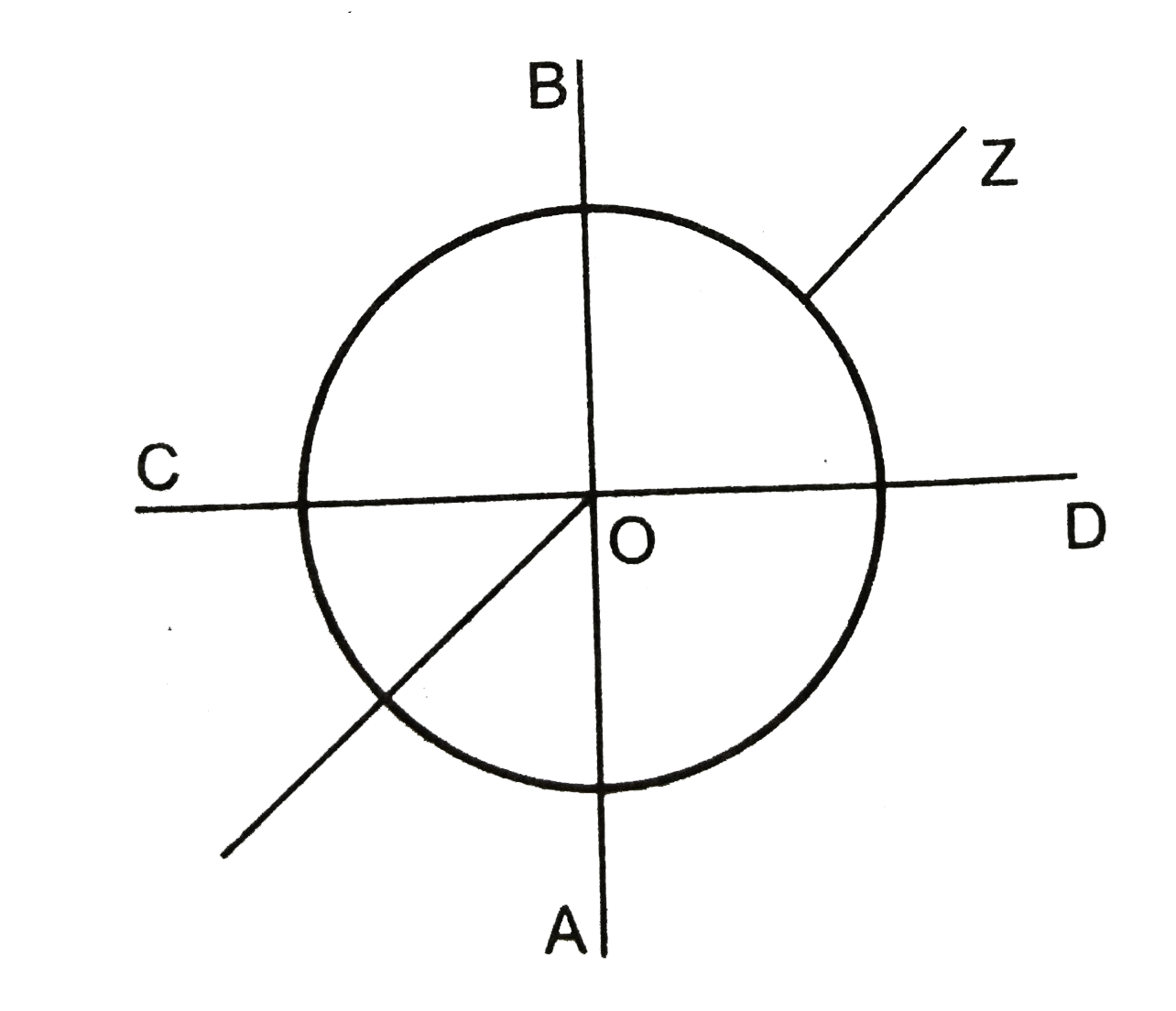
Let AB and CD be two mutually perpendicular diameter of the ring. Take them as M and Y axes and the line perpendicular to the plane of the ring through the centre as the Z-axis. The moment of inertia of the ring about the Z-axis is $I = M R^{2}$ . As the ring is uniform, all of its diameters are equivalent and so $I_{x} = I_{y}$ . From perpendicular axes theorem
$I_{2} = I_{x} + I_{y} H e n c e I_{x} = \frac{I_{z}}{2} = \frac{M R^{2}}{2}$ .
Similarly the moment of inertia of a uniform disc about a diameter is a $M \frac{R^{2}}{4}$

Updated on:21/07/2023

Moment of inertia of a circular wire of mass M and radius R about its diameter is
A $M \frac{R^{2}}{2}$
B $M R^{2}$
C $2 M R^{2}$
D $M \frac{R^{2}}{4}$
Moment of inertia of a disc of mass 'M' and radius 'R' about the diameter is
A $\frac{M R^{2}}{2}$
B $\frac{M R^{2}}{4}$
C $\frac{M R^{2}}{3}$
D $\frac{M R^{2}}{12}$
The moment of inertia of a thin circular disc of mass M and radius R about any diameter is
A $\frac{M R^{2}}{4}$
B $\frac{M R^{2}}{2}$
C $M R^{2}$
D $2 M R^{2}$