The optimal value of the objective function is attained at the points A)given by intersection of inequations with axes only B) given by intersection of inequations with X-axis only C) given by corner points of the feasible region D) none of teh above
given by intersection of inequations with axes only
given by intersection of inequations with X-axis only
given by corner points of the feasible region
None of the above
The correct Answer is:C
The optimal value of the objective function is attained at the points given by corner points of the feasible region.
Topper's Solved these Questions
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - Linear Programming EXERCISE - EXERCISE 1 (TOPICAL PROBLEMS )(Solution of LPP Graphical Method )15videos
Linear Programming
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - Linear Programming EXERCISE - EXERCISE 2 (MISCELLANEOUS PROBLEMS )30videos
Linear Programming
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - LINEEXERCISE - MHT CET Corner3videos
LINE
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - MATHEMATICAL LOGIC EXERCISE - MHT CET CORNER22videos
MATHEMATICAL LOGIC
Similar Questions
The optimal value of the objective function is attained at the points A)Given by intersection of inequations with the axes only B)Given by intersection of inequations with the axes only C)Given by corner points of the feasible region D)None of these
View SolutionThe maximum value of objective function z=2x+3y in the given feasible region is
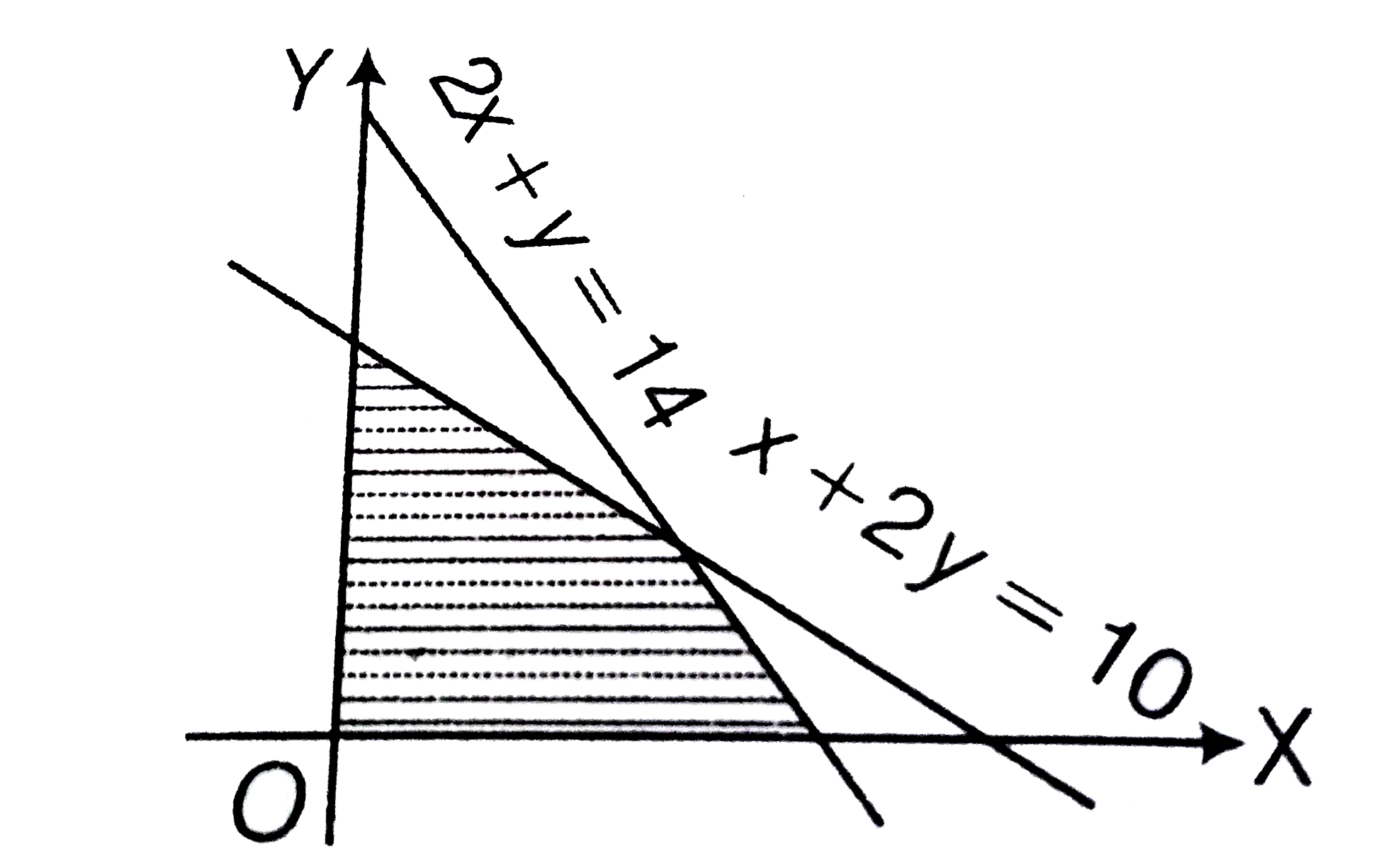 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Consider the following statements
I. If the feasible region of an LPP is undbounded then maximum or minimum value of the obJective function Z = ax + by may or may not exist .
II. Maximum value of the objective function Z = ax + by in an LPP always occurs at only one corner point of the feasible region.
Ill. In an LPP, the minimum value of the objective function Z = ax + by is always 0, if origin is one of the corner point of the feasible region.
IV. In an LPP, the maximum value of the objective function Z = ax + by is always finite.
Which of the following statements are true?AI and IVBII and IIICI and IIIDII and IV - Question 2 - Select One
At the point of intersection of the two curves shown, the conc. of B is given by ……… for, A→nB:
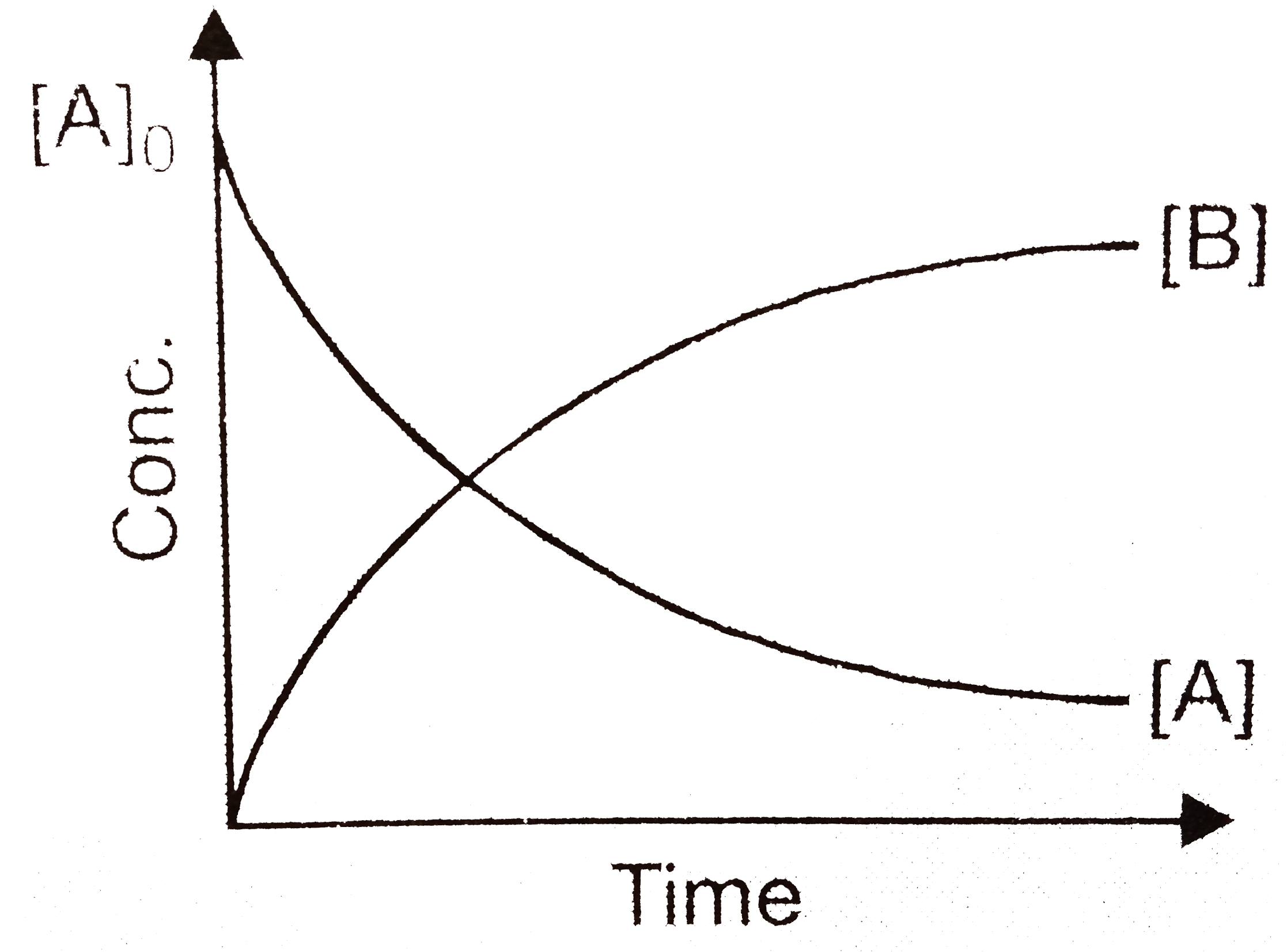 AnA02BA0n−1CnA0n+1D(n−1n+1)A0
AnA02BA0n−1CnA0n+1D(n−1n+1)A0 - Question 3 - Select One
The maximum or minimum of the objective funtion occurs only at the corner points of the feasible region. This theorem is known as fundamental theorem of
AAlgebraBArithmeticCCalculusDExtreme point
The maximum value of objective function Z=3x+y under given feasible region is
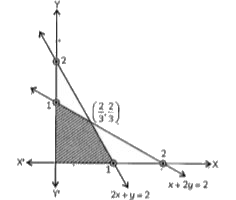 View Solution
View SolutionMaximum value of the objective function Z = ax +by in a LPP always occurs at only one corner point of the feasible region.
View SolutionIn a LPP, the maximum value of the objective function Z = ax +by is always 0, if origin is one of the corner point of the feasible region.
View SolutionIn the given figure, name:

lines whose point of intersection is CView SolutionAt the point of intersection of the two curves shown, the conc.of B is given by….. ….. ……….for, A→nB
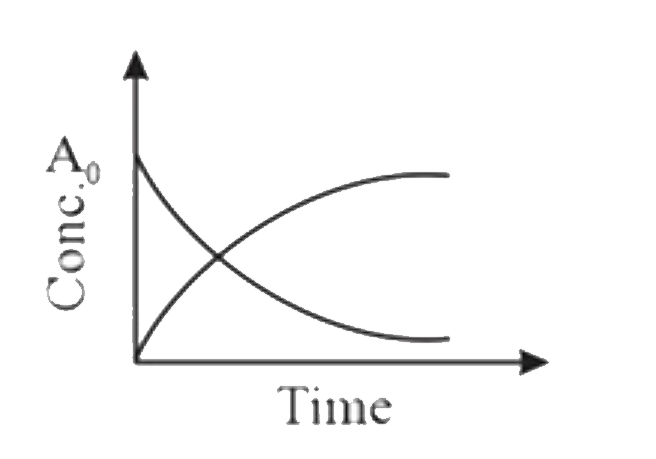 View Solution
View Solution

