If z0,z1 represent points P and Q on the circle |z−1|=1 taken in anticlockwise sense such that the line segment PQ subtends a right angle at the center of the circle, then z1=
A
1+i(z0−1)
B
iz0
C
1−i(z0−1)
D
i(z0−1)
Video Solution
Text Solution
The correct Answer is:A
We have,
∠PCQ=π2,
⇒ CQ is obtained by rotating CP through π/2 in anticlockwise sense.
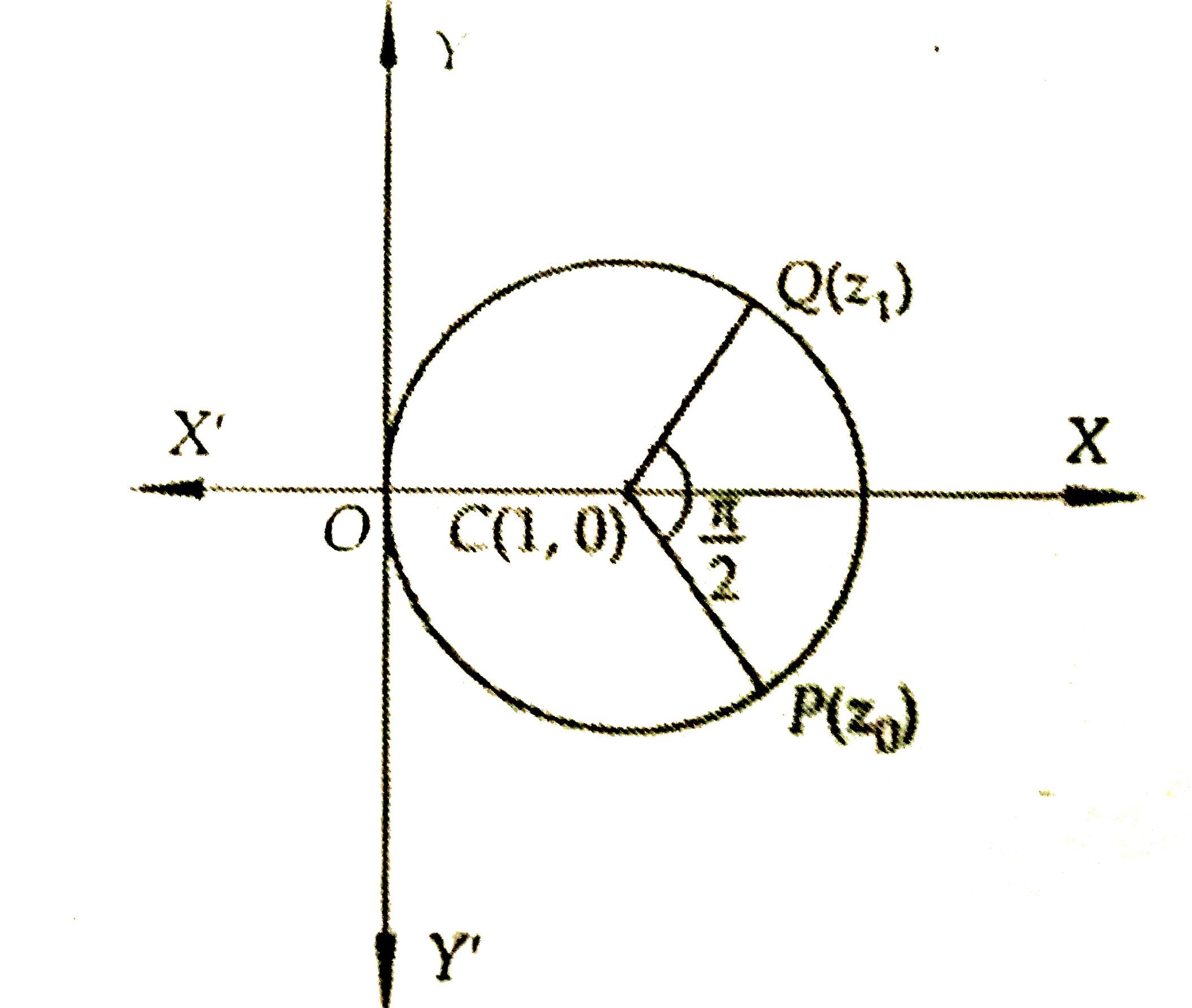
⇒z1−1=|z1−1||z0−1|(z0−1)eiπ/2
rArz1−1=(z0−1)i [∴|z1−1|=|z0−1| = radius]
⇒z1=1+i(z0−1)
|
Topper's Solved these Questions
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Section II - Assertion Reason Type
15 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Exercise
131 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Chapter Test
59 Videos
- CIRCLESBOOK - OBJECTIVE RD SHARMACHAPTER - CIRCLESEXERCISE - Chapter Test
55 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - OBJECTIVE RD SHARMACHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise
86 Videos
Similar Questions
Knowledge Check
If z is a point on the circle |z−1|=1, then arg z =
Aarg|z−1|B12arg(z−1)Carg(z2−z)D13arg(z2−z)If z lies on the circle |z−1|=1, then z−2z is
Apurely realBPurely imaginaryCpositive realDhyperbolaIf z1,z2,z3 be vertices of an equilateral triangle occurig in the anticlockwise sense, then
Az21+z22+z23=2(z1z2+z2z3+z3z1)B1z1+z2+1z2+z3+1z3+z1=0Cz1+ωz2+ω2z3=0Dnone of these
If z lies on the circle I z l = 1, then 2/z lies on
View SolutionIf the circle |z−1|=3and|z−z0|=4 intersect orthogonally, then
View SolutionLet z1,z2 be two complex numbers represented by points on the circle |z1|=andand|z2|=2 are then
View SolutionQ. Let z1 and z2 be nth roots of unity which subtend a right angle at the origin, then n must be the form 4k.
View SolutionLet z1,z2 be two complex numbers represented by points on the circle |z1|=1and|z2|=2 respectively then
View Solution
