If , lies in on , then :
A
B
C
D
Video Solution
Text Solution
The correct Answer is:D
We observe that represent the set of all interior and boundary points of the circle having center at and radius 4.
The equation represents the set of all points lying on the boundary of the ellipse having its two foci at and and major axis of length 3.
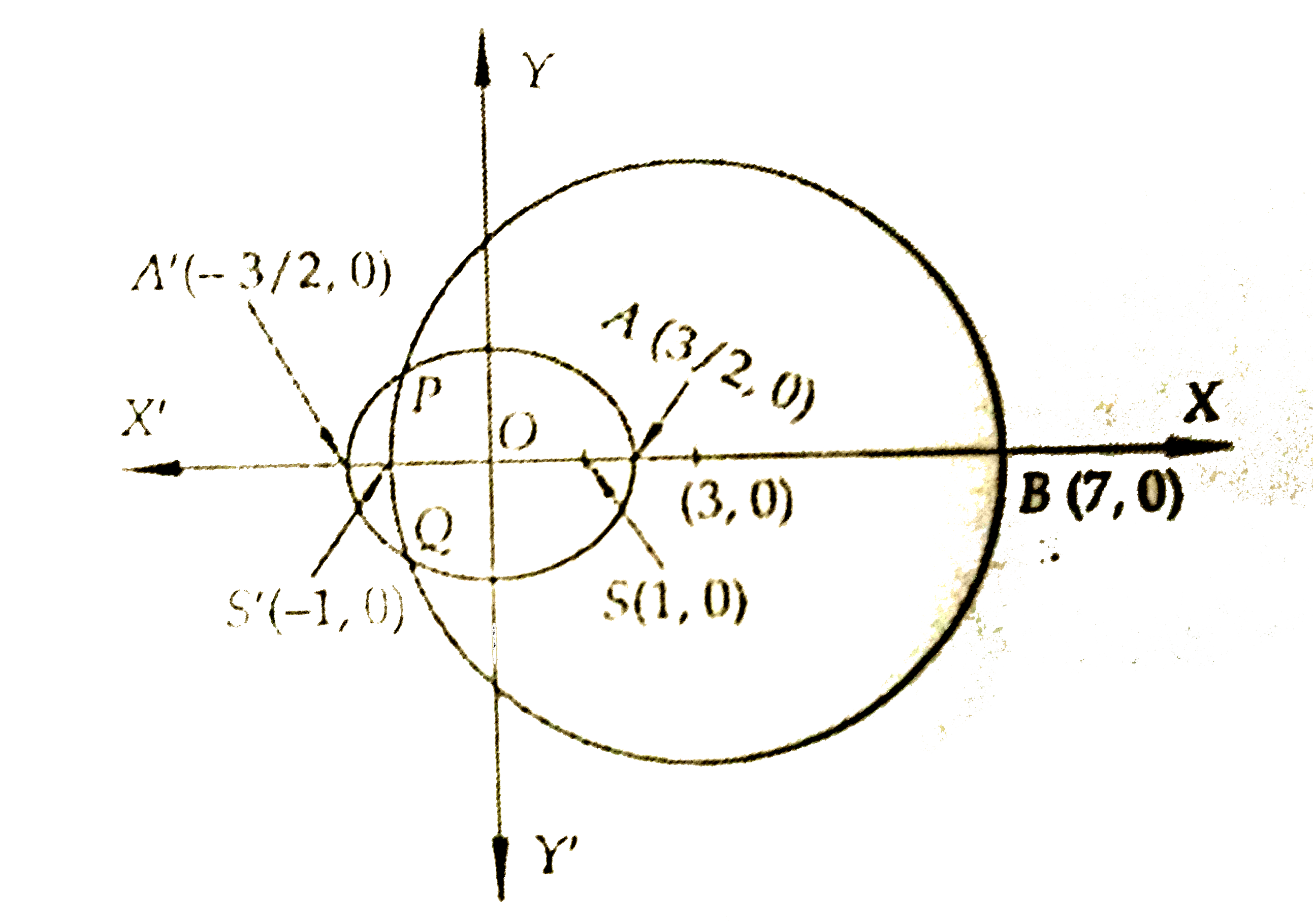
Clearly, when (=affix of P or Q)
Also, is greatest when = affix of B and = affix of a.
max.
Hence, .
|
Topper's Solved these Questions
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Section II - Assertion Reason Type
15 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Exercise
131 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Chapter Test
59 Videos
- CIRCLESBOOK - OBJECTIVE RD SHARMACHAPTER - CIRCLESEXERCISE - Chapter Test
55 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - OBJECTIVE RD SHARMACHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise
86 Videos
Similar Questions
Knowledge Check
If z1 lies on |z| = r, then equation of tangent at z1 is
Az¯z1+¯z¯z1=2Bz¯z1+¯z¯z1=rCz¯z1+¯zz1=2Dz¯z1+¯zz1=rif A(z1),B(z2),C(z3),D(z4) lies on |z|=4 (taken in order) , where z1+z2+z3+z4=0 then :
AMax. area of quadrilateral ABCD=32BMax. area of quadrilateral ABCD=16CThe triangle ΔABC is right angledDThe quadrilateral ABCD is rectangleIf z=x+iy lies in the third quadrant, then ¯zz also lies in the third quadrant, if
Ax>y>0Bx<y<0Cy<x<0Dy>x>0
If z1 and z2 lies on |z|=9 and |z−3−4i|=4 respectively, find minimum possible value of |z1−z2| (A) 0 (B) 5 (C) 13 (D) 2
View SolutionIf z1,z2,z3 are non zero non collinear complex number such that 2z1=1z2+1z3,then (A) ponts z1,z2,z3 form and equilateral triangle (B) points z1,z2,z3lies on a circle (C) z1,z2,z3 and origin are concylic (D) z1+z2+z3=0
View SolutionIf z lies on the curve |z−3−4i|=3,then least value of |z| is
View SolutionIf z = x + iy lies in III quadrant, then ¯zz also lies in III quadrant If:
View SolutionIf z lies on the circle I z l = 1, then 2/z lies on
View Solution
